
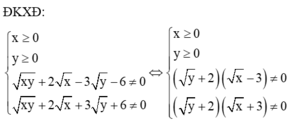
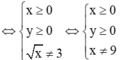
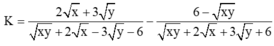
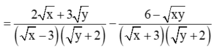
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

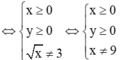
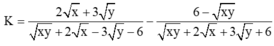
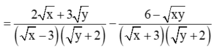
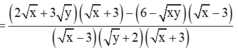
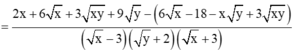
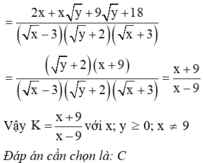

a/ \(P=\frac{1}{\sqrt{xy}}\)
b/ \(x^3=8-6x\)
\(\Rightarrow P=\frac{1}{\sqrt{x\left(x^2+6\right)}}=\frac{1}{\sqrt{x^3+6x}}=\frac{1}{\sqrt{8-6x+6x}}=\frac{1}{2\sqrt{2}}\)

a: \(=\dfrac{3}{2}\sqrt{6}+\dfrac{2}{3}\sqrt{6}-2\sqrt{6}\)
\(=\dfrac{1}{6}\sqrt{6}\)
b: \(VT=\dfrac{\sqrt{y}}{\sqrt{x}\left(\sqrt{x}-\sqrt{y}\right)}+\dfrac{\sqrt{x}}{\sqrt{y}\left(\sqrt{y}-\sqrt{x}\right)}\)
\(=\dfrac{y-x}{\sqrt{xy}\left(\sqrt{x}-\sqrt{y}\right)}=\dfrac{-\left(\sqrt{x}+\sqrt{y}\right)}{\sqrt{xy}}\)

a: \(=\dfrac{3}{2}\sqrt{6}+\dfrac{2}{3}\sqrt{6}-2\sqrt{3}=\dfrac{13}{6}\sqrt{6}-2\sqrt{3}\)
b: \(VT=\dfrac{\sqrt{xy}\left(\sqrt{x}+\sqrt{y}\right)}{\sqrt{xy}}\cdot\left(\sqrt{x}+\sqrt{y}\right)=\left(\sqrt{x}+\sqrt{y}\right)^2\)
c: \(VT=\dfrac{\sqrt{y}}{\sqrt{x}\left(\sqrt{x}-\sqrt{y}\right)}+\dfrac{\sqrt{x}}{\sqrt{y}\left(\sqrt{y}-\sqrt{x}\right)}\)
\(=\dfrac{y-x}{\sqrt{xy}\left(\sqrt{x}-\sqrt{y}\right)}=\dfrac{-\left(\sqrt{x}+\sqrt{y}\right)}{\sqrt{xy}}\)

\(A=b^5\sqrt{\dfrac{a^2+6a+9}{b^8}}\)
\(A=b^5\dfrac{\sqrt{\left(a+3\right)^2}}{\sqrt{b^8}}\)
\(A=b^5\dfrac{|a+3|}{b^4}=b|a+3|\)
\(B=\dfrac{x+y+2\sqrt{xy}}{x\sqrt{x}-y\sqrt{y}+x\sqrt{y}-y\sqrt{x}}\)
\(B=\dfrac{\left(\sqrt{x}+\sqrt{y}\right)^2}{\left(x-y\right)\left(\sqrt{x}+\sqrt{y}\right)}=\dfrac{\sqrt{x}+\sqrt{y}}{x-y}\)
\(B=\dfrac{\sqrt{x}+\sqrt{y}}{\left(\sqrt{x}-\sqrt{y}\right)\left(\sqrt{x}+\sqrt{y}\right)}=\dfrac{1}{\sqrt{x}-\sqrt{y}}\)

a: \(=6\cdot\sqrt{\dfrac{2xy}{4y^2}}\)
\(=6\cdot\dfrac{\sqrt{2xy}}{-2y}=-\dfrac{3\sqrt{2xy}}{y}\)
b: \(=\dfrac{4xy^2}{3}\cdot\dfrac{3}{\sqrt{xy}}=4\sqrt{x}\cdot y\sqrt{y}\)