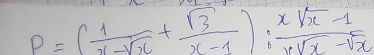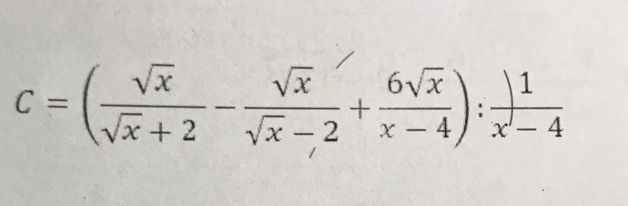K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
ND
1
NV
Nguyễn Việt Lâm
Giáo viên
19 tháng 3 2023
\(f\left(1-3x\right)=2\left(1-3x\right)-\left(1-3x\right)^2=1-9x^2\)
K
2
HT
1 tháng 9 2020
đk: \(x\ge0\)
Ta có: \(\sqrt{x}+2\sqrt{x+3}=x+4\)
\(\Leftrightarrow\left(x+3\right)-2\sqrt{x+3}+1=\sqrt{x}-1\)
\(\Leftrightarrow\sqrt{\left(\sqrt{x-3}-1\right)^2}=\sqrt{x}-1\)
\(\Leftrightarrow\left|\sqrt{x-3}-1\right|=\sqrt{x}-1\)
\(\Leftrightarrow\orbr{\begin{cases}\sqrt{x-3}-1=\sqrt{x}-1\\\sqrt{x-3}-1=1-\sqrt{x}\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}\sqrt{x-3}=\sqrt{x}\left(ktm\right)\\\sqrt{x-3}+\sqrt{x}=2\end{cases}}\)
\(\Leftrightarrow x-3+x+2\sqrt{x\left(x-3\right)}=4\)
\(\Leftrightarrow2\sqrt{x^2-3x}=7-2x\)
\(\Leftrightarrow4\left(x^2-3x\right)=\left(7-2x\right)^2\)
\(\Leftrightarrow4x^2-12x=49-28x+4x^2\)
\(\Leftrightarrow16x=49\)
\(\Rightarrow x=\frac{49}{16}\)
16 tháng 12 2021
\(C=\dfrac{\sqrt{x}\left(\sqrt{x}-2\right)-\sqrt{x}\left(\sqrt{x}+2\right)+6\sqrt{x}}{x-4}.\left(x-4\right)=2\sqrt{x}\)
T
1
AH
Akai Haruma
Giáo viên
15 tháng 7 2023
Lời giải:
$\frac{x-2y}{3z}$ có thể nhận giá trị lớn nhất nếu $x$ lớn nhất và $y,z$ nhỏ nhất có thể.
$x$ lớn nhất có thể nhận là $14$ (theo điều kiện)
$y,z$ nhỏ nhất có thể nhận là $1,2$ (do $y,z$ phân biệt)
Nếu $x=14, y=1,z=2$ thì $\frac{x-2y}{3z}=2$
Nếu $x=14; y=2, z=1$ thì $\frac{x-2y}{3z}=\frac{10}{3}>2$
Đáp án D.