Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

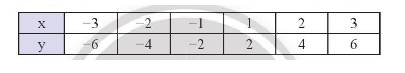
a) \(f\left( 1 \right) = 3.1 = 3;f\left( { - 2} \right) = 3.\left( { - 2} \right) = - 6;f\left( {\dfrac{1}{3}} \right) = 3.\dfrac{1}{3} = 1\).
b) Ta có: \(f\left( { - 3} \right) = 3.\left( { - 3} \right) = - 9;f\left( { - 1} \right) = 3.\left( { - 1} \right) = - 3\)
\(f\left( 0 \right) = 3.0 = 0;f\left( 2 \right) = 3.2 = 6;f\left( 3 \right) = 3.3 = 9\);
Ta lập được bảng sau
\(x\) | –3 | –2 | –1 | 0 | 1 | 2 | 3 |
\(y\) | –9 | -6 | –3 | 0 | 3 | 6 | 9 |

a) Đại lượng \(y\) là hàm số của đại lượng \(x\) vì với mỗi giá trị của \(x\) ta chỉ xác nhận được duy nhất một giá trị \(y\) tương ứng.
b) \(f\left( 2 \right) = {2^2} = 4;f\left( { - 3} \right) = {\left( { - 3} \right)^2} = 9\)
Ta có: \(f\left( { - 2} \right) = {\left( { - 2} \right)^2} = 4;f\left( { - 1} \right) = {\left( { - 1} \right)^2} = 1\)
\(f\left( 0 \right) = {0^2} = 0;f\left( 1 \right) = {1^2} = 1\)
\(f\left( 2 \right) = {2^2} = 4;f\left( 3 \right) = {3^2} = 9\)
\(x\) | –3 | –2 | –1 | 0 | 1 | 2 | 3 |
\(f\left( x \right)\) | 9 | 4 | 1 | 0 | 1 | 4 | 9 |

a) Để hàm số \(y = \left( {m - 1} \right)x + m\) là hàm số bậc nhất thì \(m - 1 \ne 0 \Leftrightarrow m \ne 1\).
Vậy để hàm số \(y = \left( {m - 1} \right)x + m\) là hàm số bậc nhất thì \(m \ne 1\).
b) Ta có: \(y = 3 - 2mx = - 2mx + 3\)
Để hàm số \(y = - 2mx + 3\) là hàm số bậc nhất thì \( - 2m \ne 0 \Leftrightarrow m \ne 0\).
Vậy để hàm số \(y = 3 - 2mx\) là hàm số bậc nhất thì \(m \ne 0\).
a) \(y=\left(m-1\right)x+1\) là hàm số bậc nhất khi và chỉ khi
\(m-1\ne0\Leftrightarrow m\ne1\)
b) \(y=3-2mx\) là hàm số bậc nhất khi và chỉ khi
\(-2m\ne0\Leftrightarrow m\ne0\)

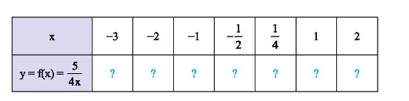
a) Ta có:
\(f\left( {\dfrac{1}{5}} \right) = \dfrac{5}{{4.\dfrac{1}{5}}} = \dfrac{5}{{\dfrac{4}{5}}} = 5:\dfrac{4}{5} = 5.\dfrac{5}{4} = \dfrac{{25}}{4};\)
\(f\left( { - 5} \right) = \dfrac{5}{{4.\left( { - 5} \right)}} = \dfrac{5}{{ - 20}} = \dfrac{{ - 1}}{4};\)
\(f\left( {\dfrac{4}{5}} \right) = \dfrac{5}{{4.\dfrac{4}{5}}} = \dfrac{5}{{\dfrac{{16}}{5}}} = 5:\dfrac{{16}}{5} = 5.\dfrac{5}{{16}} = \dfrac{{25}}{{16}}\)
b) Ta có:
\(f\left( { - 3} \right) = \dfrac{5}{{4.\left( { - 3} \right)}} = \dfrac{5}{{ - 12}} = \dfrac{{ - 5}}{{12}};\)
\(f\left( { - 2} \right) = \dfrac{5}{{4.\left( { - 2} \right)}} = \dfrac{5}{{ - 8}} = \dfrac{{ - 5}}{8};\)
\(f\left( { - 1} \right) = \dfrac{5}{{4.\left( { - 1} \right)}} = \dfrac{5}{{ - 4}} = \dfrac{{ - 5}}{4};\)
\(f\left( { - \dfrac{1}{2}} \right) = \dfrac{5}{{4.\left( { - \dfrac{1}{2}} \right)}} = \dfrac{5}{{\dfrac{{ - 4}}{2}}} = \dfrac{5}{{ - 2}} = \dfrac{{ - 5}}{2}\);
\(f\left( {\dfrac{1}{4}} \right) = \dfrac{5}{{4.\dfrac{1}{4}}} = \dfrac{5}{{\dfrac{4}{4}}} = \dfrac{5}{1} = 5\);
\(f\left( 1 \right) = \dfrac{5}{{4.1}} = \dfrac{5}{4}\);
\(f\left( 2 \right) = \dfrac{5}{{4.2}} = \dfrac{5}{8}\)
Ta có bảng sau:
\(x\) | –3 | –2 | –1 | \( - \dfrac{1}{2}\) | \(\dfrac{1}{4}\) | 1 | 2 |
\(y = f\left( x \right) = \dfrac{5}{{4x}}\) | \(\dfrac{{ - 5}}{{12}}\) | \(\dfrac{{ - 5}}{8}\) | \(\dfrac{{ - 5}}{4}\) | \(\dfrac{{ - 5}}{2}\) | 5 | \(\dfrac{5}{4}\) | \(\dfrac{5}{8}\) |

a, A = (x-1)(x+6) (x+2)(x+3)
= (x^2 + 5x -6 ) (x^2 + 5x + 6)
Đặt t = x^2 +5x
A= (t-6)(t+6)
= t^2 - 36
GTNN của A là -36 khi và ck t= 0
<=> x^2 +5x = 0
<=> x=0 hoặc x=-5
Vậy...

5.\(C\text{ó}x^2-12=0\Rightarrow x^2=12\Rightarrow x=\sqrt{12}ho\text{ặc}x=-\sqrt{12}\)
Mà x>0\(\Rightarrow x=\sqrt{12}\)
6.Vì x-y=4\(\Rightarrow\left(x-y\right)^2=x^2-2xy+y^2=x^2-10+y^2=4^2=16\Rightarrow x^2+y^2=26\)
Có \(\left(x+y\right)^2=x^2+2xy+y^2=26+10=36=6^2=\left(-6\right)^2\)
Vì xy>0 và x>0 =>y>0=>x+y>0=>x+y=6
7. \(3x^2+7=\left(x+2\right)\left(3x+1\right)\)
\(3x^2+7=3x^2+7x+2\)
\(3x^2+7-3x^2-7x-2=0\)
-7x+5=0
-7x=-5
\(x=\frac{5}{7}\)
8.\(\left(2x+1\right)^2-4\left(x+2\right)^2=9\)
\(\left(2x+1\right)^2-\left(2x+4\right)^2=9\)
(2x+1-2x-4)(2x+1+2x+4)=9
-3(4x+5)=9
4x+5=-3
4x=-8
x=-2
Còn câu 9 và 10 để mình nghiên cứu đã

a)
\(\begin{array}{l}A = 0,2\left( {5{\rm{x}} - 1} \right) - \dfrac{1}{2}\left( {\dfrac{2}{3}x + 4} \right) + \dfrac{2}{3}\left( {3 - x} \right)\\A = x - 0,2 - \dfrac{1}{3}x - 2 + 2 - \dfrac{2}{3}x\\ = \left( {x - \dfrac{1}{3}x - \dfrac{2}{3}x} \right) + \left( {\dfrac{{ - 1}}{2} - 2 + 2} \right)\\ = - \dfrac{1}{2}\end{array}\)
Vậy \(A = - \dfrac{1}{2}\) không phụ thuộc vào biến x
b)
\(\begin{array}{l}B = \left( {x - 2y} \right)\left( {{x^2} + 2{\rm{x}}y + 4{y^2}} \right) - \left( {{x^3} - 8{y^3} + 10} \right)\\B = \left[ {x - {{\left( {2y} \right)}^3}} \right] - {x^3} + 8{y^3} - 10\\B = {x^3} - 8{y^3} - {x^3} + 8{y^3} - 10 = - 10\end{array}\)
Vậy B = -10 không phụ thuộc vào biến x, y.
c)
\(\begin{array}{l}C = 4{\left( {x + 1} \right)^2} + {\left( {2{\rm{x}} - 1} \right)^2} - 8\left( {x - 1} \right)\left( {x + 1} \right) - 4{\rm{x}}\\{\rm{C = 4}}\left( {{x^2} + 2{\rm{x}} + 1} \right) + \left( {4{{\rm{x}}^2} - 4{\rm{x}} + 1} \right) - 8\left( {{x^2} - 1} \right) - 4{\rm{x}}\\C = 4{{\rm{x}}^2} + 8{\rm{x}} + 4 + 4{{\rm{x}}^2} - 4{\rm{x}} + 1 - 8{{\rm{x}}^2} + 8 - 4{\rm{x}}\\C = \left( {4{{\rm{x}}^2} + 4{{\rm{x}}^2} - 8{{\rm{x}}^2}} \right) + \left( {8{\rm{x}} - 4{\rm{x}} - 4{\rm{x}}} \right) + \left( {4 + 1 + 8} \right)\\C = 13\end{array}\)
Vậy C = 13 không phụ thuộc vào biến x

Ta có:
\(\begin{array}{l}f(1) = 3.1 + 2 = 5;\\f(0) = 3.0 + 2 = 2\\f( - 2) = 3.\left( { - 2} \right) + 2 = - 4;\\f\left( {\dfrac{1}{2}} \right) = 3.\dfrac{1}{2} + 2 = \dfrac{7}{2};\\f\left( { - \dfrac{2}{3}} \right) = 3.\left( { - \dfrac{2}{3}} \right) + 2 = 0\end{array}\)

+ Với \(x = - 3\)\( \Rightarrow f\left( { - 3} \right) = 4.\left( { - 3} \right) - 1 = - 13;g\left( { - 3} \right) = - 0,5.\left( { - 3} \right) + 8 = 9,5\);
+ Với \(x = - 2\)\( \Rightarrow f\left( { - 2} \right) = 4.\left( { - 2} \right) - 1 = - 9;g\left( { - 2} \right) = - 0,5.\left( { - 2} \right) + 8 = 9\);
+ Với \(x = - 1\)\( \Rightarrow f\left( { - 1} \right) = 4.\left( { - 1} \right) - 1 = - 5;g\left( { - 1} \right) = - 0,5.\left( { - 1} \right) + 8 = 8,5\);
+ Với \(x = 0\)\( \Rightarrow f\left( 0 \right) = 4.0 - 1 = - 1;g\left( 0 \right) = - 0,5.0 + 8 = 8\);
+ Với \(x = 1\)\( \Rightarrow f\left( 1 \right) = 4.1 - 1 = 3;g\left( 1 \right) = - 0,5.1 + 8 = 7,5\);
+ Với \(x = 2\)\( \Rightarrow f\left( 2 \right) = 4.2 - 1 = 7;g\left( 2 \right) = - 0,5.2 + 8 = 7\);
+ Với \(x = 3\)\( \Rightarrow f\left( 3 \right) = 4.3 - 1 = 11;g\left( 3 \right) = - 0,5.3 + 8 = 6,5\).
Ta có bảng sau:
\(x\)
–3
–2
–1
0
1
2
3
\(y = f\left( x \right) = 4x - 1\)
–13
–9
–5
–1
3
7
11
\(y = g\left( x \right) = - 0,5x + 8\)
9,5
9
8,5
8
7,5
7
6,5