
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


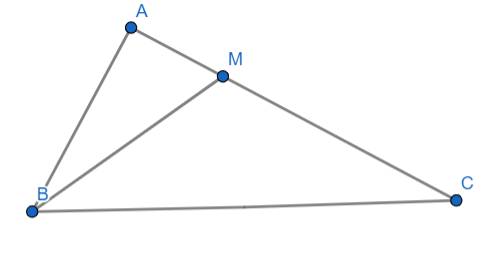
a) Áp dụng định lý Py-ta-go cho tam giác ABC vuông tại A ta có:
\(BC^2=AB^2+AC^2\)
\(\Rightarrow BC=\sqrt{AB^2+AC^2}\)
\(\Rightarrow BC=\sqrt{10^2+20^2}=10\sqrt{5}\left(cm\right)\)
Áp dụng định lý Py-ta-go cho tam giác ABM vuông tại A ta có:
\(BM^2=AB^2+AM^2\)
\(\Rightarrow BM=\sqrt{AB^2+AM^2}\)
\(\Rightarrow BM=\sqrt{10^2+5^2}=5\sqrt{5}\left(cm\right)\)
b) Ta có:
\(\dfrac{AM}{AB}=\dfrac{1}{2}\)
\(\dfrac{BM}{BC}=\dfrac{1}{2}\)
\(\Rightarrow\dfrac{AM}{AB}=\dfrac{MB}{BC}=\dfrac{1}{2}\)
Xét hai tam giác ABC và AMB có:
\(\widehat{BAC}\) chung
\(\dfrac{AM}{AB}=\dfrac{MB}{BC}=\dfrac{1}{2}\)
\(\Rightarrow\Delta ABC\sim\Delta AMB\left(c.g.c\right)\)

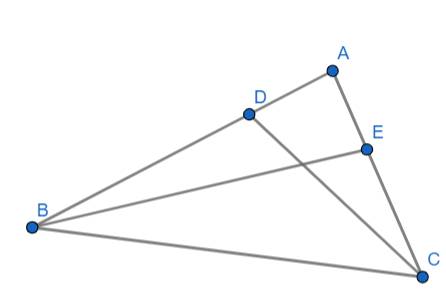
a) Xét hai tam giác ABE và ACD có:
\(\widehat{ACD}=\widehat{ABE}\left(gt\right)\)
\(\widehat{BAC}\) chung
\(\Rightarrow\Delta ABE\sim\Delta ACD\left(g.g\right)\)
b) Ta có: \(\Delta ABE\sim\Delta ACD\left(cmt\right)\)
\(\Rightarrow\dfrac{AB}{AC}=\dfrac{AE}{AD}\)


a) 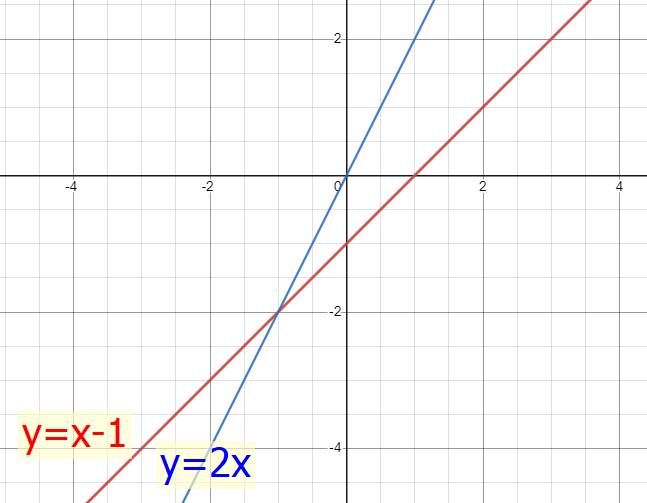
b) Phương trình hoành độ giao điểm của hai đường thẳng là:
\(x-1=2x\)
\(\Leftrightarrow2x-x=-1\)
\(\Leftrightarrow x=-1\)
Thay x = - 1 vào y = 2x ta có: \(y=2\cdot-1=-2\)
Vậy tọa độ giao điểm của 2 đường thẳng là \(\left(-1;-2\right)\)

a) Vào năm 2000 diện tích đất nông nghiệp ở nước ta là:
Thay t = 0 vào \(S=0,12t+8,97\) (vì t được tính theo số năm kể từ năm 2000) ta có:
\(S=0,12\cdot0+8,97=8,97\left(tr.ha\right)\)
b) Diện tích đất nông nghiệp ở nước ra đạt 10,05 triệu hec-ta ta thay \(S=10,05\) ta có:
\(10,05=0,12t+8,97\)
\(\Leftrightarrow0,12t=10,05-8,97\)
\(\Leftrightarrow0,12t=1,08\)
\(\Leftrightarrow t=1,08:0,12\)
\(\Leftrightarrow t=9\)
Vậy năm nước ta đạt 10,05 triệu héc-ta là: \(2000+9=2009\)

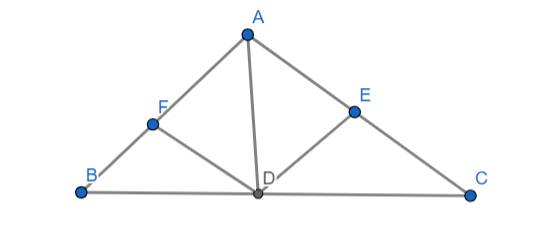
a) Ta có:
\(DF//AC\left(gt\right)\) (1)
\(DE//AB\left(gt\right)\) (2)
Từ (1) và (2) ⇒ AEDF là hình bình hành (3)
Mà AD là phân giác của góc FAE (4)
Từ (3) và (4) ⇒ AEDF là hình thoi
b) Xét hai tam giác CDE và CBA có:
\(\widehat{ACB}\) chung
\(\widehat{CED}=\widehat{CAB}\) (đồng vị vì DE//AB)
\(\Rightarrow\Delta CDE\sim\Delta CBA\left(g.g\right)\)
\(\Rightarrow\dfrac{DE}{AB}=\dfrac{CE}{AC}\Rightarrow DE\cdot AC=CE\cdot AB\)
Do: AEDF là hình thoi nên: DE = AE = AF
\(\Rightarrow AF\cdot AC=\left(AC-AE\right)\cdot AB\)
\(\Rightarrow\left(AB-BF\right)\cdot AC=AC\cdot AB-AE\cdot AB\)
\(\Rightarrow AB\cdot AC-BF\cdot AC=AC\cdot AB-AE\cdot AB\)
\(\Rightarrow BF\cdot AC=AE\cdot AB\)
\(\Rightarrow AF\cdot AB=BF\cdot AC\left(đpcm\right)\)



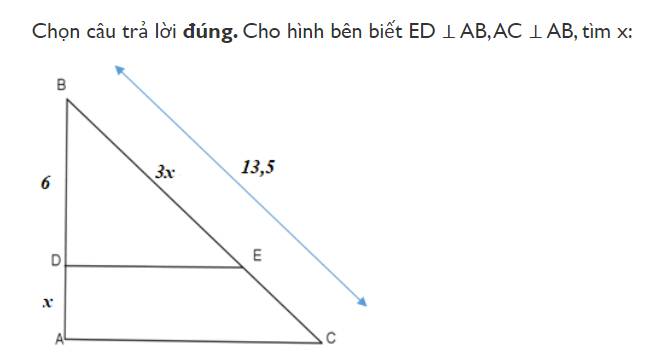
Ta có: DE//AC (cùng vuông góc với AB)
Áp dụng định lý Ta-lét ta có:
\(\dfrac{BD}{AD}=\dfrac{BE}{CE}\Rightarrow\dfrac{BD}{AD}=\dfrac{BE}{BC-BE}\Rightarrow\dfrac{6}{x}=\dfrac{3x}{13,5-3x}\)
\(\Leftrightarrow6\left(13,5-3x\right)=x\cdot3x\)
\(\Leftrightarrow81-18x=3x^2\)
\(\Leftrightarrow27-6x=x^2\)
\(\Leftrightarrow x^2+6x-27=0\)
\(\Leftrightarrow x^2-3x+9x-27=0\)
\(\Leftrightarrow x\left(x-3\right)+9\left(x-3\right)=0\)
\(\Leftrightarrow\left(x-3\right)\left(x+9\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\left(tm\right)\\x=-9\left(ktm\right)\end{matrix}\right.\)
Vậy: `x=3`
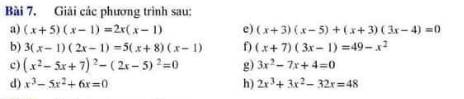
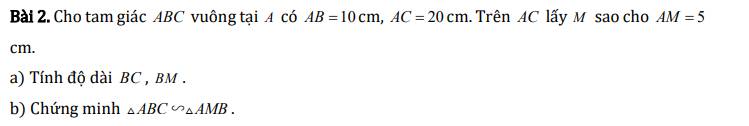
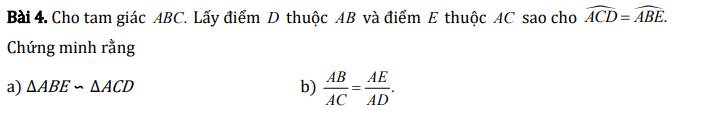





 Trả lời lẹ giúp em đi ạ
Trả lời lẹ giúp em đi ạ
a: \(\left(x+5\right)\left(x-1\right)=2x\left(x-1\right)\)
=>\(2x\left(x-1\right)-\left(x+5\right)\left(x-1\right)=0\)
=>\(\left(x-1\right)\left(2x-x-5\right)=0\)
=>(x-1)(x-5)=0
=>\(\left[{}\begin{matrix}x=1\\x=5\end{matrix}\right.\)
b: \(3\left(x-1\right)\left(2x-1\right)=5\left(x+8\right)\left(x-1\right)\)
=>\(\left(x-1\right)\left(6x-3\right)-\left(x-1\right)\left(5x+40\right)=0\)
=>\(\left(x-1\right)\left(6x-3-5x-40\right)=0\)
=>(x-1)(x-43)=0
=>\(\left[{}\begin{matrix}x=1\\x=43\end{matrix}\right.\)
c: \(\left(x^2-5x+7\right)^2-\left(2x-5\right)^2=0\)
=>\(\left(x^2-5x+7-2x+5\right)\left(x^2-5x+7+2x-5\right)=0\)
=>\(\left(x^2-7x+12\right)\left(x^2-3x+2\right)=0\)
=>(x-3)(x-4)(x-1)(x-2)=0
=>\(\left[{}\begin{matrix}x=3\\x=4\\x=1\\x=2\end{matrix}\right.\)
d: \(x^3-5x^2+6x=0\)
=>\(x\left(x^2-5x+6\right)=0\)
=>x(x-2)(x-3)=0
=>\(\left[{}\begin{matrix}x=0\\x=2\\x=3\end{matrix}\right.\)
e: \(\left(x+3\right)\left(x-5\right)+\left(x+3\right)\left(3x-4\right)=0\)
=>(x+3)(x-5+3x-4)=0
=>(x+3)(4x-9)=0
=>\(\left[{}\begin{matrix}x=-3\\x=\dfrac{9}{4}\end{matrix}\right.\)
f: \(\left(x+7\right)\left(3x-1\right)=49-x^2\)
=>\(\left(x+7\right)\left(3x-1\right)+x^2-49=0\)
=>(x+7)(3x-1)+(x-7)(x+7)=0
=>(x+7)(3x-1+x-7)=0
=>(x+7)(4x-8)=0
=>\(\left[{}\begin{matrix}x=-7\\x=2\end{matrix}\right.\)
g: \(3x^2-7x+4=0\)
=>\(3x^2-3x-4x+4=0\)
=>(x-1)(3x-4)=0
=>\(\left[{}\begin{matrix}x=1\\x=\dfrac{4}{3}\end{matrix}\right.\)
h: \(2x^3+3x^2-32x=48\)
=>\(2x^3+3x^2-32x-48=0\)
=>\(x^2\left(2x+3\right)-16\left(2x+3\right)=0\)
=>\(\left(2x+3\right)\left(x^2-16\right)=0\)
=>(2x+3)(x-4)(x+4)=0
=>\(\left[{}\begin{matrix}x=-\dfrac{3}{2}\\x=4\\x=-4\end{matrix}\right.\)
a) (x + 5)(x - 1) = 2x(x - 1)
(x + 5)(x - 1) - 2x(x - 1) = 0
(x - 1)(x + 5 - 2x) = 0
(x - 1)(5 - x) = 0
x - 1 = 0 hoặc 5 - x = 0
*) x - 1 = 0
x = 1
*) 5 - x = 0
x = 5
Vậy S = {1; 5}
b) 3(x - 1)(2x - 1) = 5(x + 8)(x - 1)
(x - 1)(6x - 3) = (5x + 40)(x - 1)
(x - 1)(6x - 3) - (5x + 40)(x - 1) = 0
(x - 1)(6x - 3 - 5x - 40) = 0
(x - 1)(x - 43) = 0
x - 1 = 0 hoặc x - 43 = 0
*) x - 1 = 0
x = 1
*) x - 43 = 0
x = 43
Vậy S = {1; 43}
c) (x² - 5x + 7)² - (2x - 5)² = 0
(x² - 5x + 7 - 2x + 5)(x² - 5x + 7 + 2x - 5) = 0
(x² - 7x + 12)(x² - 3x + 2) = 0
x² - 7x + 12 = 0 hoặc x² - 3x + 2 = 0
*) x² - 7x + 12 = 0
x² - 3x - 4x + 12 = 0
(x² - 3x) - (4x + 12) = 0
x(x - 3) - 4(x - 3) = 0
(x - 3)(x - 4) = 0
x - 3 = 0 hoặc x - 4 = 0
+) x - 3 = 0
x = 3
+) x - 4 = 0
x = 4
*) x² - 3x + 2 = 0
x² - x - 2x + 2 = 0
(x² - x) - (2x - 2) = 0
x(x - 1) - 2(x - 1) = 0
(x - 1)(x - 2) = 0
x - 1 = 0 hoặc x - 2 = 0
+) x - 1 = 0
x = 1
+) x - 2 = 0
x = 2
Vậy S = {1; 2; 3; 4}
d) x³ - 5x² + 6x = 0
x(x² - 5x + 6) = 0
x = 0 hoặc x² - 5x + 6 = 0
*) x² - 5x + 6 = 0
x² - 2x - 3x + 6 = 0
(x² - 2x) - (3x - 6) = 0
x(x - 2) - 3(x - 2) = 0
(x - 2)(x - 3) = 0
x - 2 = 0 hoặc x - 3 = 0
+) x - 2 = 0
x = 2
+) x - 3 = 0
x = 3
Vậy S = {0; 2; 3}