
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Chọn D
Ta có: 1 x ( x + 3 ) = 1 3 1 x - 1 x + 3
Nên ∫ f ( x ) d x = ∫ 1 3 1 x - 1 x + 3 d x = 1 3 ln x x + 3 + C

\(\int_0^2\left[4f_{\left(x\right)}-3\right]dx\)
= \(4\int_0f_{\left(x\right)}^2dx-3x|^2_0\)
= 12 - 6
= 6

Đáp án D
Phương pháp:
Sử dụng công thức từng phần:
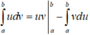
Cách giải:
![]()
![]()
![]()
![]()
![]()
![]()

Chọn A
Đặt u = ln x - 1 d v = 2 x d x ⇒ d u = 1 x - 1 d x v = x 2 - 1
![]()

a.
\(y'=-\dfrac{3}{2}x^3+\dfrac{6}{5}x^2-x+5\)
b.
\(y'=\dfrac{\left(x^2+4x+5\right)'}{2\sqrt{x^2+4x+5}}=\dfrac{2x+4}{2\sqrt{x^2+4x+5}}=\dfrac{x+2}{\sqrt{x^2+4x+5}}\)
c.
\(y=\left(3x-2\right)^{\dfrac{1}{3}}\Rightarrow y'=\dfrac{1}{3}\left(3x-2\right)^{-\dfrac{2}{3}}=\dfrac{1}{3\sqrt[3]{\left(3x-2\right)^2}}\)
d.
\(y'=2\sqrt{x+2}+\dfrac{2x-1}{2\sqrt{x+2}}=\dfrac{6x+7}{2\sqrt{x+2}}\)
e.
\(y'=3sin^2\left(\dfrac{\pi}{3}-5x\right).\left[sin\left(\dfrac{\pi}{3}-5x\right)\right]'=-15sin^2\left(\dfrac{\pi}{3}-5x\right).cos\left(\dfrac{\pi}{3}-5x\right)\)
g.
\(y'=4cot^3\left(\dfrac{\pi}{6}-3x\right)\left[cot\left(\dfrac{\pi}{3}-3x\right)\right]'=12cot^3\left(\dfrac{\pi}{6}-3x\right).\dfrac{1}{sin^2\left(\dfrac{\pi}{3}-3x\right)}\)

a. \(\int\dfrac{x^3}{x-2}dx=\int\left(x^2+2x+4+\dfrac{8}{x-2}\right)dx=\dfrac{1}{3}x^3+x^2+4x+8ln\left|x-2\right|+C\)
b. \(\int\dfrac{dx}{x\sqrt{x^2+1}}=\int\dfrac{xdx}{x^2\sqrt{x^2+1}}\)
Đặt \(\sqrt{x^2+1}=u\Rightarrow x^2=u^2-1\Rightarrow xdx=udu\)
\(I=\int\dfrac{udu}{\left(u^2-1\right)u}=\int\dfrac{du}{u^2-1}=\dfrac{1}{2}\int\left(\dfrac{1}{u-1}-\dfrac{1}{u+1}\right)du=\dfrac{1}{2}ln\left|\dfrac{u-1}{u+1}\right|+C\)
\(=\dfrac{1}{2}ln\left|\dfrac{\sqrt{x^2+1}-1}{\sqrt{x^2+1}+1}\right|+C\)
c. \(\int\left(\dfrac{5}{x}+\sqrt{x^3}\right)dx=\int\left(\dfrac{5}{x}+x^{\dfrac{3}{2}}\right)dx=5ln\left|x\right|+\dfrac{2}{5}\sqrt{x^5}+C\)
d. \(\int\dfrac{x\sqrt{x}+\sqrt{x}}{x^2}dx=\int\left(x^{-\dfrac{1}{2}}+x^{-\dfrac{3}{2}}\right)dx=2\sqrt{x}-\dfrac{1}{2\sqrt{x}}+C\)
e. \(\int\dfrac{dx}{\sqrt{1-x^2}}=arcsin\left(x\right)+C\)
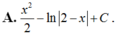
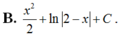
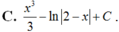
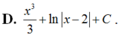
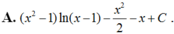
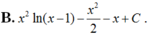
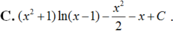
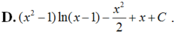
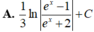
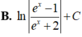
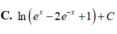
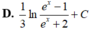
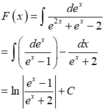
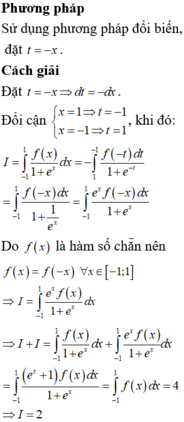
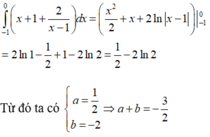
Ta có
- x 3 + 5 x + 2 4 - x 2 = x 3 - 5 x - 2 x 2 - 4 = ( x + 2 ) ( x 2 - 2 x - 1 ) ( x + 2 ) ( x - 2 ) = x - 1 x - 2
Nên
∫ - x 3 + 5 x + 2 4 - x 2 d x = ∫ x - 1 x - 2 d x = x 2 2 - ln 2 - x + C .
Chọn A.