Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Các bạn giúp mk làm 5 bài này nhé. mk đang cần gấp. Thanks các bạn nhiều
Mk cần gấp 5 bài này trong hôm nay. Các bạn cố gắng giúp mk. Thanks


c, là hằng đẳng thức nha bạn
(\(\sqrt{x}\)+\(\sqrt{2x}\))2=0
suy ra \(\sqrt{x}\)+\(\sqrt{2x}\)=0
\(\sqrt{x}\)=\(\sqrt{2x}\)
suy ra x=0
Bài 2: Tìm x:
a) \(3x^2\)\(-27x=0\)
\(< =>3x\left(x-9\right)=0\)
\(=>x=0\) hay \(x-9=0\)
\(=>x=0\) hay \(x=9\)

Bài 1:
a, 4x2+6x=2x(2x+3)
b, 12x(x-2y)-9y(x-2y)=3(x-2y)(4x-3y)
c, 3x3-6x2+3x=3x(x2-2x+1)=3x(x-1)2
d, 2x3-2xy2+12x2+18x=2x(x2-y2)+2x(6x+9)=2x(x2+6x+9-y2)
=2x[(x+3)2-y2 ]=2x(x+y+3)(x-y+3)
Bài 2:
a, 5x(x-1)+10x-10=0 <=> 5x(x-1)+10(x-1)=0 <=> 5(x-1)(x+2)=0
\(\Leftrightarrow\orbr{\begin{cases}5\left(x-1\right)=0\\x+2=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=1\\x=-2\end{cases}}}\)
b,(x+2)(x+3)-2x=6 <=> (x+2)(x+3)-2(x+3)=0 <=> (x+3)(x+2-2)=0 <=> x(x+3)=0
\(\Leftrightarrow\orbr{\begin{cases}x=0\\x+3=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=0\\x=-3\end{cases}}}\)
c, \(\left(x-1\right)\left(x-2\right)-2=0\Leftrightarrow x^2-3x+2-2=0\Leftrightarrow x\left(x-3\right)\)\(\Leftrightarrow\orbr{\begin{cases}x=0\\x-3=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=0\\x=3\end{cases}}}\)
Bài 3
a, \(x^4y+3x^3y^2+3x^2y^3+xy^4=xy\left(x^3+3x^2y+3xy^2+y^3\right)=xy\left(x+y\right)^3\)
b, \(x^4+4=x^4+4x^2+4-4x^2=\left(x^2+2\right)-\left(2x\right)^2=\left(x^2+2x+2\right)\left(x^2-2x+2\right)\)
hình học
Bài 1 \(\widehat{D}=360^o-\widehat{A}-\widehat{B}-\widehat{C}=360^o-50^o-120^o-90^o=100^o\)
Bài 2 \(Tc:\widehat{C}+\widehat{D}=360^o-\widehat{A}-\widehat{B}=360^o-50^o-110^o=200^o\)
\(\Rightarrow\widehat{C}=200^o-\widehat{D}\)mà \(\widehat{C}=3\widehat{D}\)nên ta có \(3\widehat{D}=200^o-\widehat{D}\Leftrightarrow4\widehat{D}=200^o\Leftrightarrow\widehat{D}=50^o\Rightarrow\widehat{C}=3.50^o=150^o\)
Bài 4 \(\widehat{C}+\widehat{D}=360^o-90^o-110^o=160^o\)
Áp dụng dãy tỉ số bằng nhau
\(\frac{\widehat{C}}{3}=\frac{\widehat{D}}{5}=\frac{\widehat{C}+\widehat{D}}{3+5}=\frac{160^0}{8}=30^o\)
\(\Rightarrow\frac{\widehat{C}}{3}=30^o\Rightarrow\widehat{C}=30^o.3=90^o\Rightarrow\widehat{D}=160^o-90^o=70^o\)


b) Bạn đã chứng minh được tứ giác EKFC là hình bình hành ở câu a, mà EF cắt CK tại I \(\Rightarrow\)I là trung điểm EF (tính chất hình bình hành)
\(\Rightarrow AI\)là trung tuyến của \(\Delta AEF\)
Mà \(\Delta AEF\)vuông tại A \(\Rightarrow AI=\frac{1}{2}EF\)(tính chất tam giác vuông)
Lại có \(EI=\frac{1}{2}EF\)do I là trung điểm của đoạn EF \(\Rightarrow AI=EI\left(=\frac{1}{2}EF\right)\)
Mặt khác \(BE\perp AF\), \(MI\perp AF\left(gt\right)\)\(\Rightarrow BE//MI\)(quan hệ từ vuông góc đến song song)
Mà tứ giác BEFD là hình bình hành \(\Rightarrow BD//EF\)(tính chất hình bình hành)
\(\Rightarrow BM//EI\)(vì \(M\in BD;I\in EF\))
Xét tứ giác BEIM có \(BE//MI\left(cmt\right);BM//EI\left(cmt\right)\)\(\Rightarrow\)Tứ giác BEIM là hình bình hành (định nghĩa)
\(\Rightarrow BM=EI\)(tính chất hình bình hành)
Mà \(AI=EI\left(cmt\right)\)\(\Rightarrow AI=BM\left(=EI\right)\left(đpcm\right)\)
c) Do tứ giác BEFD là hình bình hành \(\Rightarrow\hept{\begin{cases}BE//DF\\BE=DF\end{cases}}\)(tính chất hình bình hành)
Mà \(\hept{\begin{cases}BE\perp CF\\BE=CF\end{cases}}\left(gt\right)\Rightarrow\hept{\begin{cases}DF\perp CFtạiF\\DF=CF\end{cases}}\)\(\Rightarrow\)F nằm trên đường trung trực của đoạn CD và \(\Delta CDF\)vuông cân tại F
\(\Rightarrow\widehat{DCF}=45^0\)
\(\Delta ABC\)vuông cân tại A (gt) \(\Rightarrow\widehat{ACB}=45^0\)
\(\Rightarrow\widehat{BCD}=180^0-\widehat{ACB}-\widehat{DCF}=180^0-45^0-45^0=90^0\)
\(\Rightarrow\Delta BCD\)vuông tại C.
Xét hình thang BEFD (BE//DF) ta có I là trung điểm EF (cmt) và IM//BE (cmt) \(\Rightarrow\)M là trung điểm của đoạn BD
\(\Rightarrow\)CM là trung tuyến của \(\Delta BCD\)
Mặt khác \(\Delta BCD\)vuông tại C \(\Rightarrow CM=\frac{1}{2}BD\)(tính chát tam giác vuông)
Mà \(DM=\frac{1}{2}BD\)do M là trung điểm BD \(\Rightarrow DM=CM\left(=\frac{1}{2}BD\right)\)
\(\Rightarrow\)M nằm trên đường trung trực của đoạn CD.
Mà F cũng nằm trên đường trung trực của đoạn CD (cmt)
\(\Rightarrow\)MF là đường trung trực của đoạn CD \(\Rightarrow\)C đối xứng với D qua MF (đpcm)

Điều kiện:
\(x-1\ne0\Rightarrow x\ne1\)
\(x^3+x\ne0\Leftrightarrow x\ne0\)
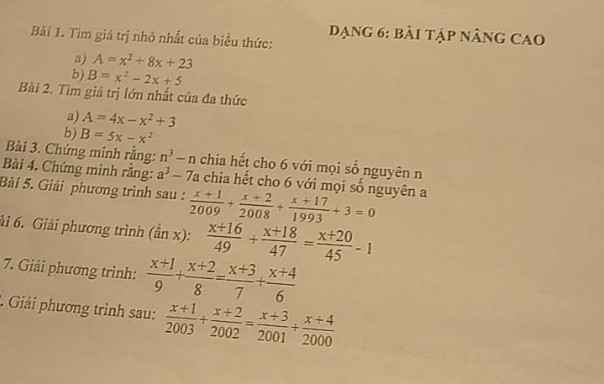



 giúp mk nha mờn nhìu ạk
giúp mk nha mờn nhìu ạk
 giải giúp mk bài 2 tìm x nhé(trừ câu a,b). Xin cảm ơn trước ạ
giải giúp mk bài 2 tìm x nhé(trừ câu a,b). Xin cảm ơn trước ạ

 GIÚP MÌNH BÀI 4 CÂU B,C THÔI Ạ MÌNH CẢM ƠN NHIỀU
GIÚP MÌNH BÀI 4 CÂU B,C THÔI Ạ MÌNH CẢM ƠN NHIỀU




 Mọi Người giải giúp em ạ em cảm ơn ạ
Mọi Người giải giúp em ạ em cảm ơn ạ
Bài 7:
\(\Leftrightarrow\dfrac{x+1}{9}+1+\dfrac{x+2}{8}+1=\dfrac{x+3}{7}+1+\dfrac{x+4}{6}+1\)
=>x+10=0
hay x=-10
Bài 8:
\(\Leftrightarrow\dfrac{x+1}{2003}+1+\dfrac{x+2}{2002}+1=\dfrac{x+3}{2001}+1+\dfrac{x+4}{2000}+1\)
=>x+2004=0
hay x=-2004