
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(11x^2-15x+4=0\)
\(\Leftrightarrow11x^2-11x-4x+4=0\)
\(\Leftrightarrow11x\left(x-1\right)-4\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(11x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\11x-4=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{4}{11}\end{matrix}\right.\)
\(S=\left\{1,\dfrac{4}{11}\right\}\)
Đặt C(x)=0
\(\Leftrightarrow11x^2-15x+4=0\)
\(\Leftrightarrow11x^2-11x-4x+4=0\)
\(\Leftrightarrow11x\left(x-1\right)-4\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(11x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\11x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\11x=4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{4}{11}\end{matrix}\right.\)
Vậy: Nghiệm của đa thức \(C\left(x\right)=11x^2-15x+4\) là 1 và \(\dfrac{4}{11}\)

Ta có: x+y+1=0
nên x+y=-1
Ta có: \(N=x^2\left(x+y\right)-y^2\left(x+y\right)+x^2-y^2+2\left(x+y\right)+3\)
\(=\left(x+y\right)\left(x^2-y^2\right)+\left(x^2-y^2\right)+2\left(x+y\right)+3\)
\(=\left(x^2-y^2\right)\left(x+y+1\right)+2\left(x+y\right)+3\)
\(=\left(x^2-y^2\right)\cdot0+2\cdot\left(-1\right)+3\)
=-2+3=1

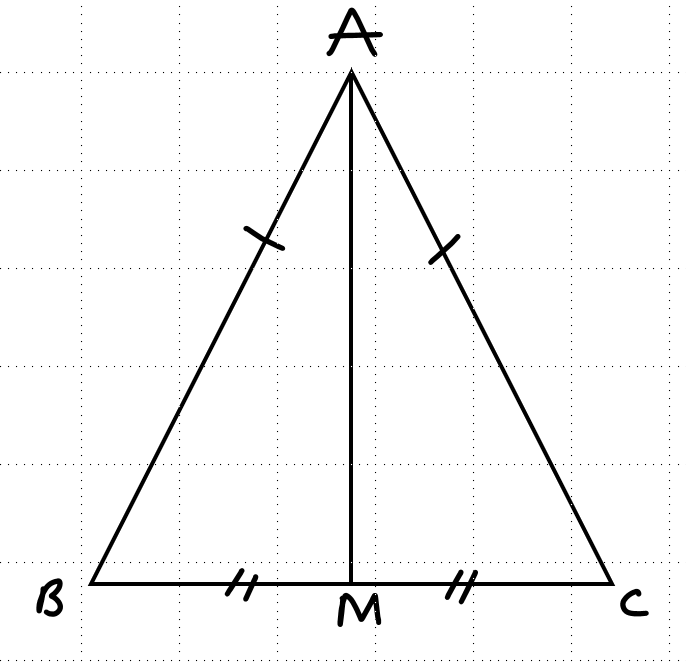
a. xét tam giác ABC có AB = AC (=17cm)
=> △ABC là △ cân tại A
lại có AM là đường trung tuyến
=> AM cũng là đường cao
=> AM vuông góc với BC
b. độ dài đoạn thẳng MB:
\(MB=\dfrac{1}{2}BC=\dfrac{1}{2}\cdot16=8\left(cm\right)\)
áp dụng định lý pythagore vào △BAM vuông tại M ta có:
\(AM=\sqrt{AB^2-BM^2}=\sqrt{17^2-8^2}=15\left(cm\right)\)

Đáp án:
P=\(\frac{2}{3}\)
Giải thích các bước giải:
x:y:z=5:4:3
⇒ x5x5 =y4y4 ⇒y= 4x54x5
⇒ x5x5 =z3z3 ⇒z= 3x53x5
Thay vào biểu thức ta được:
P= x+2y−3zx−2y+3zx+2y−3zx−2y+3z= x+2.4x5−33x5x−2.4x5+33x5x+2.4x5−33x5x−2.4x5+33x5 =4x56x54x56x5 =2323
Vậy P=\(\frac{2}{3}\)
# Chúc bạn học tốt!
Vì x,y,z tỉ lệ với các số 5,4,3 nên ta có : \(x:y:z=5:4:3\) hoặc \(\frac{x}{5}=\frac{y}{4}=\frac{z}{3}\)
Ta lại có : \(\frac{x}{5}=\frac{y}{4}=\frac{z}{3}=\frac{x}{5}=\frac{2y}{8}=\frac{3z}{9}\)
Đặt \(\frac{x}{5}=\frac{2y}{8}=\frac{3z}{9}=k\Rightarrow\hept{\begin{cases}x=5k\\2y=8k\\3z=9k\end{cases}}\)
\(P=\frac{x+2y-3z}{x-2y+3z}=\frac{5k+8k-9k}{5k-8k+9k}=\frac{4k}{6k}=\frac{4}{6}=\frac{2}{3}\)
Vậy \(P=\frac{2}{3}\)
B24:
a)
f(x)=9 - x5 + 4x - 2x3 +x2 -7x4
= - x5 -7x4 - 2x3 +4x +9
g(x)=x5 - 9 + 2x2 +7x4 +2x3
=x5 + 7x4 +2x3 +2x2 -9
f(x)= - x5 -7x4 - 2x3 +4x +9
+
g(x)=x5 + 7x4 +2x3 +2x2 -9
___________________________
h(x)= 2x2 +4x
b)
Để h=0 thì :
2x^2+2x=0
2x(x+1)=0
\(\orbr{\begin{cases}2x=0\\x+1=0\end{cases}}\)\(\Leftrightarrow\)\(\orbr{\begin{cases}x=0\\x=-1\end{cases}}\)
Vậy x=0 hoặc x=-1 là no của đa thức h(x)
7 nha dễ như ăn bánh