
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 38:
Xét ΔABD và ΔACB có
\(\frac{AB}{AC}=\frac{AD}{AB}\left(\frac{10}{20}=\frac{5}{10}=\frac12\right)\)
góc BAD chung
Do đó: ΔABD~ΔACB
=>\(\hat{ABD}=\hat{ACB}\)
Bài 36:
Xét ΔABD và ΔBDC có
\(\frac{AB}{BD}=\frac{BD}{DC}\left(\frac48=\frac{8}{16}=\frac12\right)\)
\(\hat{ABD}=\hat{BDC}\) (hai góc so le trong, AB//CD)
Do đó: ΔABD~ΔBDC
=>\(\hat{BAD}=\hat{DBC}\)
ΔABD~ΔBDC
=>\(\frac{AD}{BC}=\frac{AB}{BD}=\frac48=\frac12\)
=>BC=2AD
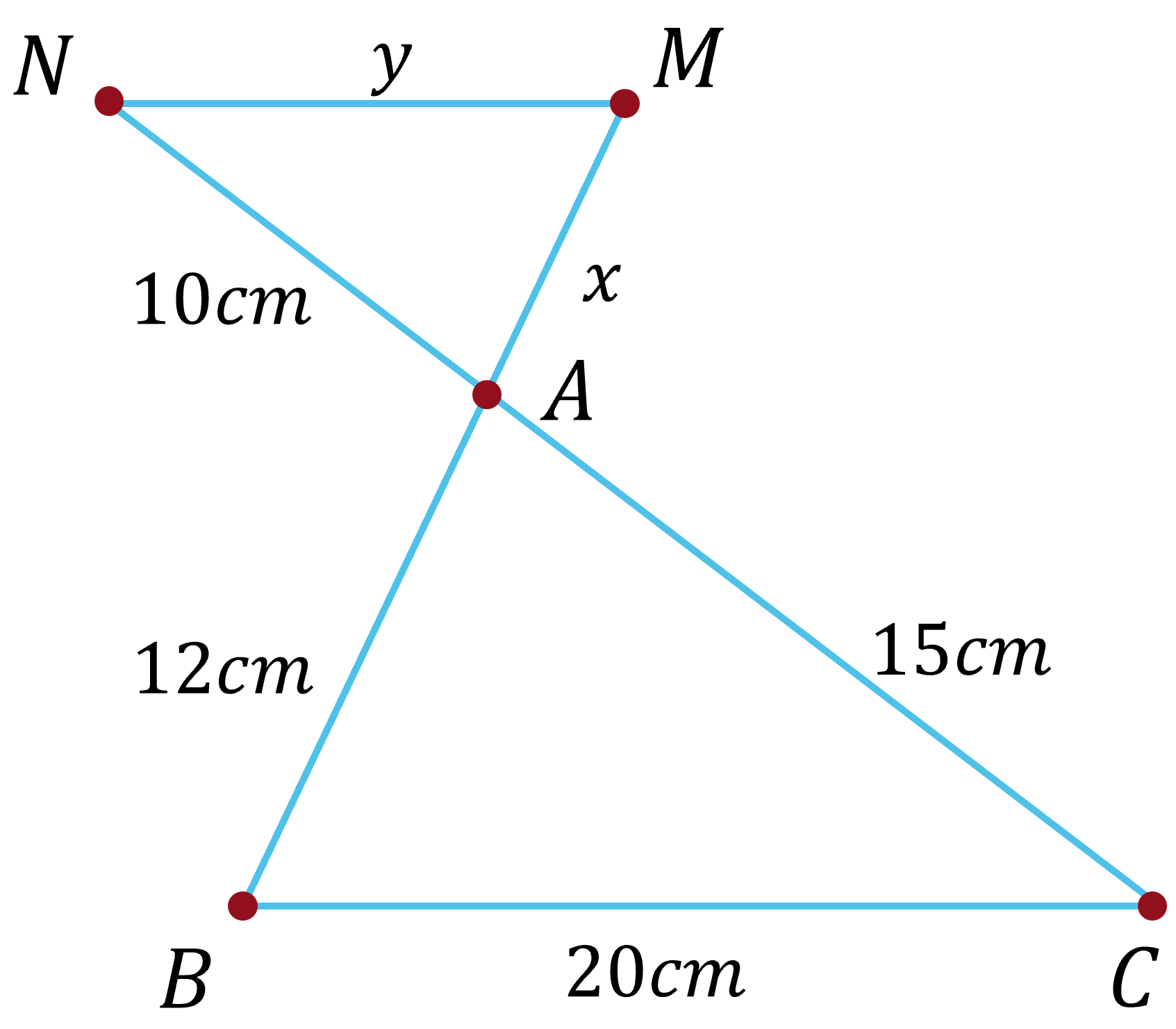
35:
Xét ΔAMN và ΔACB có
\(\frac{AM}{AC}=\frac{AN}{AB}\left(\frac{10}{15}=\frac{8}{12}=\frac23\right)\)
góc MAN chung
Do đó: ΔAMN~ΔACB
=>\(\frac{MN}{CB}=\frac{AM}{AC}=\frac23\)
=>\(MN=18\cdot\frac23=12\left(\operatorname{cm}\right)\)

a.
\(A=\left(\dfrac{\left(x-1\right)\left(x^2+x+1\right)}{x\left(x-1\right)}+\dfrac{\left(x-2\right)\left(x+2\right)}{x\left(x-2\right)}+\dfrac{x-2}{x}\right):\dfrac{x+1}{x}\)
\(=\left(\dfrac{x^2+x+1}{x}+\dfrac{x+2}{x}+\dfrac{x-2}{x}\right):\dfrac{x+1}{x}\)
\(=\left(\dfrac{x^2+3x+1}{x}\right).\dfrac{x}{x+1}\)
\(=\dfrac{x^2+3x+1}{x+1}\)
2.
\(x^3-4x^3+3x=0\Leftrightarrow x\left(x^2-4x+3\right)=0\)
\(\Leftrightarrow x\left(x-1\right)\left(x-3\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\left(loại\right)\\x=1\left(loại\right)\\x=3\end{matrix}\right.\)
Với \(x=3\Rightarrow A=\dfrac{3^2+3.3+1}{3+1}=\dfrac{19}{4}\)


14:
a: \(\frac{7x-1}{2x^2+6x}=\frac{7x-1}{2x\left(x+3\right)}=\frac{\left(7x-1\right)\left(x-3\right)}{2x\left(x+3\right)\left(x-3\right)}=\frac{7x^2-22x+3}{2x\left(x+3\right)\left(x-3\right)}\)
\(\frac{5-3x}{x^2-9}=\frac{2x\left(5-3x\right)}{2x\left(x-3\right)\left(x+3\right)}=\frac{10x-6x^2}{2x\left(x-3\right)\left(x+3\right)}\)
b: \(\frac{x+1}{x-x^2}=\frac{-\left(x+1\right)}{x^2-x}=\frac{-\left(x+1\right)}{x\left(x-1\right)}=\frac{-\left(x+1\right)\cdot2\left(x-1\right)}{2x\left(x-1\right)^2}=\frac{-2x^2+2}{2x\left(x-1\right)^2}\)
\(\frac{x+2}{2x^2-4x+2}=\frac{x+2}{2\left(x^2-2x+1\right)}=\frac{x+2}{2\left(x-1\right)^2}=\frac{x\left(x+2\right)}{2x\left(x-1\right)^2}=\frac{x^2+2x}{2x\left(x-1\right)^2}\)
c: \(\frac{4x^2-3x+5}{x^3-1}=\frac{4x^2-3x+5}{\left(x-1\right)\cdot\left(x^2+x+1\right)}\)
\(\frac{2x}{x^2+x+1}=\frac{2x\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\frac{2x^2-2x}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\frac{6}{x-1}=\frac{6\left(x^2+x+1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\frac{6x^2+6x+6}{\left(x-1\right)\left(x_{}^2+x+1\right)}\)
d: \(\frac{7}{5x}=\frac{7\cdot2\cdot\left(x-2y\right)\left(x+2y\right)}{5x\cdot2\cdot\left(x-2y\right)\left(x+2y\right)}=\frac{14\left(x^2-4y^2\right)}{10x\left(x-2y\right)\left(x+2y\right)}=\frac{14x^2-56y^2}{10x\left(x-2y\right)\left(x+2y\right)}\)
\(\frac{4}{x-2y}=\frac{4\cdot5x\cdot2\cdot\left(x+2y\right)}{\left(x-2y\right)\cdot5x\cdot2\cdot\left(x+2y\right)}=\frac{40x\left(x+2y\right)}{10x\left(x-2y\right)\left(x+2y\right)}=\frac{40x^2+80xy}{10x\left(x-2y\right)\left(x+2y\right)}\)
\(\frac{y-x}{8y^2-2x^2}=\frac{x-y}{2x^2-8y^2}=\frac{x-y}{2\left(x-2y\right)\left(x+2y\right)}=\frac{5x\left(x-y\right)}{2\cdot5x\left(x-2y\right)\left(x+2y\right)}=\frac{5x^2-5xy}{10x\left(x-2y\right)\left(x+2y\right)}\)
e: \(\frac{5x^2}{x^3+6x^2+12x+8}=\frac{5x^2}{\left(x+2\right)^3}=\frac{5x^2\cdot2}{2\left(x+2\right)^3}=\frac{10x^2}{2\left(x+2\right)^3}\)
\(\frac{4x}{x^2+4x+4}=\frac{4x}{\left(x+2\right)^2}=\frac{4x\cdot2\cdot\left(x+2\right)}{2\left(x+2\right)^3}=\frac{8x^2+16x}{2\left(x+2\right)^3}\)
\(\frac{3}{2x+4}=\frac{3}{2\left(x+2\right)}=\frac{3\left(x+2\right)^2}{2\left(x+2\right)^3}=\frac{3\left(x^2+4x+4\right)}{2\left(x+2\right)^3}=\frac{3x^2+12x+12}{2\left(x+2\right)^3}\)
13:
a: \(\frac{25}{14x^2y}=\frac{25\cdot3\cdot y^4}{14x^2y\cdot3y^4}=\frac{75y^4}{45x^2y^5}\)
\(\frac{14}{21xy^5}=\frac{14\cdot2\cdot x}{2x\cdot21xy^5}=\frac{28x}{42x^2y^5}\)
b: \(\frac{11}{102x^4y}=\frac{11\cdot y^2}{102x^4y\cdot y^2}=\frac{11y^2}{102x^4y^3}\)
\(\frac{3}{34xy^3}=\frac{3\cdot x^3\cdot3}{34xy^3\cdot3x^3}=\frac{9x^3}{102x^4y^3}\)
c: \(\frac{3x+1}{12xy^4}=\frac{\left(3x+1\right)\cdot3\cdot x}{12xy^4\cdot3x}=\frac{9x^2+3x}{36x^2y^4}\)
\(\frac{y-2}{9x^2y^3}=\frac{\left(y-2\right)\cdot4\cdot y}{9x^2y^3\cdot4y}=\frac{4y^2-8y}{36x^2y^4}\)
d: \(\frac{1}{6x^3y^2}=\frac{1\cdot6\cdot xy^2}{6x^3y^2\cdot6xy^2}=\frac{6xy^2}{36x^4y^4}\)
\(\frac{x+1}{9x^2y^4}=\frac{\left(x+1\right)\cdot4\cdot x^2}{9x^2y^4\cdot4x^2}=\frac{4x^3+4x^2}{36x^4y^4}\)
\(\frac{x-1}{4xy^3}=\frac{\left(x-1\right)\cdot9\cdot x^3y}{4xy^3\cdot9x^3y}=\frac{9x^4y-9x^3y}{36x^4y^4}\)
e: \(\frac{3+2x}{10x^4y}=\frac{\left(2x+3\right)\cdot4y^4}{10x^4y\cdot4y^4}=\frac{8xy^4+12y^4}{40x^4y^5}=\frac{3\left(8xy^4+12y^4\right)}{3\cdot40x^4y^4}=\frac{24xy^4+36y^4}{120x^4y^4}\)
\(\frac{5}{8x^2y^2}=\frac{5\cdot5\cdot x^2y^3}{8x^2y^2\cdot5x^2y^3}=\frac{25x^2y^3}{40x^4y^5}=\frac{25x^2y^3\cdot3}{40x^4y^5\cdot3}=\frac{75x^2y^3}{120x^4y^5}\)
\(\frac{2}{3xy^5}=\frac{2\cdot40\cdot x^3}{3xy^5\cdot40x^3}=\frac{80x^3}{120x^4y^5}\)
f: \(\frac{4x-4}{2x\left(x+3\right)}=\frac{2\cdot\left(x-1\right)}{2x\cdot\left(x+3\right)}=\frac{x-1}{x\left(x+3\right)}=\frac{\left(x-1\right)\cdot3\left(x+1\right)}{3x\left(x+3\right)\left(x+1\right)}=\frac{3x^2-3}{3x\left(x+3\right)\left(x+1\right)}\)
\(\frac{x-3}{3x\left(x+1\right)}=\frac{\left(x-3\right)\left(x+3\right)}{3x\left(x+1\right)\left(x+3\right)}=\frac{x^2-9}{3x\left(x+1\right)\left(x+3\right)}\)
g: \(\frac{2x}{\left(x+2\right)^3}=\frac{2x\cdot2x}{2x\left(x+2\right)^3}=\frac{4x^2}{2x\left(x+2\right)^3}\)
\(\frac{x-2}{2x\left(x+2\right)^2}=\frac{\left(x-2\right)\left(x+2\right)}{2x\left(x+2\right)^2\cdot\left(x+2\right)}=\frac{x^2-4}{2x\left(x+2\right)^3}\)
h: \(\frac{5}{3x^3-12x}=\frac{5}{3x\left(x^2-4\right)}=\frac{5}{3x\left(x-2\right)\left(x+2\right)}=\frac{5\cdot2\left(x+3\right)}{3x\left(x-2\right)\left(x+2\right)\cdot2\left(x+3\right)}=\frac{10x+30}{6x\left(x-2\right)\left(x+2\right)\left(x+3\right)}\)
\(\frac{3}{\left(2x+4\right)\left(x+3\right)}=\frac{3}{2\left(x+2\right)\left(x+3\right)}=\frac{3\cdot3x\left(x-2\right)}{2\left(x+2\right)\left(x+3\right)\cdot3x\left(x-2\right)}=\frac{9x^2-18x}{6x\left(x-2\right)\left(x+2\right)\left(x+3\right)}\)

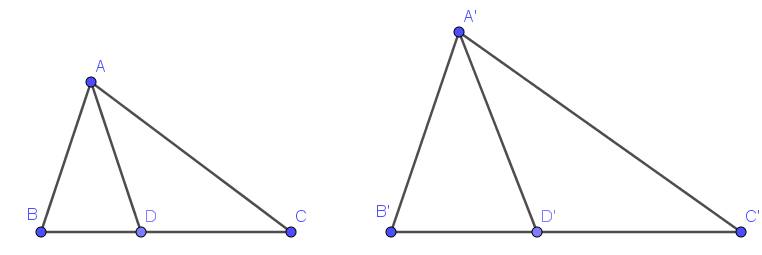
Bài 4:
a. Vì $\triangle ABC\sim \triangle A'B'C'$ nên:
$\frac{AB}{A'B'}=\frac{BC}{B'C'}=\frac{AC}{A'C'}(1)$ và $\widehat{ABC}=\widehat{A'B'C'}$
$\frac{DB}{DC}=\frac{D'B'}{D'C}$
$\Rightarrow \frac{BD}{BC}=\frac{D'B'}{B'C'}$
$\Rightarrow \frac{BD}{B'D'}=\frac{BC}{B'C'}(2)$
Từ $(1); (2)\Rightarrow \frac{BD}{B'D'}=\frac{BC}{B'C'}=\frac{AB}{A'B'}$
Xét tam giác $ABD$ và $A'B'D'$ có:
$\widehat{ABD}=\widehat{ABC}=\widehat{A'B'C'}=\widehat{A'B'D'}$
$\frac{AB}{A'B'}=\frac{BD}{B'D'}$
$\Rightarrow \triangle ABD\sim \triangle A'B'D'$ (c.g.c)
b.
Từ tam giác đồng dạng phần a và (1) suy ra:
$\frac{AD}{A'D'}=\frac{AB}{A'B'}=\frac{BC}{B'C'}$
$\Rightarrow AD.B'C'=BC.A'D'$

ĐKXĐ: \(\left|x-2\right|-1\ne0\)
\(\Rightarrow\left|x-2\right|\ne1\)
\(\Rightarrow\left\{{}\begin{matrix}x-2\ne1\\x-2\ne-1\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x\ne3\\x\ne1\end{matrix}\right.\)

Vì MN // BC theo Talet ta có:
\(\dfrac{y}{20}\) = \(\dfrac{10}{15}\) = \(\dfrac{x}{12}\) => x = \(\dfrac{10}{15}\) . 12 = 8; y = \(\dfrac{10}{15}\) . 20 = \(\dfrac{40}{3}\)

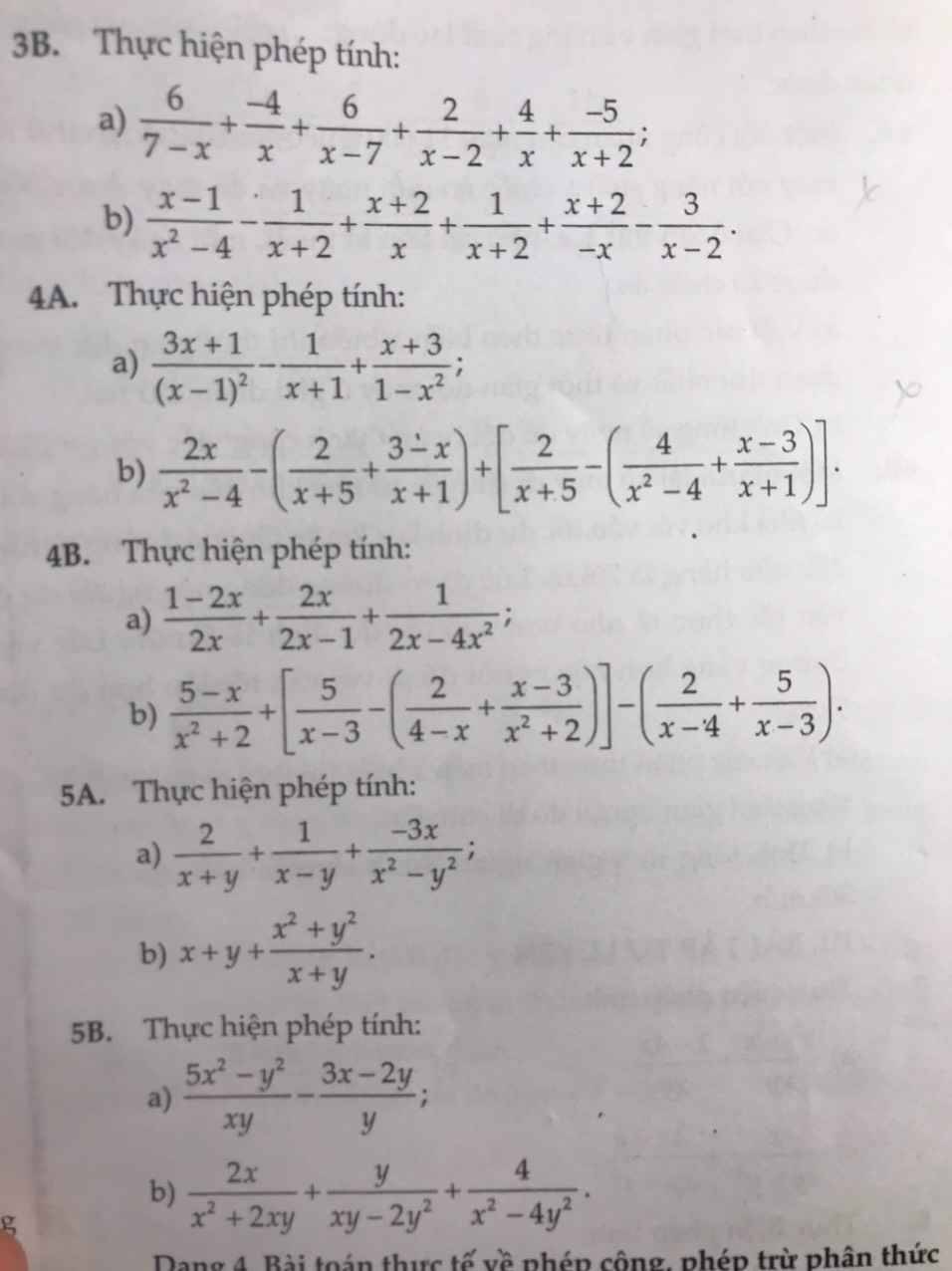 giúp mik bài3B 4A vs ạ
giúp mik bài3B 4A vs ạ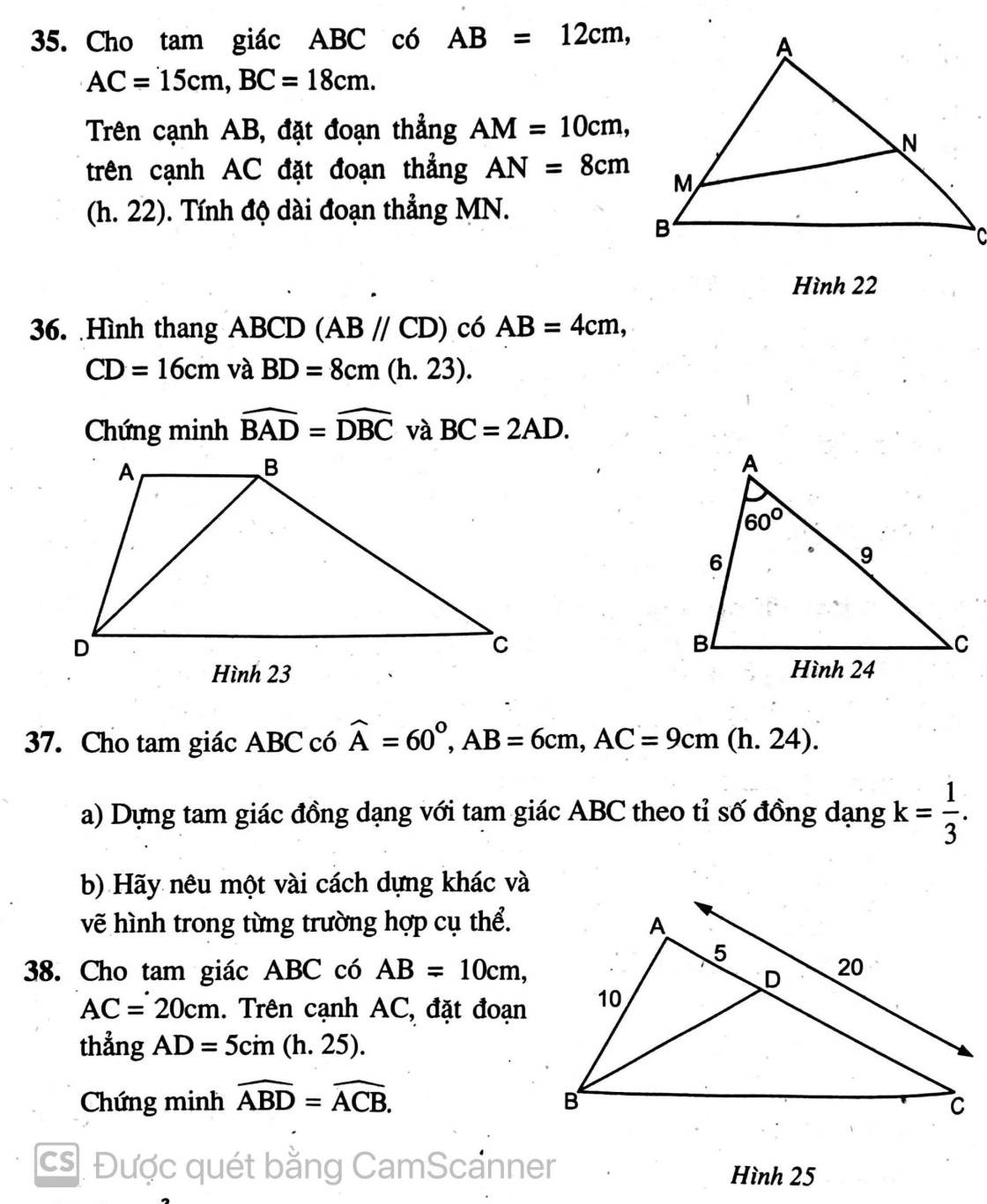
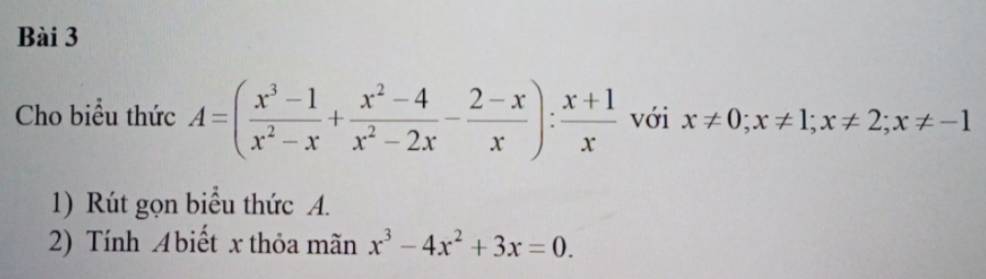


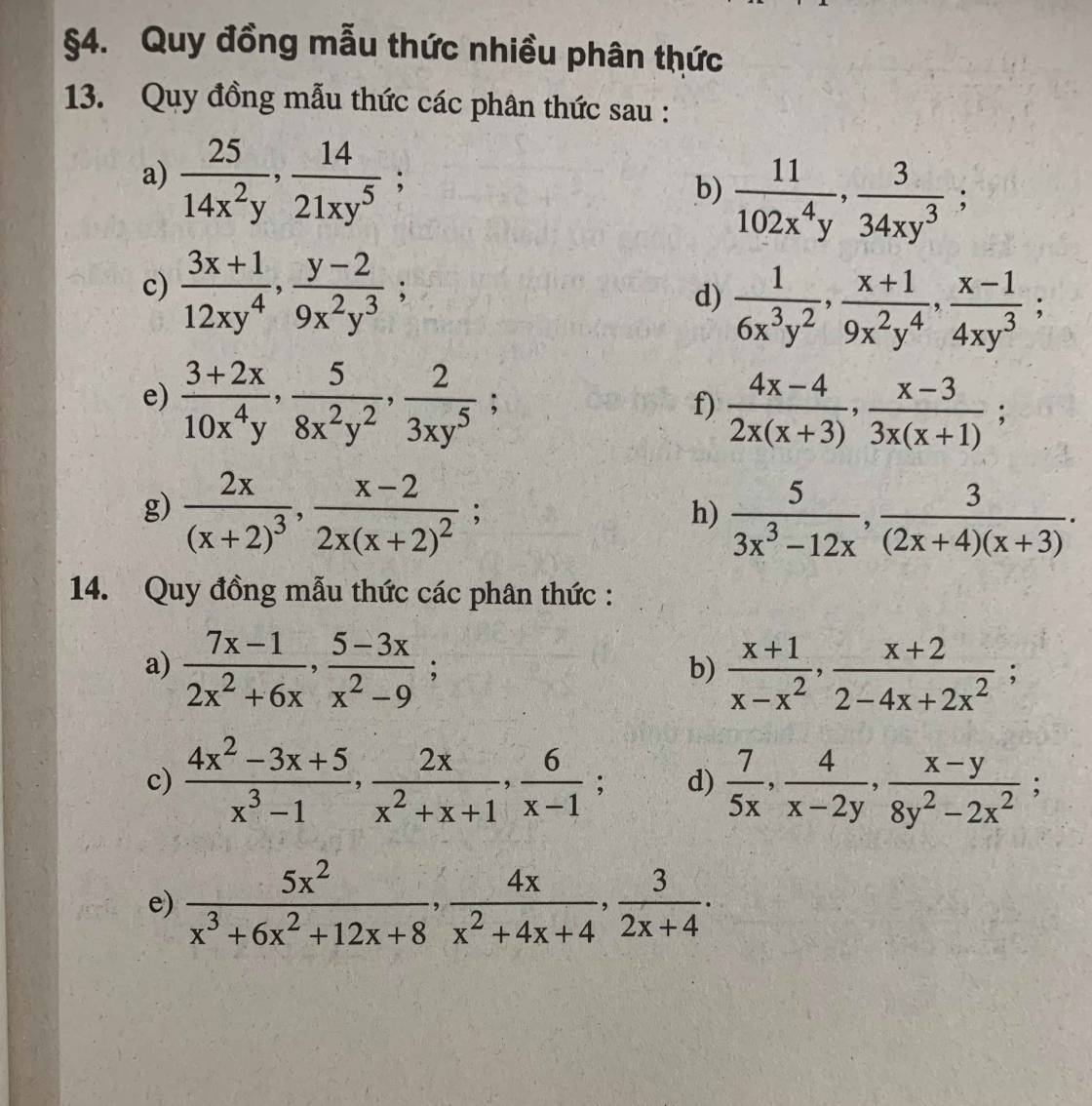

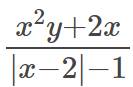
3B.
a.
\(\dfrac{6}{7-x}+\dfrac{-4}{x}+\dfrac{6}{x-7}+\dfrac{2}{x-2}+\dfrac{4}{x}+\dfrac{-5}{x+2}\)
\(=\dfrac{6}{7-x}-\dfrac{6}{7-x}+\dfrac{-4+4}{x}+\dfrac{2\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}+\dfrac{-5\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}\)
\(=0+0+\dfrac{2x+4-5x+10}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{-3x+14}{\left(x-2\right)\left(x+2\right)}\)
b.
\(\dfrac{x-1}{x^2-4}-\dfrac{1}{x+2}+\dfrac{x+2}{x}+\dfrac{1}{x+2}+\dfrac{x+2}{-x}-\dfrac{3}{x-2}\)
\(=\dfrac{x-1}{\left(x-2\right)\left(x+2\right)}-\dfrac{3\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}+\left(\dfrac{1}{x+2}-\dfrac{1}{x+2}\right)+\left(\dfrac{x+2}{x}-\dfrac{x+2}{x}\right)\)
\(=\dfrac{x-1-3x-6}{\left(x-2\right)\left(x+2\right)}+0+0\)
\(=\dfrac{-2x-7}{\left(x-2\right)\left(x+2\right)}\)
4A.
\(\dfrac{3x+1}{\left(x-1\right)^2}-\dfrac{1}{x+1}+\dfrac{x+3}{1-x^2}\)
\(=\dfrac{\left(3x+1\right)\left(x+1\right)}{\left(x+1\right)\left(x-1\right)^2}-\dfrac{\left(x-1\right)^2}{\left(x+1\right)\left(x-1\right)^2}-\dfrac{\left(x+3\right)\left(x-1\right)}{\left(x+1\right)\left(x-1\right)^2}\)
\(=\dfrac{3x^2+4x+1-\left(x^2-2x+1\right)-\left(x^2+2x-3\right)}{\left(x+1\right)\left(x-1\right)^2}\)
\(=\dfrac{x^2+4x+3}{\left(x+1\right)\left(x-1\right)^2}=\dfrac{\left(x+1\right)\left(x+3\right)}{\left(x+1\right)\left(x-1\right)^2}\)
\(=\dfrac{x+3}{\left(x-1\right)^2}\)
b.
\(\dfrac{2x}{x^2-4}-\left(\dfrac{2}{x+5}+\dfrac{3-x}{x+1}\right)+\left(\dfrac{2}{x+5}-\left(\dfrac{4}{x^2-4}+\dfrac{x-3}{x+1}\right)\right)\)
\(=\dfrac{2x}{x^2-4}+\left(\dfrac{2}{x+5}-\dfrac{2}{x+5}\right)+\left(\dfrac{x-3}{x+1}-\dfrac{x-3}{x+1}\right)-\dfrac{4}{x^2-4}\)
\(=\dfrac{2x-4}{x^2-4}=\dfrac{2\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{2}{x+2}\)