G
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
HH
3 tháng 7 2020
a) 4 ( x + 5 )( x + 6 )( x + 10 )( x + 12 ) = 3x2
Do x = 0 không là nghiệm pt nên chia 2 vế pt cho \(x^2\ne0\), ta được :
\(\frac{4}{x^2}\left(x^2+60+17x\right)\left(x^2+60+16x\right)=3\)
\(\Leftrightarrow4\left(x+\frac{60}{x}+17\right)\left(x+\frac{60}{x}+16\right)=3\)
Đến đây ta đặt \(x+\frac{60}{x}+16=t\left(1\right)\)
Ta được :
\(4t\left(t+1\right)=3\Leftrightarrow4t^2+4t-3=0\Leftrightarrow\left(2t+3\right)\left(2t-1\right)=0\)
Từ đó ta lắp vào ( 1 ) tính được x
1 tháng 2 2022
a: \(\Leftrightarrow2\sqrt{3x}+12-4x+5\sqrt{3}=0\)
\(\Leftrightarrow-4x+2\sqrt{3}\cdot\sqrt{x}+12+5\sqrt{3}=0\)
Đặt \(\sqrt{x}=a\left(a>=0\right)\)
Phương trình trở thành \(-4a^2+2\sqrt{3}a+12+5\sqrt{3}=0\)
\(\Delta=\left(2\sqrt{3}\right)^2-4\cdot\left(-4\right)\cdot\left(12+5\sqrt{3}\right)\)
\(=12+16\left(12+5\sqrt{3}\right)\)
\(=12+192+80\sqrt{3}=204+80\sqrt{3}\)
Vì Δ>0 nên phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}a_1=\dfrac{-2\sqrt{3}-\sqrt{204+80\sqrt{3}}}{-8}=\dfrac{2\sqrt{3}+\sqrt{204+80\sqrt{3}}}{8}\left(nhận\right)\\a_2=\dfrac{-2\sqrt{3}+\sqrt{204+80\sqrt{3}}}{-8}\left(loại\right)\end{matrix}\right.\)
\(\Leftrightarrow a=\dfrac{2\sqrt{3}+2\sqrt{26+20\sqrt{3}}}{8}=\dfrac{\sqrt{3}+\sqrt{26+20\sqrt{3}}}{4}\)
\(\Leftrightarrow x=a^2\simeq5,66\)
c: \(\Leftrightarrow x\sqrt{2}+5\sqrt{2}-4x-5-4\sqrt{2}=0\)
\(\Leftrightarrow x\left(\sqrt{2}-4\right)+\sqrt{2}-5=0\)
\(\Leftrightarrow x=\dfrac{5-\sqrt{2}}{\sqrt{2}-4}=\dfrac{-18-\sqrt{2}}{14}\)
d: \(\Leftrightarrow\dfrac{7x+1-4x-4002}{2001}=\dfrac{3x+2}{2003}-1\)
\(\Leftrightarrow3x-4001=0\)
hay x=4001/3
28 tháng 7 2019
a, \(\sqrt{x^2+2x-5}\)= \(\sqrt{2x-1}\)( x \(\ge\frac{1}{2}\))
\(\Leftrightarrow x^2+2x-5=2x-1\)
\(\Leftrightarrow x^2=4\)
\(\Leftrightarrow\orbr{\begin{cases}x=2\left(tm\right)\\x=-2\left(ktm\right)\end{cases}}\)
#mã mã#
28 tháng 7 2019
b, \(\sqrt{x\left(x^3-3x+1\right)}\)\(=\sqrt{x\left(x^3-x\right)}\)\(\left(x\ge1\right)\)
\(\Leftrightarrow x\left(x^3-3x+1\right)\)= \(x\left(x^3-1\right)\)
\(\Leftrightarrow\)x( x3 - 3x + 1 ) - x ( x3 - 1 ) = 0
\(\Leftrightarrow\)x ( x3 - 3x + 1 - x3 + 1 ) = 0
\(\Leftrightarrow\)x( 2-3x ) = 0
\(\Leftrightarrow\orbr{\begin{cases}x=0\\2-3x=0\end{cases}}\)\(\Leftrightarrow\orbr{\begin{cases}x=0\left(ktm\right)\\x=\frac{2}{3}\left(ktm\right)\end{cases}}\)
vậy pt vô nghiệm
#mã mã#
19 tháng 7 2019
a) \(1-\frac{2x+3}{7}=0\)
=> \(\frac{7-2x-3}{7}=0\)
=> \(\frac{4-2x}{7}=0\)
=> 4 - 2x = 0
=> 2x = 4
=> x = 4 : 2 = 2
19 tháng 7 2019
a) \(1-\frac{2x+3}{7}=0\)
\(\Leftrightarrow\frac{7}{7}-\frac{2x-3}{7}=0\)
\(\Leftrightarrow\frac{7-2x+3}{7}=0\)
\(\Leftrightarrow7-2x+3=0\)
\(\Leftrightarrow-2x+10=0\)
\(\Leftrightarrow-2\left(x-10\right)=0\)
\(\Leftrightarrow x-10=0\)
\(\Leftrightarrow x=10\)
17 tháng 5 2022
a: 3x+2>8
nên 3x>6
hay x>2
b: 4x-5<7
nên 4x<12
hay x<3
c: -2x+1<7
nên -2x<6
hay x>-3
d: -3x+13>-2
=>-3x>-15
hay x<5
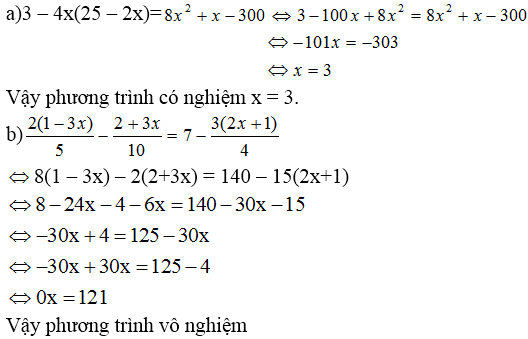
đK: \(x\ge-\frac{7}{3}\)
pt đã cho tương đương với: \(3x^2+3x-3x\sqrt{3x+7}-4x-4+4\sqrt{3x+7}+x\sqrt{3x+7}+\sqrt{3x+7}-3x-7=0\)
\(\Leftrightarrow3x\left(x+1-\sqrt{3x-7}\right)-4\left(x+1-\sqrt{3x+7}\right)+\sqrt{3x+7}\left(x+1-\sqrt{3x+7}\right)=0\)
\(\Leftrightarrow\left(x+1-\sqrt{3x+7}\right)\left(3x-4+\sqrt{3x+7}\right)=0\)
ta có: \(\sqrt{3x+7}=x+1\Leftrightarrow\hept{\begin{cases}3x+7=\left(x+1\right)^2\\x\ge-1\end{cases}}\Leftrightarrow x=3\)
Và \(\sqrt{3x+7}=4-3x\Leftrightarrow\hept{\begin{cases}3x+7=\left(4-3x\right)^2\\\frac{-7}{3}\le x\le\frac{4}{3}\end{cases}\Rightarrow x=\frac{3-\sqrt{5}}{2}}\)