
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


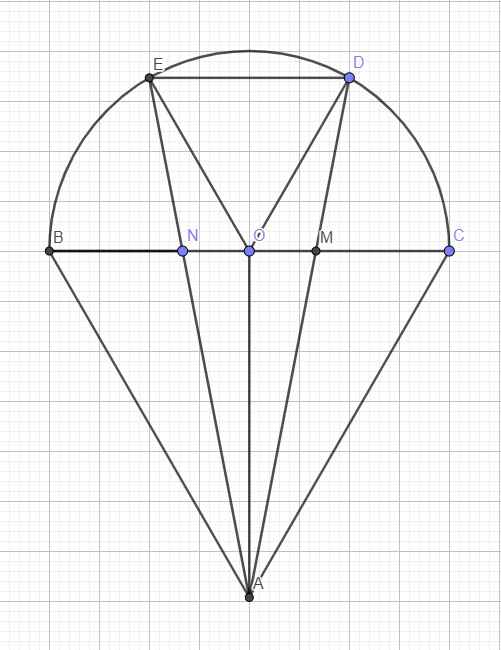
Gọi O là tâm đường tròn \(\Rightarrow\) O là trung điểm BC
\(\stackrel\frown{BE}=\stackrel\frown{ED}=\stackrel\frown{DC}\Rightarrow\widehat{BOE}=\widehat{EOD}=\widehat{DOC}=\dfrac{180^0}{3}=60^0\)
Mà \(OD=OE=R\Rightarrow\Delta ODE\) đều
\(\Rightarrow ED=R\)
\(BN=NM=MC=\dfrac{2R}{3}\Rightarrow\dfrac{NM}{ED}=\dfrac{2}{3}\)
\(\stackrel\frown{BE}=\stackrel\frown{DC}\Rightarrow ED||BC\)
Áp dụng định lý talet:
\(\dfrac{AN}{AE}=\dfrac{MN}{ED}=\dfrac{2}{3}\Rightarrow\dfrac{EN}{AN}=\dfrac{1}{2}\)
\(\dfrac{ON}{BN}=\dfrac{OB-BN}{BN}=\dfrac{R-\dfrac{2R}{3}}{\dfrac{2R}{3}}=\dfrac{1}{2}\)
\(\Rightarrow\dfrac{EN}{AN}=\dfrac{ON}{BN}=\dfrac{1}{2}\) và \(\widehat{ENO}=\widehat{ANB}\) (đối đỉnh)
\(\Rightarrow\Delta ENO\sim ANB\left(c.g.c\right)\)
\(\Rightarrow\widehat{NBA}=\widehat{NOE}=60^0\)
Hoàn toàn tương tự, ta có \(\Delta MDO\sim\Delta MAC\Rightarrow\widehat{MCA}=\widehat{MOD}=60^0\)
\(\Rightarrow\Delta ABC\) đều


đây là bài lớp 10 chứ nhỉ
ta có \(AC=20\times2=40\text{ hải lí}\), \(AB=15\times2=30\text{ hải lí}\)
áp dụng định lý cosin ta có :
\(BC=\sqrt{AB^2+AC^2-2AB.AC\text{c}osA}=\sqrt{40^2+30^2-2\times30\times40\times cos60^o}\simeq36.06\text{ hải lí}\)


\(\left(d\right):\frac{x}{a}+\frac{y}{b}=1\)\(\left(1\right)\)
Thế \(x=a,y=0\)vào phương trình \(\left(1\right)\)thỏa mãn nên \(A\left(a,0\right)\)thuộc \(\left(d\right)\).
Thế \(x=0,y=b\)vào phương trình \(\left(1\right)\)thỏa mãn nên \(B\left(0,b\right)\)thuộc \(\left(d\right)\).
Do đó ta có đpcm.

1.3 Giải phương trình:
a) \(\sqrt{2x+3}=1+\sqrt{2}\)(ĐK: \(x\ge-\frac{3}{2}\))
\(\Leftrightarrow2x+3=\left(1+\sqrt{2}\right)^2=3+2\sqrt{2}\)
\(\Leftrightarrow2x=2\sqrt{2}\)
\(\Leftrightarrow x=\sqrt{2}\)(tm)
b) \(\sqrt{x+1}=\sqrt{5}+3\)(ĐK: \(x\ge-1\))
\(\Leftrightarrow x+1=\left(\sqrt{5}+3\right)^2=14+6\sqrt{5}\)
\(\Leftrightarrow x=13+6\sqrt{5}\)(tm)
c) \(\sqrt{3x-2}=2-\sqrt{3}\)(ĐK: \(x\ge\frac{2}{3}\))
\(\Leftrightarrow3x-2=\left(2-\sqrt{3}\right)^2=7-4\sqrt{3}\)
\(\Leftrightarrow x=\frac{9-4\sqrt{3}}{3}\)(tm)
1.4: Phân tích thành nhân tử:
a) \(ab+b\sqrt{a}+\sqrt{a}+1=b\sqrt{a}\left(\sqrt{a}+1\right)+\left(\sqrt{a}+1\right)=\left(b\sqrt{a}+1\right)\left(\sqrt{a}+1\right)\)
b) \(\sqrt{x^3}-\sqrt{y^3}+\sqrt{x^2y}-\sqrt{xy^2}=x\sqrt{x}-y\sqrt{y}+x\sqrt{y}-y\sqrt{x}\)
\(=\left(x-y\right)\left(\sqrt{x}+\sqrt{y}\right)\)


Bài 1:
Kẻ \(OM\perp AB\), \(OM\)cắt \(CD\)tại \(N\).
Khi đó \(MN=8cm\).
TH1: \(AB,CD\)nằm cùng phía đối với \(O\).
\(R^2=OC^2=ON^2+CN^2=h^2+\left(\frac{25}{2}\right)^2\)(\(h=CN\)) (1)
\(R^2=OA^2=OM^2+AM^2=\left(h+8\right)^2+\left(\frac{15}{2}\right)^2\)(2)
Từ (1) và (2) suy ra \(R=\frac{\sqrt{2581}}{4},h=\frac{9}{4}\).
TH2: \(AB,CD\)nằm khác phía với \(O\).
\(R^2=OC^2=ON^2+CN^2=h^2+\left(\frac{25}{2}\right)^2\)(\(h=CN\)) (3)
\(R^2=OA^2=OM^2+AM^2=\left(8-h\right)^2+\left(\frac{15}{2}\right)^2\)(4)
Từ (3) và (4) suy ra \(R=\frac{\sqrt{2581}}{4},h=\frac{-9}{4}\)(loại).
Bài 3:
Lấy \(A'\)đối xứng với \(A\)qua \(Ox\), khi đó \(A'\)có tọa độ là \(\left(1,-2\right)\).
\(MA+MB=MA'+MB\ge A'B\)
Dấu \(=\)xảy ra khi \(M\)là giao điểm của \(A'B\)với trục \(Ox\).
Suy ra \(M\left(\frac{5}{3},0\right)\).

a, \(P=\frac{a^3-a+2b-\frac{b^2}{a}}{\left(1-\sqrt{\frac{a+b}{a^2}}\right)\left(a+\sqrt{a+b}\right)}:\left[\frac{a^2\left(a+b\right)+a\left(a+b\right)}{\left(a-b\right)\left(a+b\right)}+\frac{b}{a-b}\right]\)
\(=\frac{\frac{a^4-a^2-2ab-b^2}{a}}{\frac{\left(a-\sqrt{a+b}\right)\left(a+\sqrt{a+b}\right)}{a}}:\left[\frac{\left(a+b\right)\left(a^2+a\right)}{\left(a+b\right)\left(a-b\right)}+\frac{b}{a-b}\right]\)
\(=\frac{a^4-a^2-2ab-b^2}{a^2-a-b}:\frac{a^2+a+b}{a-b}\)
\(=\frac{a^4-a^2-2ab-b^2}{a^2-\left(a+b\right)}.\frac{a-b}{a^2+\left(a+b\right)}\)
\(=\frac{\left(a^4-a^2-2ab-b^2\right).\left(a-b\right)}{a^4-\left(a+b\right)^2}=\frac{\left[a^4-\left(a+b\right)^2\right].\left(a-b\right)}{a^4-\left(a+b\right)^2}=a-b\)
b, Có \(P=a-b=1\)\(\Rightarrow a=1+b\)
\(a^3-b^3=7\Leftrightarrow\left(a^2+ab+b^2\right)\left(a-b\right)=7\)
\(\Rightarrow a^2+ab+b^2=7\)
\(\Leftrightarrow\left(1+b\right)^2+\left(1+b\right)b+b^2=7\)
\(\Leftrightarrow b^2+2b+1+b^2+b+b^2=7\)
\(\Leftrightarrow3b^2+3b-6=0\)
Bạn tự giải phương trình tìm b => a
Bài 2 :
\(a,y=\left(m+1\right)x-2m-5\) \(\Leftrightarrow\left(m+1\right)x-2m-5-y=0\)
\(\Leftrightarrow mx+x-2m-5-y=0\)\(\Leftrightarrow m\left(x-2\right)+x-y-5=0\)
Có y luôn qua điểm A cố định với A( x0 ; y0 ) \(\orbr{\begin{cases}x_0-2=0\\x_0-y_0-5=0\end{cases}}\Rightarrow\orbr{\begin{cases}x_0=2\\y_0=-3\end{cases}}\)
=> A( 2;-3)
Gọi H là chân đường vuông góc hạ từ O xuống d => \(OH\le OA\)
\(OH_{max}=OA\)khi \(H\equiv A\)\(\left(d\perp OA\right)\)
=> đường thẳng OA qua O( 0;0 ) và A( 2;-3 ) => \(y=-\frac{3}{2}x\)
\(\Rightarrow d\perp OA\)=> hệ số góc \(m.\) \(-\frac{3}{2}=-1\Rightarrow m=\frac{2}{3}\)
b, \(y=0\Rightarrow\left(m+1\right)x-2m-5=0\)\(\Rightarrow x=\frac{2m+5}{m+1}\)\(\Rightarrow A\left(\frac{2m+5}{m+1};0\right)\)
\(x=0\Rightarrow y=-2m-5\Rightarrow B\left(0;-2m-5\right)\)
\(\Rightarrow OA=\sqrt{\frac{2m+5}{m+1}};OB=\sqrt{-2m-5}\)
\(\Rightarrow\frac{1}{2}.OA.OB=\frac{3}{2}\Rightarrow OA.OB=3\)
\(\Rightarrow\left(OA.OB\right)^2=9\Rightarrow\frac{\left(2m+5\right)^2}{m+1}=9\)
\(\Rightarrow4m^2+20m+25-9m-9=\)
\(\Rightarrow4m^2+11m+16=0\)



 Giải giúp mình với ạ, cần gấp lắm, cảm ơn
Giải giúp mình với ạ, cần gấp lắm, cảm ơn
 ai giúp mình giải câu này với ạ, mình cám ơn mn nhiều
ai giúp mình giải câu này với ạ, mình cám ơn mn nhiều
 ai giúp mình bài này với, mình cảm ơn nhiều
ai giúp mình bài này với, mình cảm ơn nhiều 



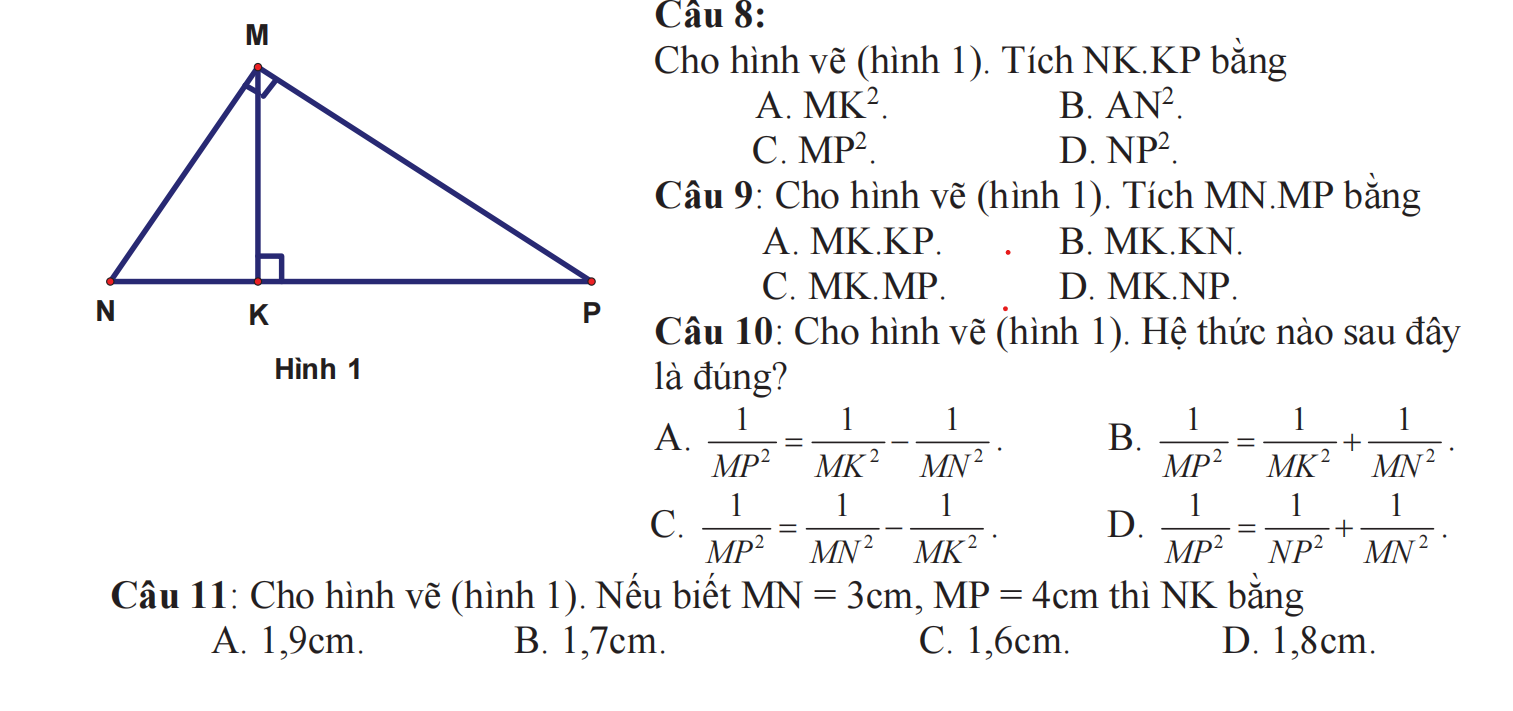
8.A
9. D
10. A
11. D
8A 9D 10 Hệ thức đúng: \(\dfrac{1}{MK^2}=\dfrac{1}{MN^2}+\dfrac{1}{MP^2}\)(k thấy trong các câu chọn)
11D