giải giúp mình nhé, cảm ơn mọi người và vote 5 điểm nha
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
H9
HT.Phong (9A5)
CTVHS
1 tháng 4 2023
1. \(\left(2x^2-3x+1\right)+\left(3x^2+2x-1\right)\)
\(=2x^2-3x+1+3x^2+2x-1\)
\(=\left(2x^2+3x^2\right)-\left(3x-2x\right)+\left(1-1\right)\)
\(=5x^2-x\)
2. \(\left(4x^3-2x^2+3x\right)-\left(2x^3+3x^2-4x\right)\)
\(=4x^3-2x^2+3x-2x^3-3x^2+4x\)
\(=\left(4x^3-2x^3\right)-\left(2x^2+3x^2\right)+\left(3x+4x\right)\)
\(=2x^3-5x^2+7x\)
3. \(\left(x^2-5x+6\right)+\left(-3x^2+2x-1\right)\)
\(=x^2-5x+6-3x^2+2x-1\)
\(=\left(x^2-3x^2\right)-\left(5x-2x\right)+\left(6-1\right)\)
\(=-2x^2-3x+5\)
H9
HT.Phong (9A5)
CTVHS
1 tháng 4 2023
4. \(\left(2x^3+5x^2-3x+1\right)-\left(x^3-2x^2+x-1\right)\)
\(=2x^3+5x^2-3x+1-x^3+2x^2-x+1\)
\(=\left(2x^3-x^3\right)+\left(5x^2+2x^2\right)-\left(3x+x\right)+\left(1+1\right)\)
\(=x^3+7x^2-4x+2\)
5. \(\left(3x^2+2x-4\right)+\left(4x^2-x+5\right)\)
\(=3x^2+2x-4+4x^2-x+5\)
\(=\left(3x^2+4x^2\right)+\left(2x-x\right)-\left(4-5\right)\)
\(=7x^2+x+1\)
6. \(\left(x^3-2x^2+5x-1\right)-\left(2x^3+3x^2-4x+2\right)\)
\(=x^3-2x^2+5x-1-2x^3-3x^2+4x-2\)
\(=\left(x^3-2x^3\right)-\left(2x^2+3x^2\right)+\left(5x+4x\right)-\left(1+2\right)\)
\(=-x^3-5x^2+9x-3\)
N
5
24 tháng 9 2021
\(\Rightarrow2^{3n-n}=16=2^4\Rightarrow2n=4\Rightarrow n=2\)
XO
10 tháng 8 2020
Ta có : \(\frac{3}{x-1}=\frac{4}{y-2}=\frac{5}{z-3}\Rightarrow1:\frac{3}{x-1}=1:\frac{4}{y-2}=1:\frac{5}{z-3}\)
\(\Rightarrow\frac{x-1}{3}=\frac{y-2}{4}=\frac{z-3}{5}\)
Đặt \(\frac{x-1}{3}=\frac{y-2}{4}=\frac{z-3}{5}=k\Rightarrow\hept{\begin{cases}x=3k+1\\y=4k+2\\z=5k+3\end{cases}}\)
Khi đó x + y + z = 18
<=> 3k + 1 + 4k + 2 + 5k + 3 = 18
=> 12k + 6 = 18
=> 12k = 12
=> k = 1
=> x = 4 ; y = 6 ; z = 8
F
11 tháng 8 2020
Bài giải
Áp dụng tính chất của dãy tỉ số bằng nhau, ta có :
\(\frac{3}{x-1}=\frac{4}{y-2}=\frac{5}{z-3}=\frac{3+4+5}{x-1+y-2+z-3}=\frac{12}{12}=1\)
\(\Rightarrow\text{ }\hept{\begin{cases}x=3\text{ : }1+1=4\\y=4\text{ : }1+2=6\\z=5\text{ : }1+3=8\end{cases}}\)
\(\Rightarrow\text{ }x=4\text{ ; }y=6\text{ ; }z=8\)
LT
3
7 tháng 2 2017
x + y + xy = 2
x + y(x + 1) = 2
x + 1 + y(x + 1) = 3
(x + 1)(y + 1) = 3
=> x + 1 và y + 1 thuộc ước của 3
Ư(3) = { - 3; - 1; 1; 3 }
Ta có bảng sau :
| x + 1 | - 3 | - 1 | 3 | 1 |
| y + 1 | - 1 | - 3 | 1 | 3 |
| x | - 4 | - 2 | 2 | 0 |
| y | - 2 | - 4 | 0 | 2 |
Vậy ( x;y ) = { ( -4;-2 );( -2;-4 );( 2;0 );( 0;2 ) }
BV
4
15 tháng 4 2023
Bài 1
1.\(x\left(x+3\right)\)
\(=x^2+3x\)
2.\(3x\left(x+2\right)\)
\(=3x^2+6x\)
3,\(x^2\left(3x-1\right)\)
\(=3x^3-x^2\)
4.\(-5x^3\left(3x^2-7\right)\)
\(=-15x^5+35x^3\)
5.\(3x\left(5x^2-2x-1\right)\)
\(=15x^3-6x^2-3x\)
6.\(-x^2\left(5x^3-x-\dfrac{1}{2}\right)\)
\(=-5x^5+x^3+\dfrac{x^2}{2}\)
7.\(\left(x^2+2x-3\right).\left(-x\right)\)
\(=-x^3-2x^2+3x\)
8.\(4x^3\left(-2x^2+4x^4-3\right)\)
\(=-8x^5+16x^7-12x^3\)
9.\(-5x^2\left(3x^2-2x+1\right)\)
\(=-15x^4+10x^3-5x^2\)
10.\(-4x^5\left(x^3-4x^2+7x-3\right)\)
\(=-4x^8+16x^7-28x^6+12x^5\)
11.\(\left(x+2\right)\left(x+3\right)\)
\(=x^2+3x+2x+6\)
12.\(\left(x-7\right)\left(x-5\right)\)
\(=x^2-5x-7x+35\)
13.\(\left(3x+5\right)\left(2x-7\right)\)
\(=6x^2-21x+10x-35\)
14.\(\left(x-3\right)\left(x^2-2x-1\right)\)
\(x^3-2x^2-x-3x^2+6x+3\)
15.\(\left(2x-1\right)\left(x^2-5x+3\right)\)
\(=2x^3-10x^2+6x-x^2+5x-3\)
16.\(\left(x-5\right)\left(-x^2+x-1\right)\)
\(=-x^3+x^2-x+5x^2-5x+5\)
17,\(\left(\dfrac{1}{2}x+3\right)\left(2x^2-4x-6\right)\)
\(=x^3-2x^2-3x+6x^2-12x-18\)
P/s:mình làm hơi tắt tại bài dài quá:))
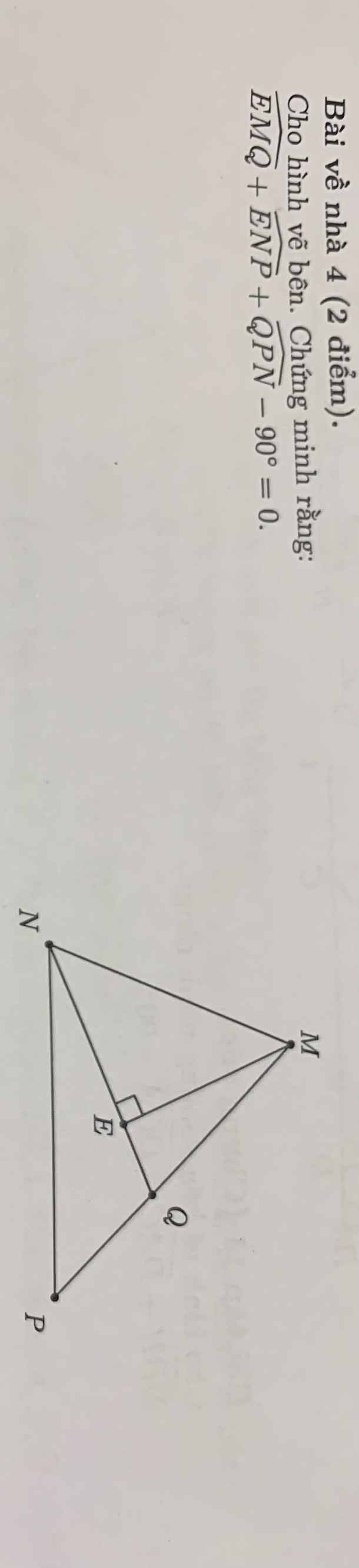
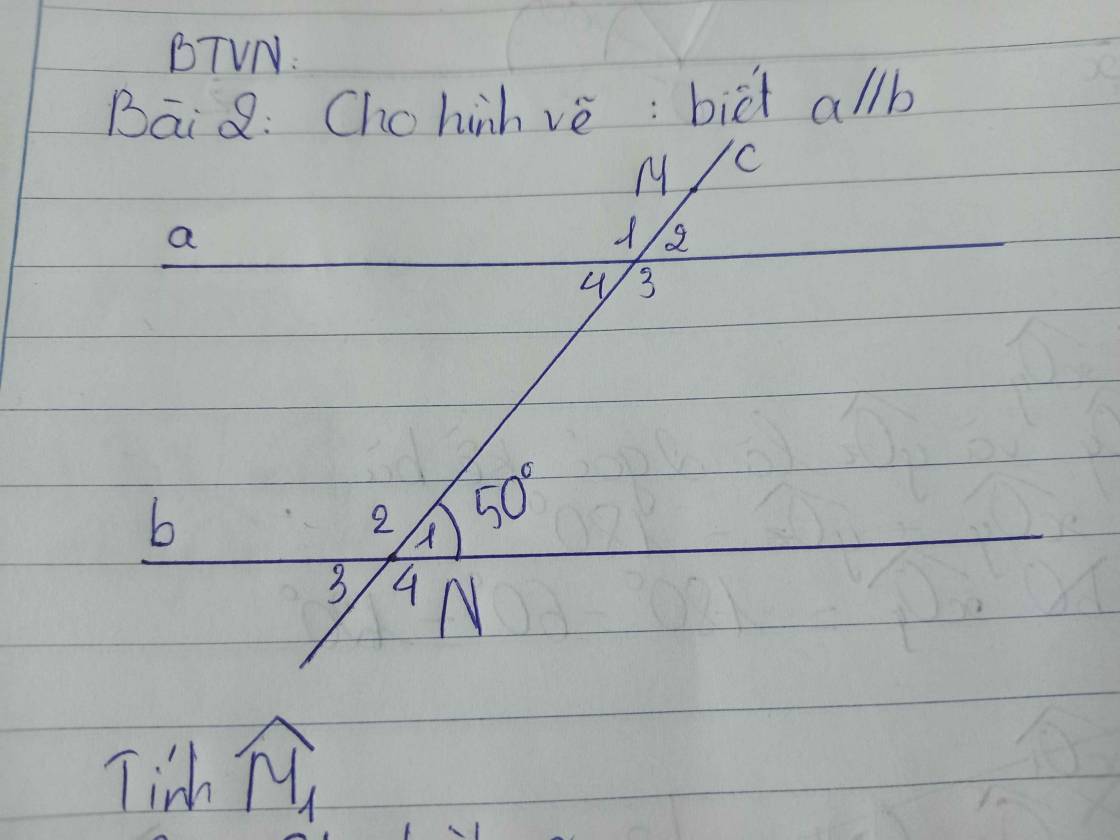
`a,`
`P(x)=x^2-5+x^4-4x^3-x^6`
`P(x)= -x^6+x^4-4x^3+x^2-5`
`Q(x)=2x^5-x^4+x^2-x^3+x-1`
`Q(x)=2x^5-x^4-x^3+x^2+x-1`
`b,`
`P(x)+Q(x)=(-x^6+x^4-4x^3+x^2-5)+(2x^5-x^4-x^3+x^2+x-1)`
`= -x^6+x^4-4x^3+x^2-5+2x^5-x^4-x^3+x^2+x-1`
`= -x^6+2x^5+(x^4-x^4)+(-4x^3-x^3)+(x^2+x^2)+x+(-5-1)`
`= -x^6+2x^5-5x^3+2x^2+x-6`
`c,`
`P(x)-Q(x)=(-x^6+x^4-4x^3+x^2-5)-(2x^5-x^4-x^3+x^2+x-1)`
`= -x^6+x^4-4x^3+x^2-5-2x^5+x^4+x^3-x^2-x+1`
`= -x^6-2x^5+(x^4+x^4)+(-4x^3+x^3)+(x^2-x^2)+x+(-5+1)`
`= -x^6-2x^5+2x^4-3x^3+x-4`
Ote.
Phần trừ đa thức một biến, bạn phải chú ý trước có dấu trừ, bạn ngoặc vào nhé! Còn trước dấu ngoặc có dấu trừ, đổi dấu. Khi gộp và rút gọn các đa thức cùng bậc, chú ý trước dấu ngoặc nên để dấu cộng, khi gộp vào phải đưa nguyên dấu của hạng tử, không được tự tiện đổi. Những cái này là phải nhớ nhé!