KZ
3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PT
1
CM
8 tháng 1 2017
Biểu thức x + 1 x 2 xác định khi x ≠ 0
Biểu thức x 2 + 1 x 2 + 2 x + 1 1 x + 1 xác định khi x ≠ 0 và x ≠ - 1
Với điều kiện x ≠ 0 và x ≠ - 1, ta có:
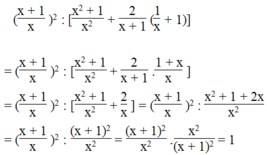
Vậy giá trị của biểu thức x + 1 x 2 : x 2 + 1 x 2 + 2 x + 1 1 x + 1 bằng 1 với mọi giá trị x ≠ 0 và x ≠ -1.
TV
1
30 tháng 10 2021
\(-x^2+8x-19=-\left(x^2-8x+16\right)-3=-\left(x-4\right)^2-3\le-3< 0\)
12 tháng 4 2018
Ta có :
\(x^2-4x+5=\left(x^2-2.2x+2^2\right)+1=\left(x-2\right)^2+1\ge1>0\)
Vậy đa thức \(x^2-4x+5\) vô nghiệm với mọi giá trị của x
Chúc bạn học tốt ~
7 tháng 10 2021
a: \(x^2-5x+10\)
\(=x^2-2\cdot x\cdot\dfrac{5}{2}+\dfrac{25}{4}+\dfrac{15}{4}\)
\(=\left(x-\dfrac{5}{2}\right)^2+\dfrac{15}{4}>0\forall x\)
b: \(2x^2+8x+15\)
\(=2\left(x^2+4x+\dfrac{15}{2}\right)\)
\(=2\left(x^2+4x+4+\dfrac{7}{2}\right)\)
\(=2\left(x+2\right)^2+7>0\forall x\)
NA
0
5 tháng 11 2021
a: \(A=x^3-27-x^3+3x^2-3x+1-4\left(x^2-4\right)-x\)
\(=3x^2-4x-26-4x^2+16\)
\(=-x^2-4x-10\)
NA
6 tháng 10 2018
a) Ta có \(2x^2-8x+13=2x^2-8x+8+5\)
\(=2\left(x^2-4x+4\right)+5\)
\(=2\left(x-2\right)^2+5\ge5\forall x\)
6 tháng 10 2018
Giả sử trước khi làm nhé
\(a)\)\(2x^2-8x+13>0\)
\(\Leftrightarrow\)\(4x^2-16x+26>0\)
\(\Leftrightarrow\)\(\left(4x^2-16+16\right)+10>0\)
\(\Leftrightarrow\)\(\left(2x-4\right)^2+10\ge10>0\) ( luôn đúng )
Vậy ...
\(b)\)\(-2+2x-x^2< 0\)
\(\Leftrightarrow\)\(x^2-2x+2>0\)
\(\Leftrightarrow\)\(\left(x^2-2x+1\right)+1>0\)
\(\Leftrightarrow\)\(\left(x-1\right)^2+1\ge1>0\) ( luôn đúng )
Vậy ...
Chúc bạn học tốt ~
CM
27 tháng 9 2018
a) Rút gọn E Þ đpcm.
b) Điều kiện xác định E là: x ≠ ± 1
Rút gọn F ta thu được F = 4 Þ đpcm
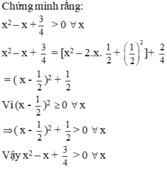
\(x-x^2-2\)
\(=x-\left(x^2+2\right)\)
Ta có : \(x^2\ge0\forall x\)
\(\Rightarrow x^2+2\ge0\)
mà \(x\le x^2\)
\(\Rightarrow x< x^2+2\)
\(\Rightarrow x-\left(x^2+2\right)< 0\)
\(\Rightarrow x-x^2-2< 0\)
Ta có: \(x-x^2-2=x-x^2-\frac{1}{4}-\frac{7}{4}\)
\(=\left(x-x^2-\frac{1}{4}\right)-\frac{7}{4}=-\left(x^2-x+\frac{1}{4}\right)-\frac{7}{4}\)
\(=-\left(x^2-2.\frac{1}{2}x+\frac{1}{4}\right)-\frac{7}{4}=-\left(x-\frac{1}{2}\right)^2-\frac{7}{4}\)
Vì \(\left(x-\frac{1}{2}\right)^2\ge0\forall x\)\(\Rightarrow-\left(x-\frac{1}{2}\right)^2\le0\forall x\)
\(\Rightarrow-\left(x-\frac{1}{2}\right)^2-\frac{7}{4}\le-\frac{7}{4}\)\(\forall x\)
\(\Rightarrow x-x^2-2\le-\frac{7}{4}\)\(\forall x\)
hay \(x-x^2-2< 0\)( đpcm )