K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên

5 tháng 7 2021
Vì \(\widehat{B}=120^0\) nên đường cao AH ứng với cạnh BC sẽ nằm ngoài tam giác ABC
Ta có: \(\widehat{ABH}+\widehat{ABC}=180^0\)(hai góc kề bù)
\(\Leftrightarrow\widehat{ABH}+120^0=180^0\)
hay \(\widehat{ABH}=60^0\)
Xét ΔABH vuông tại H có
\(\widehat{ABH}=60^0\)(cmt)
nên \(\sin\widehat{ABH}=\dfrac{\sqrt{3}}{2}\); \(\cos\widehat{ABH}=\dfrac{1}{2}\); \(\tan\widehat{ABH}=\sqrt{3}\); \(\cot\widehat{ABH}=\dfrac{\sqrt{3}}{3}\)
Xét ΔABH vuông tại H có
\(\widehat{BAH}=30^0\)
nên \(\sin\widehat{BAH}=\dfrac{1}{2}\); \(\cos\widehat{BAH}=\dfrac{\sqrt{3}}{2}\); \(\tan\widehat{BAH}=\dfrac{\sqrt{3}}{3}\); \(\cot\widehat{BAH}=\sqrt{3}\)
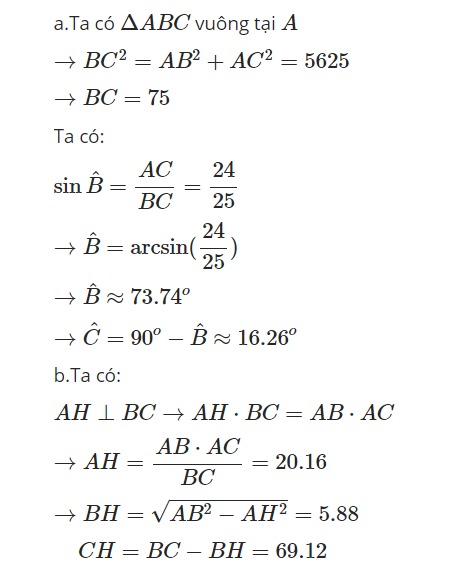
A B C H
\(sinABH=\frac{AH}{AB}\) \(cosABH=\frac{BH}{AB}\)
\(tanABH=\frac{AH}{BH}\) \(cotABH=\frac{BH}{AH}\)
\(sinHAB=\frac{BH}{AB}\) \(cosHAB=\frac{AH}{AB}\)
\(tanHAB=\frac{BH}{AH}\) \(cotHAB=\frac{AH}{BH}\)
\(\sin ABH=\frac{AH}{AB}\) \(\cos ABH=\frac{BH}{AB}\)
\(\tan ABH=\frac{AH}{BH}\) \(\cot ABH=\frac{BH}{AH}\)
\(\sin HAB=\frac{BH}{AB}\) \(\cos HAB=\frac{AH}{AB}\)
\(\tan HAB=\frac{BH}{AH}\) \(\cot HAB=\frac{AH}{BH}\)
Sorry ko vẽ đc hình
Code : Breacker