VN
và 
< a < π.
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PT
1
CM
8 tháng 7 2018
Vì π < a < 3 π 2 nên sina < 0; cosa < 0. Ta có
sin α - 2 cos α = 1 sin 2 α + cos 2 α = 1 ⇒ 1 + 2 cos α 2 + cos 2 α = 1 ⇒ 5 cos 2 α + 4 cos α = 0 ⇒ cos α = - 4 5
Suy ra α = - 1 - cos 2 α = - 3 5 ; tan α = 3 4 ; c o t α = 4 3 . Vậy A = 2tana - cota = 2 . 3 4 - 4 3 = 1 6
Đáp án B
CM
7 tháng 11 2019
Đáp án C
Phương pháp:
- Xác định góc giữa hai đường thẳng: Cho a, b là hai đường thẳng bất kì, đường thẳng a’ // a => (a;b) = (a’;b)
Cách giải:
Gọi O, M lần lượt là tâm của hình chữ nhật ABCD và trung điểm của SA
=> MO là đường trung bình của tam giác SAC
=>MO//SC
=>(BD,SC)=(BD,MO)
+) ABCD là hình chữ nhật
+) M là trung điểm SA
Tam giác MAB vuông tại A
Tam giác MAO vuông tại A
+) Xét tam giác MBO:
HT
25 tháng 12 2015
a) Do A + B + C = 180 độ nên góc A bù với góc B + C => sin(B + C) = sinA (sin hai góc bù bằng nhau)
(A + B)/2 + C/2 = 90 độ => hai góc (A + B)/2 và C/2 là hai góc phụ nhau => cos (A + B)/2 = sin(C/2) (Chắc đề bài bạn cho nhầm thành sinC)
b) Bạn xem lại đề nhé
c) \(sin^6a+cos^6a+3sin^2a.cos^2a=\left(sin^2a\right)^3+\left(cos^2a\right)^3+3.sin^2a.cos^2a\)
= \(\left(sin^2a+cos^2a\right)\left(sin^4a+cos^4a-sin^2a.cos^2a\right)+3sin^2a.cos^2a\)
= \(sin^4a+cos^4a+2sin^2a.cos^2a\)
= \(\left(sin^2a+cos^2a\right)^2=1\)
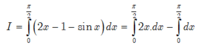
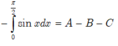
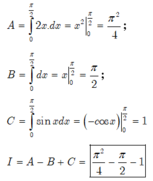
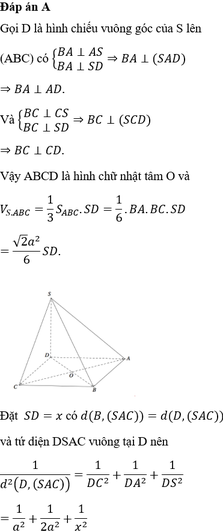
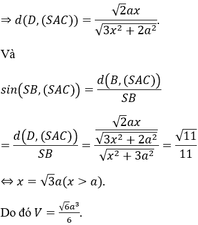
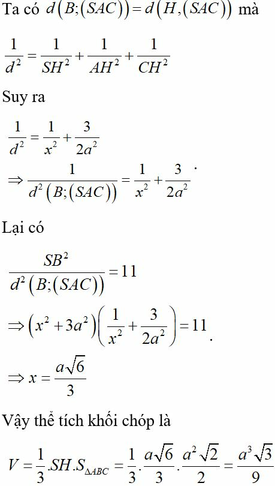
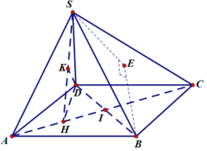
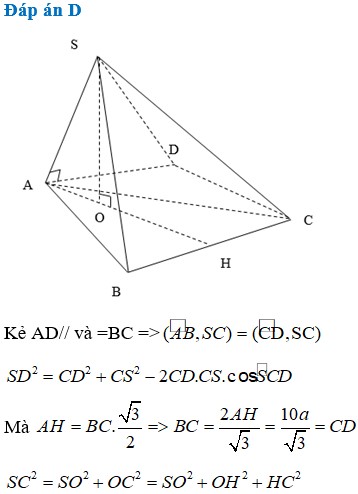
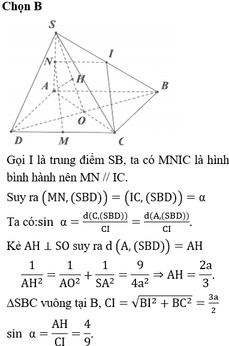
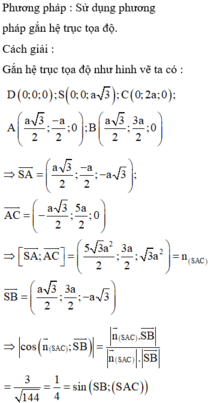
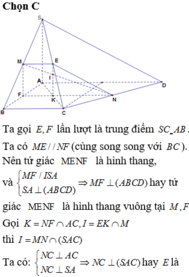
cos2a =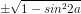
= ±
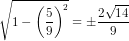
Nếu cos2a =
thì
sina =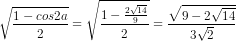
=
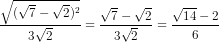
cosa = -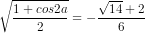
Nếu cos2a = -
thì
sina =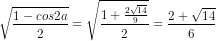
cosa = -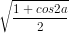
MMẫu giáo thật cơ ạ:))