Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

A B C D B' O
\(cos\left(\overrightarrow{AC};\overrightarrow{BA}\right)=cos\left(\overrightarrow{AC};\overrightarrow{AB'}\right)=cos\widehat{CAB'}=cos135^o\)\(=\dfrac{\sqrt{2}}{2}\).
\(sin\left(\overrightarrow{AC};\overrightarrow{BD}\right)=sin90^o=1\) do \(AC\perp BD\).
\(cos\left(\overrightarrow{AB};\overrightarrow{CD}\right)=cos180^o=-1\) do hai véc tơ \(\overrightarrow{AB};\overrightarrow{CD}\) ngược hướng.

\(a\text{) }\overrightarrow{AB}-\overrightarrow{CD}=\left(\overrightarrow{AC}+\overrightarrow{CB}\right)-\overrightarrow{CD}\\ =\overrightarrow{AC}-\left(\overrightarrow{CD}-\overrightarrow{CB}\right)=\overrightarrow{AC}-\overrightarrow{BD}\)
\(b\text{) }\overrightarrow{AB}+\overrightarrow{DC}+\overrightarrow{BD}+\overrightarrow{CA}=\left(\overrightarrow{AB}+\overrightarrow{BD}\right)+\left(\overrightarrow{DC}+\overrightarrow{CA}\right)\\ =\left(\overrightarrow{AB}+\overrightarrow{BD}\right)+\left(\overrightarrow{DC}+\overrightarrow{CA}\right)=\overrightarrow{AD}+\overrightarrow{DA}=0\)
\(c\text{) }\overrightarrow{AC}+\overrightarrow{DE}-\overrightarrow{DC}-\overrightarrow{CE}+\overrightarrow{CB}\\ =\left(\overrightarrow{AC}+\overrightarrow{CB}\right)+\left(\overrightarrow{DE}-\overrightarrow{DC}\right)-\overrightarrow{CE}\\ =\overrightarrow{AB}+\overrightarrow{CE}-\overrightarrow{CE}=\overrightarrow{AB}\)
\(d\text{) }\overrightarrow{AB}+\overrightarrow{DE}+\overrightarrow{CF}\\ =\left(\overrightarrow{AC}+\overrightarrow{CB}\right)+\left(\overrightarrow{DF}+\overrightarrow{FE}\right)+\left(\overrightarrow{CE}+\overrightarrow{EF}\right)\\ =\overrightarrow{AC}+\overrightarrow{CE}+\overrightarrow{CB}+\overrightarrow{DF}+\left(\overrightarrow{FE}+\overrightarrow{EF}\right)\\ =\overrightarrow{AC}+\overrightarrow{CE}+\overrightarrow{CB}+\overrightarrow{DF}\)

\(\overrightarrow{AC}-\overrightarrow{AD}=\overrightarrow{AC}-\overrightarrow{BC}=\overrightarrow{AC}+\overrightarrow{CB}=\overrightarrow{AB}\)
Đáp án A đúng

Tất cả biểu thức đều là vecto, cái nào là độ dài thì nằm trong trị tuyệt đối:
\(\left|BD\right|=\sqrt{AB^2+AD^2}=a\sqrt{5}\)
\(\left|AC\right|=\sqrt{AB^2+BC^2}=a\sqrt{13}\)
a/ \(AB.BD=-BA.BD=-\left|AB\right|.\left|BD\right|.cos\widehat{ABD}\)
\(=-2a.a\sqrt{5}.\frac{2a}{a\sqrt{5}}=-4a^2\)
\(BC.BD=\left|BC\right|.\left|BD\right|.cos\widehat{DBC}=3a.a\sqrt{5}.\frac{a}{a\sqrt{5}}=3a^2\)
\(AC.BD=AC\left(BA+AD\right)=AC.BA+AC.AD\)
\(=AC.AD-AC.AB=\left|AC\right|.\left|AD\right|.cos\widehat{DAC}-\left|AB\right|.\left|AC\right|.cos\widehat{BAC}\)
\(=a.a\sqrt{13}.\frac{3a}{a\sqrt{13}}-2a.a\sqrt{13}.\frac{2a}{a\sqrt{13}}=-a^2\)
\(AC.IJ=\frac{1}{2}AC\left(AD+BC\right)=\frac{1}{2}AC.AD+\frac{1}{2}AC.BC\)
Ta có \(AC.AD=3a^2\) (ngay bên trên)
\(AC.BC=CA.CB=\left|CA\right|.\left|CB\right|.cos\widehat{BCA}=a\sqrt{13}.3a.\frac{3a}{a\sqrt{13}}=9a^2\)
\(\Rightarrow AC.IJ=6a^2\)

\(\overrightarrow{AD}=2\overrightarrow{DB}\Rightarrow\overrightarrow{AD}=\dfrac{2}{3}\overrightarrow{AB}\) ; \(\overrightarrow{CE}=3\overrightarrow{EA}\Rightarrow\overrightarrow{AE}=\dfrac{1}{4}\overrightarrow{AC}\)
Lại có M là trung điểm DE
\(\Rightarrow\overrightarrow{AM}=\dfrac{1}{2}\left(\overrightarrow{AD}+\overrightarrow{AE}\right)=\dfrac{1}{2}\left(\dfrac{2}{3}\overrightarrow{AB}+\dfrac{1}{4}\overrightarrow{AC}\right)=\dfrac{1}{3}\overrightarrow{AB}+\dfrac{1}{8}\overrightarrow{AC}\)
I là trung điểm BC \(\Rightarrow\overrightarrow{AI}=\dfrac{1}{2}\left(\overrightarrow{AB}+\overrightarrow{AC}\right)\)
\(\Rightarrow\overrightarrow{MI}=\overrightarrow{MA}+\overrightarrow{AI}=\overrightarrow{AI}-\overrightarrow{AM}=\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{AC}-\dfrac{1}{3}\overrightarrow{AB}-\dfrac{1}{8}\overrightarrow{AC}=\dfrac{1}{6}\overrightarrow{AB}+\dfrac{3}{8}\overrightarrow{AC}\)
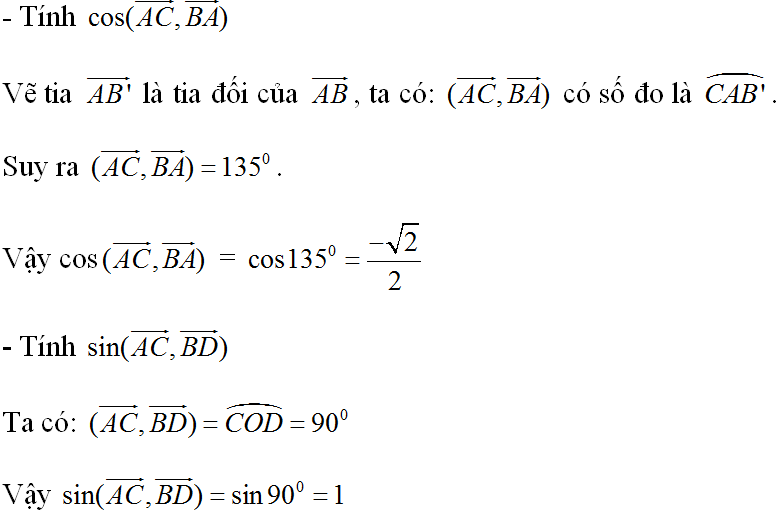
$ABCD$ là hình chữ nhật thì $AC=BD$ chứ bạn sao độ dài lại khác nhau được? Bạn xem lại đề.