Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(P=\left(\frac{\sqrt{x}\left(\sqrt{x}+1\right)}{\left(\sqrt{x}+1\right)\left(x+1\right)}+\frac{1}{x+1}\right).\frac{x+1}{\sqrt{x}-1}\)ĐK x>=0 x khác -1
=\(\frac{\sqrt{x}+1}{x+1}.\frac{x+1}{\sqrt{x}-1}=\frac{\sqrt{x}+1}{\sqrt{x}-1}\)
b/ x =\(\frac{2+\sqrt{3}}{2}=\frac{4+2\sqrt{3}}{4}=\frac{3+2\sqrt{3}+1}{4}=\frac{\left(\sqrt{3}+1\right)^2}{4}\)
\(\Rightarrow\sqrt{x}=\frac{\sqrt{3}+1}{2}\)
Em thay vào tính nhé!
c) với x>1
A=\(\frac{\sqrt{x}+1}{\sqrt{x}-1}.\sqrt{x}=\frac{x+\sqrt{x}}{\sqrt{x}-1}=\sqrt{x}+2+\frac{2}{\sqrt{x}-1}=\sqrt{x}-1+\frac{2}{\sqrt{x}-1}+3\)
Áp dụng bất đẳng thức Cosi
A\(\ge2\sqrt{2}+3\)
Xét dấu bằng xảy ra ....

a) điều kiện : \(a>0\)
ta có : \(P=\dfrac{a^2+\sqrt{a}}{a-\sqrt{a}+1}-\dfrac{2a+\sqrt{a}}{\sqrt{a}}+1\)
\(\Leftrightarrow P=\dfrac{\sqrt{a}\left(\sqrt{a}+1\right)\left(a-\sqrt{a}+1\right)}{a-\sqrt{a}+1}-\dfrac{2a+2\sqrt{a}}{\sqrt{a}}+1\)
\(\Leftrightarrow P=a+\sqrt{a}-2\sqrt{a}-2=a-\sqrt{a}-2\)
b) ta có : \(a>1\Rightarrow a>\sqrt{a}>1\Rightarrow a-\sqrt{a}>0\Rightarrow a-\sqrt{a}-2>-2\)
\(\Rightarrow\left|P\right|\ge P\) dấu "=" xảy ra khi \(a-\sqrt{a}>2\)
c) ta có : \(P=2\Leftrightarrow a-\sqrt{a}-2=2\Leftrightarrow a-\sqrt{a}-4=0\)
ta có : \(\Delta=1^2-4\left(-4\right)=17>0\) \(\Rightarrow\) phương trình có 2 nghiệm phân biệt
\(a=\dfrac{1+\sqrt{17}}{2};a=\dfrac{1-\sqrt{17}}{2}\)
vậy.................................................................................................................
d) ta có : \(P=a-\sqrt{a}-2\Leftrightarrow a-\sqrt{a}-2-P=0\)
ta có phương trình này luôn có nghiệm \(\Rightarrow\Delta\ge0\Leftrightarrow1^2-4\left(-2-P\right)\ge0\)
\(\Leftrightarrow4P+9\ge0\Leftrightarrow P\ge\dfrac{-9}{4}\)
\(\Rightarrow\) giá trị nhỏ nhất của \(P\) là \(\dfrac{-9}{4}\) ; dấu "=" xảy ra khi \(\sqrt{a}=\dfrac{-b}{2a}=\dfrac{1}{2}\Rightarrow x=\dfrac{1}{4}\)

1/ Rút gọn: \(a)3\sqrt{2a}-\sqrt{18a^3}+4\sqrt{\dfrac{a}{2}}-\dfrac{1}{4}\sqrt{128a}\left(a\ge0\right)=3\sqrt{2a}-3a\sqrt{2a}+2\sqrt{2a}-2\sqrt{2a}=3\sqrt{2a}\left(1-a\right)\)b)\(\dfrac{\sqrt{2}-1}{\sqrt{2}+2}-\dfrac{2}{2+\sqrt{2}}+\dfrac{\sqrt{2}+1}{\sqrt{2}}=\dfrac{\sqrt{2}-1-2}{\sqrt{2}+2}+\dfrac{\sqrt{2}+1}{\sqrt{2}}=\dfrac{\sqrt{2}-3}{\sqrt{2}+2}+\dfrac{\sqrt{2}+1}{\sqrt{2}}=\dfrac{\sqrt{2}-3+2+1+2\sqrt{2}}{\sqrt{2}\left(1+\sqrt{2}\right)}=\dfrac{3\sqrt{2}}{\sqrt{2}\left(1+\sqrt{2}\right)}=\dfrac{3}{1+\sqrt{2}}\)c)\(\dfrac{2+\sqrt{5}}{\sqrt{2}+\sqrt{3+\sqrt{5}}}+\dfrac{2-\sqrt{5}}{\sqrt{2}-\sqrt{3-\sqrt{5}}}=\dfrac{\sqrt{2}\left(2+\sqrt{5}\right)}{\left(\sqrt{2}+\sqrt{3+\sqrt{5}}\right)\sqrt{2}}+\dfrac{\sqrt{2}\left(2-\sqrt{5}\right)}{\sqrt{2}\left(\sqrt{2}-\sqrt{3-\sqrt{5}}\right)}=\dfrac{2\sqrt{2}+\sqrt{10}}{2+\sqrt{6+2\sqrt{5}}}+\dfrac{2\sqrt{2}-\sqrt{10}}{2-\sqrt{6-2\sqrt{5}}}=\dfrac{2\sqrt{2}+\sqrt{10}}{2+\sqrt{\left(\sqrt{5}+1\right)^2}}+\dfrac{2\sqrt{2}-\sqrt{10}}{2-\sqrt{\left(\sqrt{5}-1\right)^2}}=\dfrac{\sqrt{2}\left(2+\sqrt{5}\right)}{2+\sqrt{5}+1}+\dfrac{\sqrt{2}\left(2-\sqrt{5}\right)}{2-\sqrt{5}+1}=\dfrac{\sqrt{2}\left(2+\sqrt{5}\right)}{3+\sqrt{5}}+\dfrac{\sqrt{2}\left(2-\sqrt{5}\right)}{3-\sqrt{5}}=\dfrac{\sqrt{2}\left(2+\sqrt{5}\right)\left(3-\sqrt{5}\right)+\sqrt{2}\left(2-\sqrt{5}\right)\left(3+\sqrt{5}\right)}{\left(3+\sqrt{5}\right)\left(3-\sqrt{5}\right)}=\dfrac{\sqrt{2}\left(6-2\sqrt{5}+3\sqrt{5}-5+6+2\sqrt{5}-3\sqrt{5}-5\right)}{9-5}=\dfrac{2\sqrt{2}}{4}=\dfrac{1}{\sqrt{2}}\)
Làm nốt nè :3
\(2.a.P=\left(\dfrac{1}{x-\sqrt{x}}+\dfrac{1}{\sqrt{x}-1}\right):\dfrac{\sqrt{x}}{x-2\sqrt{x}+1}=\dfrac{\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}.\dfrac{\left(\sqrt{x}-1\right)^2}{\sqrt{x}}=\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}{x}=\dfrac{x-1}{x}\left(x>0;x\ne1\right)\)\(b.P>\dfrac{1}{2}\Leftrightarrow\dfrac{x-1}{x}-\dfrac{1}{2}>0\)
\(\Leftrightarrow\dfrac{x-2}{2x}>0\)
\(\Leftrightarrow x-2>0\left(do:x>0\right)\)
\(\Leftrightarrow x>2\)
\(3.a.A=\left(\dfrac{\sqrt{a}}{\sqrt{a}-1}-\dfrac{\sqrt{a}}{a-\sqrt{a}}\right):\dfrac{\sqrt{a}+1}{a-1}=\dfrac{\sqrt{a}-1}{\sqrt{a}-1}.\dfrac{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}{\sqrt{a}+1}=\sqrt{a}-1\left(a>0;a\ne1\right)\)
\(b.Để:A< 0\Leftrightarrow\sqrt{a}-1< 0\Leftrightarrow a< 1\)
Kết hợp với DKXĐ : \(0< a< 1\)

a) \(P=\frac{a^2+\sqrt{a}}{a-\sqrt{a}+1}-\frac{2a+\sqrt{a}}{\sqrt{a}}+1\)
\(=\frac{\sqrt{a}.\left[\left(\sqrt{a}\right)^3+1\right]}{a-\sqrt{a}+1}-\frac{\sqrt{a}.\left(2\sqrt{a}+1\right)}{\sqrt{a}}+1\)
\(=\frac{\sqrt{a}.\left(\sqrt{a}+1\right).\left(a-\sqrt{a}+1\right)}{a-\sqrt{a}+1}-2\sqrt{a}-1+1\)
\(=a+\sqrt{a}-2\sqrt{a}-1=a-\sqrt{a}\)
b)Ta có a>0 do đó: \(P=a-\sqrt{a}\ge0\)
Dấu "=" xảy ra khi a=1
c) Ta thấy \(P\ge0\)
=>P2\(\ge\)P
=>P\(\ge\)\(\sqrt{P}\)
ĐKXĐ: \(a>0\)
a/ \(P=\frac{\sqrt{a}\left(\sqrt{a}^3+1\right)}{a-\sqrt{a}+1}-\frac{\sqrt{a}\left(2\sqrt{a}+1\right)}{\sqrt{a}}+1\)
\(=\frac{\sqrt{a}\left(\sqrt{a}+1\right)\left(a-\sqrt{a}+1\right)}{a-\sqrt{a}+1}-\left(2\sqrt{a}+1\right)+1\)
\(=\sqrt{a}\left(\sqrt{a}+1\right)-2\sqrt{a}-1+1\)
\(=a-\sqrt{a}\)
b/ Ta có: \(\hept{\begin{cases}a>0\\\sqrt{a}\ge0\end{cases}\Rightarrow a-\sqrt{a}\ge0}\)
MinP = 0 khi \(\sqrt{a}=0\Rightarrow a=0\)
c/ \(P\ge\sqrt{P}\)

1: \(A=\dfrac{a+1-2\sqrt{a}}{a+1}:\dfrac{a+1-2\sqrt{a}}{\left(\sqrt{a}+1\right)\left(a+1\right)}\)
\(=\sqrt{a}+1\)
2: Khi \(a=2010-2\sqrt{2009}\) thì \(A=\sqrt{2009}-1+1=\sqrt{2009}\)

Bài 2:
a: \(P=\dfrac{a-1}{2\sqrt{a}}\cdot\left(\dfrac{\sqrt{a}\left(a-2\sqrt{a}+1\right)-\sqrt{a}\left(a+2\sqrt{a}+1\right)}{a-1}\right)\)
\(=\dfrac{a-2\sqrt{a}+1-a-2\sqrt{a}-1}{2}=-2\sqrt{a}\)
b: Để P>=-2 thì P+2>=0
\(\Leftrightarrow-2\sqrt{a}+2>=0\)
=>0<=a<1

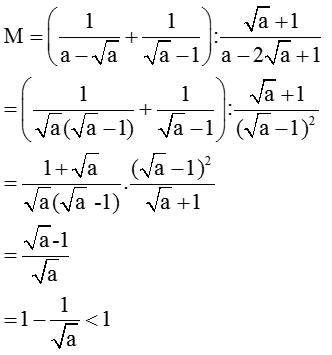
a) \(P=\dfrac{\sqrt{a}\left[\left(\sqrt{a}\right)^3+1\right]}{a-\sqrt{a}+1}-\dfrac{\sqrt{a}\left(2\sqrt{a}+1\right)}{\sqrt{a}}+1\)
\(P=\dfrac{\sqrt{a}\left(\sqrt{a}+1\right)\left(a-\sqrt{a}+1\right)}{a-\sqrt{a}+1}-\left(2\sqrt{a}+1\right)+1\)
\(P=\sqrt{a}\left(\sqrt{a}+1\right)-\left(2\sqrt{a}+1\right)+1\)
\(P=a+\sqrt{a}-2\sqrt{a}-1+1\)
\(P=a-\sqrt{a}\)
b) Với a > 1 thì \(a>\sqrt{a}\) , do đó \(P=a-\sqrt{a}>0\), suy ra \(\left|P\right|=P\)
c) \(A=a-\sqrt{a}=\left(\sqrt{a}-\dfrac{1}{2}\right)^2-\dfrac{1}{4}\ge-\dfrac{1}{4}\)
Vậy A nhỏ nhất bằng \(-\dfrac{1}{4}\) khi cà chỉ khi \(\sqrt{a}=\dfrac{1}{2}\) hay \(a=\dfrac{1}{4}\)
a: \(P=\sqrt{a}\left(\sqrt{a}+1\right)-2\sqrt{a}-1+1=a-\sqrt{a}\)
b: a>1 nên P>0
\(\Leftrightarrow P=\left|P\right|\)