
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


A = \(\dfrac{1}{2}+\dfrac{3}{2}+\dfrac{3}{2^2}+\dfrac{3}{2^3}+.....+\dfrac{3}{2^{2021}}+\dfrac{3}{2^{2022}}\)
\(2\times\)A = 1 + 3+ \(\dfrac{3}{2}\) +\(\dfrac{3}{2^2}\) + \(\dfrac{3}{2^3}\)+...........+\(\dfrac{3}{2^{2021}}\)
2 \(\times\) A - A = 4 - \(\dfrac{1}{2}\) - \(\dfrac{3}{2^{2022}}\)
A = \(\dfrac{7}{2}\) - \(\dfrac{3}{2^{2022}}\)
B = 2 \(\times\dfrac{3}{2^{2023}}\)
A - B = \(\dfrac{7}{2}-\dfrac{3}{2^{2022}}\) - 2 \(\times\) \(\dfrac{3}{2^{2023}}\)
A - B = \(\dfrac{7}{2}\) - \(\dfrac{3}{2^{2022}}\) - \(\dfrac{3}{2^{2022}}\)
A - B = \(\dfrac{7}{2}\) - \(\dfrac{6}{2^{2022}}\)
A - B = \(\dfrac{7}{2}\) - \(\dfrac{3}{2^{2021}}\)

Bạn nên viết lại đề bằng công thức toán (biểu tượng $\sum$ góc trái khung soạn thảo) để mọi người hiểu đề của bạn hơn nhé.

\(3B=1.3^2+2.3^3+3.3^4+...+2022.3^{2023}+2023.3^{2024}\)
\(2B=3B-B=-3-3^2-3^3-...-3^{2023}+2023.3^{2024}\)
\(2B=2023.3^{2024}-\left(3+3^2+3^3+...+3^{2023}\right)\)
Đặt
\(C=3+3^2+3^3+...+3^{2023}\)
\(3C=3^2+3^3+3^4+...+3^{2024}\)
\(2C=3C-C=3^{2024}-3\Rightarrow C=\dfrac{3^{2024}-3}{2}\)
\(\Rightarrow2B=2023.3^{2024}-\dfrac{3^{2024}-3}{2}=\)
\(=\dfrac{2.2023.3^{2024}-3^{2024}+3}{2}=\dfrac{4045.3^{2024}+3}{2}\)
\(\Rightarrow B=\dfrac{4045.3^{2024}+3}{4}\)

A=223+328+4215+...+2023220232−1
\(A = \frac{2^{2} - 1}{2^{2}} + \frac{3^{2} - 1}{3^{2}} + \frac{4^{2} - 1}{4^{2}} + . . . + \frac{202 3^{2} - 1}{202 3^{2}}\)
\(A = 1 - \frac{1}{2^{2}} + 1 - \frac{1}{3^{2}} + 1 - \frac{1}{4^{2}} + . . . + 1 - \frac{1}{202 3^{2}}\)
\(A = \left(\right. 1 + 1 + 1 + . . . + 1 \left.\right) - \left(\right. \frac{1}{2^{2}} + \frac{1}{3^{2}} + \frac{1}{4^{2}} + . . + \frac{1}{202 3^{2}} \left.\right)\)
Tổng số hạng của 2 ngoặc trên bằng nhau và =(2023-2):1+1=2022(số hạng)
\(A = 2022 - \left(\right. \frac{1}{2^{2}} + \frac{1}{3^{2}} + \frac{1}{4^{2}} + . . . + \frac{1}{202 3^{2}} \left.\right)\)
Ta thấy:
\(0 < \frac{1}{2^{2}} + \frac{1}{3^{2}} + \frac{1}{4^{2}} + . . . + \frac{1}{202 3^{2}} < \frac{1}{1.2} + \frac{1}{2.3} + \frac{1}{3.4} + . . + \frac{1}{2022.2023}\)
Ta có
\(\frac{1}{1.2} + \frac{1}{2.3} + \frac{1}{3.4} + . . + \frac{1}{2022.2023}\)
\(= 1 - \frac{1}{2} + \frac{1}{2} - \frac{1}{3} + \frac{1}{3} - \frac{1}{4} + . . + \frac{1}{2022} - \frac{1}{2023}\)
\(= 1 - \frac{1}{2023} < 1\)
Do đó,2021<A<2022
Vậy giá trị của A không phải 1 số tự nhiên(đpcm)

`#3107.101107`
1.
`a,`
\(A=1+3+3^2+3^3+...+3^{2012}\)
`3A = 3 + 3^2 + 3^3 + ... + 3^2013`
`3A - A = (3 + 3^2 + 3^3 + ... + 3^2013) - (1 + 3 + 3^2 + 3^3 + ... + 3^2012)`
`2A = 3 + 3^2 + 3^3 + ... + 3^2013 - 1 - 3 - 3^2 - 3^3 - ... - 3^2012`
`2A = 3^2013 - 1`
`=> A = (3^2013 - 1)/2`
Vậy, `A = (3^2013 - 1)/2`
`b,`
\(B=1+10+10^2+10^3+...+10^{2023}\)
`10B = 10 + 10^2 + 10^3 + ... + 10^2024`
`10 B - B = (10 + 10^2 + 10^3 + ... + 10^2024) - (1 - 10 + 10^2 + 10^3 + ... + 10^2023)`
`9B = 10 + 10^2 + 10^3 + ... + 10^2024 - 1 - 10^2 - 10^3 - ... - 10^2023`
`9B = 10^2024 - 1`
`=> B = (10^2024 - 1)/9`
Vậy, `B = (10^2024 - 1)/9.`
`a)A=1+3+3^2+3^3+...+3^2012`
`=>3A=3+3^2+3^3+...+3^2013`
`=>3A-A=2A=3^2013-1`
`=>A=(3^2013-1)/2`
`b)B=1+10+10^2+...+10^2024`
`=>10B=10+10^2+10^3+....+10^2025`
`=>10B-B=9B=10^2025-10`
`=>B=(10^2025-10)/9`
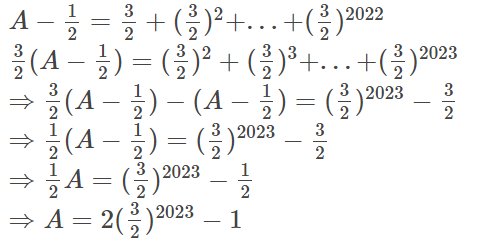
3A=3+3^2+...+3^2024
=>2A=3^2024-1
=>B-2A=3^2023-3^2024+1