Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
\(\left[\left(x+1\right).\left(x+4\right)\right].\left[\left(x+2\right).\left(x+3\right)\right]-24\)
\(=\left(x^2+5x+4\right).\left(x^2+5x+6\right)-24\)
Đặt m=x2+5x+4, ta có:
\(m.\left(m+2\right)-24=m^2+2m-24=m^2+6m-4m-24\)
\(=m.\left(m+6\right)-4.\left(m+6\right)=\left(m-4\right).\left(m+6\right)\)
Tự làm tiếp :v
\(1.a\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)-24\)
\(=\left(x^2+5x+4\right)\left(x^2+5x+6\right)-24\)
\(=\left(x^2+5x+5-1\right)\left(x^2+5x+5+1\right)-24\)
\(=\left(x^2+5x+5\right)^2-1-24\)
\(=\left(x^2+5x+5\right)^2-25\)
\(=\left(x^2+5x+5+5\right)\left(x^2+5x+5-5\right)\)
\(=\left(x^2+5x+10\right)\left(x^2+5x\right)\)
\(=x\left(x+5\right)\left(x^2+5x+10\right)\)
\(b.x^4+4=x^4+4x^2+4-4x^2=\left(x^2+2\right)^2-4x^2=\left(x^2+2x+2\right)\left(x^2-2x+2\right)\)
\(2.a\) Đặt \(a=\frac{x+3}{x-2},b=\frac{x-3}{x+2}\)
Thay vào PT ta được:\(a^2+6b^2=7ab\)
\(\Leftrightarrow a^2-7ab+6b^2=0\)
\(\Leftrightarrow a^2-ab-6ab+6b^2=0\)
\(\Leftrightarrow a\left(a-b\right)-6b\left(a-b\right)=0\)
\(\Leftrightarrow\left(a-b\right)\left(a-6b\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}a-b=0\\a-6b=0\end{cases}\Leftrightarrow\orbr{\begin{cases}a=b\\a=6b\end{cases}\Leftrightarrow}\orbr{\begin{cases}\frac{x+3}{x-2}=\frac{x-3}{x+2}\\\frac{x+3}{x-2}=6.\frac{x-3}{x+2}\end{cases}\Leftrightarrow}\orbr{\begin{cases}\left(x+3\right)\left(x+2\right)=\left(x-3\right)\left(x-2\right)\\\left(x+3\right)\left(x+2\right)=\left(6x-18\right)\left(x-2\right)\end{cases}}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=0\\x=1hayx=6\end{cases}}\) (bước kia dài bạn tự làm nhé)
Câu 1:
\(Tacó\)
\(\frac{2}{2x-1}+\frac{4x^2+1}{4x^2-1}-\frac{1}{2x+1}=\frac{2}{2x-1}+\frac{4x^2+1}{\left(2x+1\right)\left(2x-1\right)}-\frac{1}{2x+1}\)
\(=\frac{4x+2}{\left(2x+1\right)\left(2x-1\right)}+\frac{4x^2+1}{\left(2x+1\right)\left(2x-1\right)}-\frac{2x-1}{\left(2x+1\right)\left(2x-1\right)}\)
\(=\frac{4x+2+4x^2+1-2x+1}{\left(2x+1\right)\left(2x-1\right)}=\frac{2x\left(2x+1\right)+4}{\left(2x+1\right)\left(2x-1\right)}=\frac{2x+4}{2x-1}\)
\(b,x=\frac{1}{2}\Rightarrow2x-1=0\left(loại\right)\)
..... 2 câu sau easy
Bài 2: \(a,\frac{7x-1}{2x^2+6x}=\frac{7x-1}{2x\left(x+3\right)}=\frac{\left(7x-1\right)\left(x-3\right)}{2x\left(x+3\right)\left(x-3\right)}\)
\(\frac{5-3x}{x^2-9}=\frac{5-3x}{\left(x-3\right)\left(x+3\right)}=\frac{\left(5-3x\right)2x}{2x\left(x-3\right)\left(x+3\right)}\)
\(b,\frac{x+1}{x-x^2}=\frac{x+1}{x\left(1-x\right)}=-\frac{x+1}{x\left(x+1\right)}=-\frac{2\left(x-1\right)\left(x+1\right)}{2x\left(x-1\right)^2}\)
\(\frac{x+2}{2-4x+2x^2}=\frac{x+2}{2\left(x-1\right)^2}=\frac{2x\left(x+2\right)}{2x\left(x-1\right)^2}\)
\(c,\frac{4x^2-3x+5}{x^3-1}=\frac{4x^2-3x+5}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\frac{2x}{x^2+x+1}=\frac{2x\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\frac{6}{x-1}=\frac{6\left(x^2+x+1\right)}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(d,\frac{7}{5x}=\frac{7.2\left(2y-x\right)\left(2y+x\right)}{2.5x\left(2y-x\right)\left(2y+x\right)}\)
\(\frac{4}{x-2y}=-\frac{4}{2y-x}=-\frac{4.2.5x\left(2x+x\right)}{2.5x\left(2y-x\right)\left(2y+x\right)}\)
\(\frac{x-y}{8y^2-2x^2}=\frac{x-y}{2\left(4y^2-x^2\right)}=\frac{x-y}{2\left(2y-x\right)\left(2y+x\right)}=\frac{5x\left(x-y\right)}{2.5x.\left(2y-x\right)\left(2y+x\right)}\)
áp dụng bđt cauchy-shwarz dạng engel
\(\text{ Σ}_{cyc}\frac{a^2}{b+c}\ge\frac{\left(a+b+c\right)^2}{2\left(a+b+c\right)}\)\(=\frac{a+b+c}{2}\)
Ta có hđt \(\text{ Σ}_{cyc}a^3-3abc=\left(a+b+c\right)\left(a^2+b^2+c^2-ab-bc-ca\right)\)
Mà a+b+c khác 0 nên a = b = c
\(\Rightarrow N=1\)
câu a,b thì mình làm được còn câu c,d thì mình chưa làm ra. Chân thành xin lỗi
a) có \(\widehat{BDC}=45^0\)(ABCD là hình vuông, BD là đường chéo)
\(\widehat{DKN}\left(hay\widehat{DKH}\right)=45^0\)(CHIK là hình vuông và KH là đường chéo)
\(\Rightarrow\widehat{BDC}+\widehat{DKN}=45^0+45^0=90^0\)
\(\Rightarrow\Delta DKN\)vuông tại N
\(\Rightarrow KN\perp DN\)
mà \(BC\perp DK\)
KN và BC cắt nhau tại H
suy ra H là trực tâm của tam giác BDK
nên \(DH\perp BK\)
b) Xét \(\Delta DMB\&\Delta KNB\)
có \(\widehat{DMB}=\widehat{KNB}\)=900
\(\widehat{DBK}chung\)
\(\Rightarrow\Delta DMB\)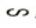
\(\Delta KNB\)(g-g)
\(\Rightarrow\frac{MB}{NB}=\frac{BD}{BK}\)
từ tỉ số trên ta đễ chứng minh \(\Delta BMN\)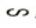\(\Delta BDK\)
cm tương tự ta có \(\Delta CMK\)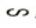\(\Delta BDK\)
\(\Rightarrow\Delta BMN\)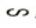\(\Delta CMK\)
\(\Rightarrow\widehat{BMN}=\widehat{CMK}\)
lại có \(\hept{\begin{cases}\widehat{BMN}+\widehat{DMN}=90^0\\\widehat{CMK}+\widehat{DMC}=90^0\end{cases}}\)(\(DM\perp BK\))
\(\Rightarrow\widehat{DMN}=\widehat{DMC}\)
nên MD là phân giác của \(\widehat{NMC}\)
Bài 2
A/ \(x^2-2xy+y^2-4x+4y-5\)
\(=\left(x^2-2xy+y^2\right)-\left(4x-4y\right)-5\)
\(=\left(x-y\right)^2-4\left(x-y\right)-5\)
\(=\left(x-y\right)\left(x-y-4\right)-5\)
b/ trên máy tính đâu có đặt cột dọc được :v chịu khó tính nháp là ra xD
Bài 3
1/a \(\left(x^2-4x\right)^2+2\left(x-2\right)^2=4^3.\)
\(\left(x^2-4x\right)^2+2\left(x^2-4x+4\right)=64\)
Cho \(x^2-4x\) là S
\(\Rightarrow S^2+2\left(S+4\right)=64\)
\(\Rightarrow S^2+2S+8=64\)
\(\Rightarrow S^2+2S=64-8\)
\(\Rightarrow S^2+2S=56\)
Tính ko ra:v đề có sai ko?
2/ \(2x^2+3y^2+4x=19\)
\(\Rightarrow2x^2+4x=19-3y^2\)
\(\Rightarrow2x^2+4x=21-2-3y^2\)
\(\Rightarrow2x^2+4x+2=21-3y^2\)
\(\Rightarrow2\left(x^2+2x+1\right)=21-3y^2\)
\(\Rightarrow2\left(x+1\right)^2=21-3y^2\)
\(\Rightarrow2\left(x+1\right)^2=3\left(7-y^2\right)\)
Từ đây xét tiếp để ra kq :v