Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: Để hai đường song song thì 3m^2+1=4m và m^2-9<>-m-5
=>(m-1)(3m-1)=0 và m^2+m-4<>0
=>m=1 hoặc m=1/3
b: Để hai đường cắt nhau thì 3m^2+1<>4m
=>m<>1 và m<>1/3
Khi m=2 thì (d1): \(y=8x-7\)
(d2): y=13x-5
Toa độ giao điểm là:
8x-7=13x-5 và y=8x-7
=>-5x=-5+7=2 và y=8x-7
=>x=-2/5 và y=-16/5-7=-16/5-35/5=-51/5

a: Để hai đường song song thì
\(\left\{{}\begin{matrix}2m^2-m=1\\m^2+m< >2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left(m-1\right)\left(2m+1\right)=0\\\left(m+2\right)\left(m-1\right)< >0\end{matrix}\right.\Leftrightarrow m=-\dfrac{1}{2}\)
b: Thay x=2 vào (d1), ta đc:
\(y=2+2=4\)
Vì (d3) vuông góc với (d1) nên (d3): y=-x+b
Thay x=2 và y=4 vào (d3), ta được:
b-2=4
=>b=6

Phương trình đường thẳng (d) luôn có dạng :
\(y=ax+b\left(d\right)\)
a/ Ta có : \(\left(d\right)\) đi qua hai điểm \(A\left(2,7\right);B\left(-1;-2\right)\)
\(\Leftrightarrow\left\{{}\begin{matrix}7=2a+b\\-2=-a+b\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a=3\\b=1\end{matrix}\right.\)
Vậy...
b/ Ta có : \(\left(d\right)\backslash\backslash\left(d_1\right)\)
\(\Leftrightarrow\left\{{}\begin{matrix}a=-2\\b\ne-6\end{matrix}\right.\)
\(\Leftrightarrow a=-2\)
Phương trình hoành độ giao điểm của \(\left(d_2\right);\left(d_3\right)\) là :
\(2x+1=-x+4\)
\(\Leftrightarrow3x=3\)
\(\Leftrightarrow x=1\)
\(\Leftrightarrow y=3\)
Tọa độ giao điểm của \(\left(d_2\right);\left(d_3\right)\) là \(H\left(1;3\right)\)
Lại có : \(\left(d\right)\) đi qua \(H\left(1;3\right)\)
\(\Leftrightarrow3=a+b\)
\(\Leftrightarrow b=5\)
Vậy....
c/ Ta có : \(\left(d\right)\) đi qua \(C\left(-2;1\right)\)
\(\Leftrightarrow-2=a+b\)
Lại có : \(\left(d\right)\perp\left(d_4\right)\)
\(\Leftrightarrow a.\frac{-1}{2}=1\)
\(\Leftrightarrow a=-2\)
\(\Leftrightarrow b=0\)
Vậy...

a, Hoành độ giao điểm (P) ; (d) thỏa mãn pt
\(x^2=2x-m\Leftrightarrow x^2-2x+m=0\)
Để pt có 2 nghiệm pb khi \(\Delta'=1-m>0\Leftrightarrow m< 1\)
Vậy với m < 1 thì (P) cắt (d) tại 2 điểm pb
b, Theo Vi et \(\hept{\begin{cases}x_1+x_2=2\\x_1x_2=m\end{cases}}\)
Ta có : \(\frac{1}{x_1^2}+\frac{1}{x_2^2}=2\Leftrightarrow\frac{x_1^2+x_2^2}{x_1^2x_2^2}=2\)
\(\Leftrightarrow\frac{\left(x_1+x_2\right)^2-2x_1x_2}{\left(x_1x_2\right)^2}=2\)Thay vào ta có :
\(\Leftrightarrow\frac{4-2m}{m^2}=2\Leftrightarrow4-2m=2m^2\Leftrightarrow2m^2+2m-4=0\)
mà a + b + c = 0 => 2 + 2 - 4 = 0
vậy pt có 2 nghiệm
\(m_1=1\left(ktm\right);m_2=-2\left(tm\right)\)

a: Tọa độ A là:
\(\left\{{}\begin{matrix}-x+5=\dfrac{1}{4}x\\y=\dfrac{1}{4}x\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-\dfrac{5}{4}x=-5\\y=\dfrac{1}{4}x\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=4\\y=1\end{matrix}\right.\)
Tọa độ B là:
-x+5=4x và y=4x
=>-5x=-5 và y=4x
=>B(1;4)
Tọa độ C là:
1/4x=4x và y=4x
=>C(0;0)
b: A(4;1); B(1;4); O(0;0)
\(OA=\sqrt{4^2+1^2}=\sqrt{17}\)
\(OB=\sqrt{4^2+1^2}=\sqrt{17}\)
=>OA=OB
=>ΔOAB cân tại O

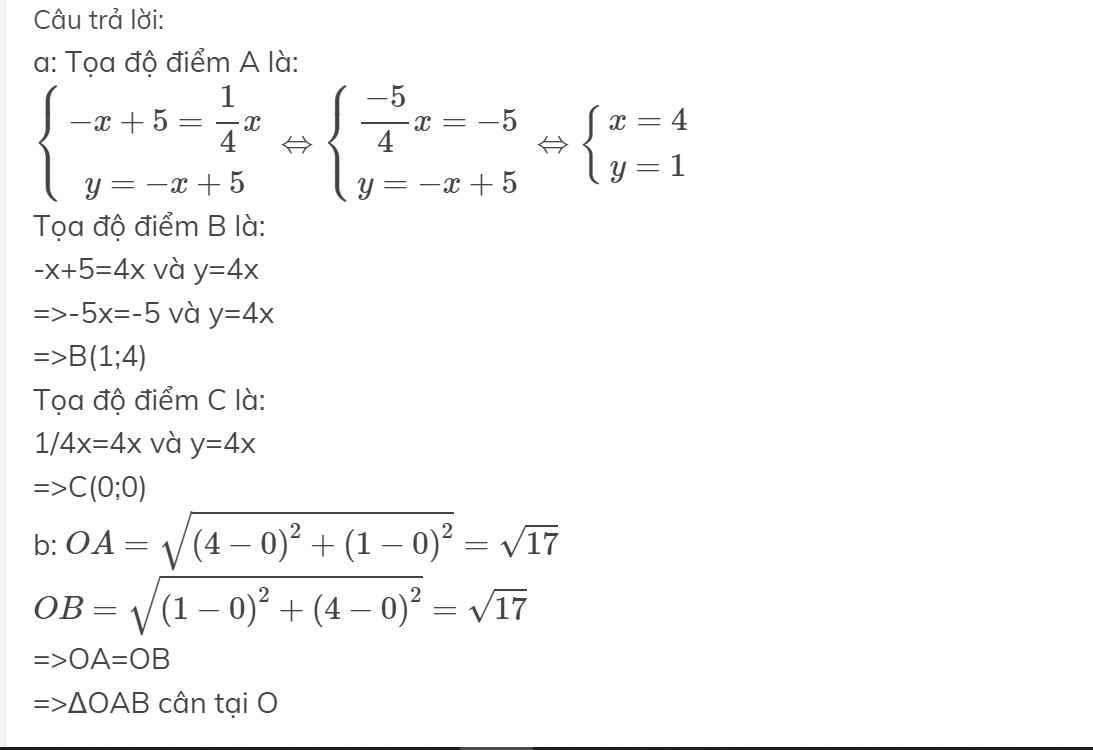
a: Tọa độ điểm A là:
\(\left\{{}\begin{matrix}-x+5=\dfrac{1}{4}x\\y=-x+5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{-5}{4}x=-5\\y=-x+5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=4\\y=1\end{matrix}\right.\)
Tọa độ điểm B là:
-x+5=4x và y=4x
=>-5x=-5 và y=4x
=>B(1;4)
Tọa độ điểm C là:
1/4x=4x và y=4x
=>C(0;0)
b: \(OA=\sqrt{\left(4-0\right)^2+\left(1-0\right)^2}=\sqrt{17}\)
\(OB=\sqrt{\left(1-0\right)^2+\left(4-0\right)^2}=\sqrt{17}\)
=>OA=OB
=>ΔOAB cân tại O

OMG!! Cái đề bài dài như Vạn Lý Trường Thành thế kia! Đau mắt quá! :D
a/ Gọi pt (d) có dạng: y= ax+b (\(a\ne0\) )
Có (d)//(d1)\(\Rightarrow\left\{{}\begin{matrix}a=2\\b\ne-5\end{matrix}\right.\)
Vì \(M\left(1;5\right)\in\left(d\right)\)
Thay xM= 1; yM= 5 vào (d) có:
\(2.1+b=5\Leftrightarrow b=3\) (t/m)
Vậy (d) y= 2x+3
b/ (d2) y= x+1
Vì (d)\(\perp\left(d_3\right)\)
\(\Rightarrow a.\frac{1}{2}=-1\Leftrightarrow a=-2\)
Vì (d) cắt (d2) tại điểm có tung độ =3
\(\Rightarrow\) Thay y=3 vào (d2) có:
x+1= 3=>x= 2
Thay y= 3, x= 2 vào (d)
\(-2.2+b=3\Leftrightarrow b=7\)
Vậy (d) y= -2x+7
c/ Vì (d) đi qua gốc toạ độ=> (d) y=ax
Xét PTHĐGĐ (d4) và (d5):
\(2x+4=-x-5\Leftrightarrow x=-3\Rightarrow y=-2\)
Thay x= -3; y= -2 vào (d)
-3a= -2
\(a=\frac{2}{3}\)
Vậy (d) y= \(\frac{2}{3}x\)
d/ Vì (d) vuông góc ....
\(\Rightarrow a.\frac{1}{3}=-1\Rightarrow a=-3\)
Vì A(3;-1) \(\in\left(d\right)\)
thay xA= 3; yA= -1 vào (d)
\(-3.3+b=-1\Leftrightarrow b=8\)
Vậy (d) y= -3x+8
e/ Vì (d) cắt trục hoành....
\(\Rightarrow y=0;x=-1\)
Thay vào (d)
-a+b= 0(1)
Có N(-2;3)\(\in\left(d\right)\)
Thay xN= -2;yN= 3 vào (d)
-2a+b= 3(2)
Từ (1) và (2) ta có hpt:
\(\left\{{}\begin{matrix}b-a=0\\b-2a=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}b=-3\\a=-3\end{matrix}\right.\)
Vậy (d)y= -3a-3



a: Để (d)//(d1) thì \(\left\{{}\begin{matrix}m^2-2=2\\m-1\ne-3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}m^2=4\\m\ne-2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}m\in\left\{2;-2\right\}\\m\ne-2\end{matrix}\right.\)
=>m=2
b: Để (d) trùng với (d2) thì
\(\left\{{}\begin{matrix}m^2-2=-1\\m-1=-2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}m^2=1\\m=-1\end{matrix}\right.\)
=>m=-1
c:
Để (d) cắt (d3) thì \(m^2-2\ne3\)
=>\(m^2\ne5\)
=>\(m\ne\pm\sqrt{5}\)
Thay x=-1 vào y=3x-2, ta được:
\(y=3\left(-1\right)-2=-5\)
Thay x=-1 và y=-5 vào (d), ta được:
\(-\left(m^2-2\right)+m-1=-5\)
=>\(-m^2+2+m-1+5=0\)
=>\(-m^2+m+6=0\)
=>\(m^2-m-6=0\)
=>(m-3)(m+2)=0
=>\(\left[{}\begin{matrix}m-3=0\\m+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}m=3\left(nhận\right)\\m=-2\left(nhận\right)\end{matrix}\right.\)
d: Để (d) vuông góc với (d4) thì \(\dfrac{4}{5}\left(m^2-2\right)=-1\)
=>\(m^2-2=-1:\dfrac{4}{5}=-\dfrac{5}{4}\)
=>\(m^2=\dfrac{3}{4}\)
=>\(m=\pm\dfrac{\sqrt{3}}{2}\)