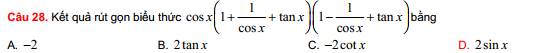LT
0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
8 tháng 11 2023
Vì \(90^o< x< 180^o\Rightarrow\cos x< 0\)
Có: \(\sin^2x+\cos^2x=1\Leftrightarrow\left(\dfrac{2}{5}\right)^2+\cos^2x=1\Leftrightarrow\cos^2x=\dfrac{21}{25}\Leftrightarrow\cos x=-\dfrac{\sqrt{21}}{5}\left(vì\cos x< 0\right)\)
2 tháng 7 2023
1:
-x^2+x+6<=0
=>x^2-x-6>=0
=>(x-3)(x+2)>=0
=>x>=3 hoặc x<=-2
2:
Phương trình tổng quát của (d) là:
x=1+t và y=-3+4t
NT
3
KV
6 tháng 10 2023
cosx(1 + 1/cosx + tanx)(1 - 1/cosx + tanx)
= cosx[(1 + tanx)² - 1/cos²x]
= cosx(1 + tan²x + 2tanx - 1 - tan²x)
= 2tanxcosx
= 2sinxcosx/cosx
= 2sinx
Chọn D
6 tháng 10 2023
\(=cosx\left(1+\dfrac{1}{cosx}+\dfrac{sinx}{cosx}\right)\left(1-\dfrac{1}{cosx}+\dfrac{sinx}{cosx}\right)\)
\(=cosx\left(\dfrac{sinx+1+cosx}{cosx}\right)\left(\dfrac{cosx+sinx-1}{cosx}\right)\)
\(=\left(cosx+sinx\right)^2\)\(-1\)
\(=cos^2x+2cosxsinx+sin^2x-1\)
\(=cos^2x+sin^2x+2cosxsinx-1\)
\(=1+2cosxsinx-1\)
\(=2cosxsinx\)
Áp dụng đẳng thức góc nhân đôi cho sin
\(=2sinx\)
21 tháng 8 2021
Hiểu như này:
\(\dfrac{a}{1+a}+\dfrac{b}{1+b}+\dfrac{b}{1+b}=3-\left(\dfrac{1}{1+a}+\dfrac{1}{1+b}+\dfrac{1}{1+b}\right)\le3-\dfrac{9}{1+a+1+b+1+b}=\dfrac{3\left(a+2b\right)}{3+a+2b}\)
NV
Nguyễn Việt Lâm
Giáo viên
26 tháng 3 2021
\(\Leftrightarrow x^2-2x-m+\dfrac{2\left(x^2-2x-m\right)\left(\sqrt{x}+1\right)}{x+\sqrt{2x+m}}=0\)
\(\Leftrightarrow\left(x^2-2x-m\right)\left(1+\dfrac{2\left(\sqrt{x}+1\right)}{x+\sqrt{2x+m}}\right)=0\)
\(\Leftrightarrow x^2-2x-m=0\)