K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
18 tháng 3 2020
\(\left(x-\frac{1}{2}\right)^2=0\)
\(\Leftrightarrow\left(x-\frac{1}{2}\right)^2=0^2\)
\(\Leftrightarrow x-\frac{1}{2}=0\)
\(\Leftrightarrow x=\frac{1}{2}\)
Vậy x = 1/2
\(\left(x-2\right)^2=1\)
\(\Leftrightarrow\left(x-2\right)^2=1^2\)
\(\Leftrightarrow x-2=1\)
\(\Leftrightarrow\orbr{\begin{cases}x-2=1\\x-2=-1\end{cases}\Leftrightarrow\orbr{\begin{cases}x=3\\x=1\end{cases}}}\)
Vậy x = 3 hoặc x = 1
\(\left(2x-1\right)^3=-8\)
\(\Leftrightarrow\left(2x-1\right)^3=\left(-2\right)^3\)
\(\Leftrightarrow2x-1=-2\)
<=> 2x = -1
<=> x = -0,5
Vậy x = -0,5
18 tháng 3 2020
\(\left(x-\frac{1}{2}\right)^2=0\)
\(x-\frac{1}{2}=0\)
\(x=\frac{1}{2}\)
\(\left(x-2\right)^2=1\)
\(\Leftrightarrow\orbr{\begin{cases}x-2=1\\x-2=-1\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=1+2\\x=-1+2\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=3\\x=1\end{cases}}\)
Vậy\(x\in\left\{3;1\right\}\)
\(\left(2x-1\right)^3=-8\)
\(\left(2x-1\right)^3=\left(-2\right)^3\)
\(2x-1=-2\)
\(2x=\left(-2\right)+1\)
\(2x=-1\)
\(x=-1\times2\)
\(x=-2\)
\(x\left(\frac{1}{2}\right)^2=\frac{1}{16}\)
\(x\left(\frac{1}{2}\right)^2=\left(\frac{1}{4}\right)^2\)
\(\Leftrightarrow\orbr{\begin{cases}x\frac{1}{2}=\frac{1}{4}\\x\frac{1}{2}=-\frac{1}{4}\end{cases}\Leftrightarrow\orbr{\begin{cases}x=\frac{1}{4}:\frac{1}{2}\\x=-\frac{1}{4}:\frac{1}{2}\end{cases}\Leftrightarrow}\orbr{\begin{cases}x=\frac{1}{2}\\x=-\frac{1}{2}\end{cases}}}\)
23 tháng 3 2017
M+N=(3/2x6-7x+4x^5+2,5x^2)+(-3x^6+1/2^5-13/2x^2+4x)
M+N=3/2x6-7x+4x^5+2,5x^2+-3x^6+1/2^5-13/2x^2+4x
= (3/2x^6-3x^6)+(7x+4x)+(4x^5+1/2^5)+(2,5x^2-13/2x^2)
=-1,5x^6+11x+4,5x^5-4x^2
M-N=(3/2^6-7x+4x^5+2,5x^2)-(-3x^6+1/2^5-13/2x^2+4x)
=3/2^6-7x+4x^5+2,5x^2+3x^6-1/2^5+13/2x^2-4x
= (3/2x^6+3x^6)+(-7x-4x)+(4x^5-1/2^5)+(2,5x^2+13/2x^2)
= 4,5x^6-11x+3,5x^5+9x^2
N-M=(-3x^6+1/2^5-13/2x^2+4x)-(3/2^6-7x+4x^5+2,5x^2)
= -3x^6+1/2^5-13/2x^2+4x-3/2^6-7x-4x^5-2,5x^2
= (-3x^6-3/2x^6)+(1/2x^5-4x^5)+(-13/2x^2-2,5x^2)+(4x-7x)
= -4,5x^6-3,5x^5-9x^2-3x
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
26 tháng 3
a,(\(6x-5x^2-15+2x^3:\left(2x-5\right)\)
\(\left(2x^3-5x^2+6x-15\right):\left(2x-5\right)\)
2 tháng 10 2016
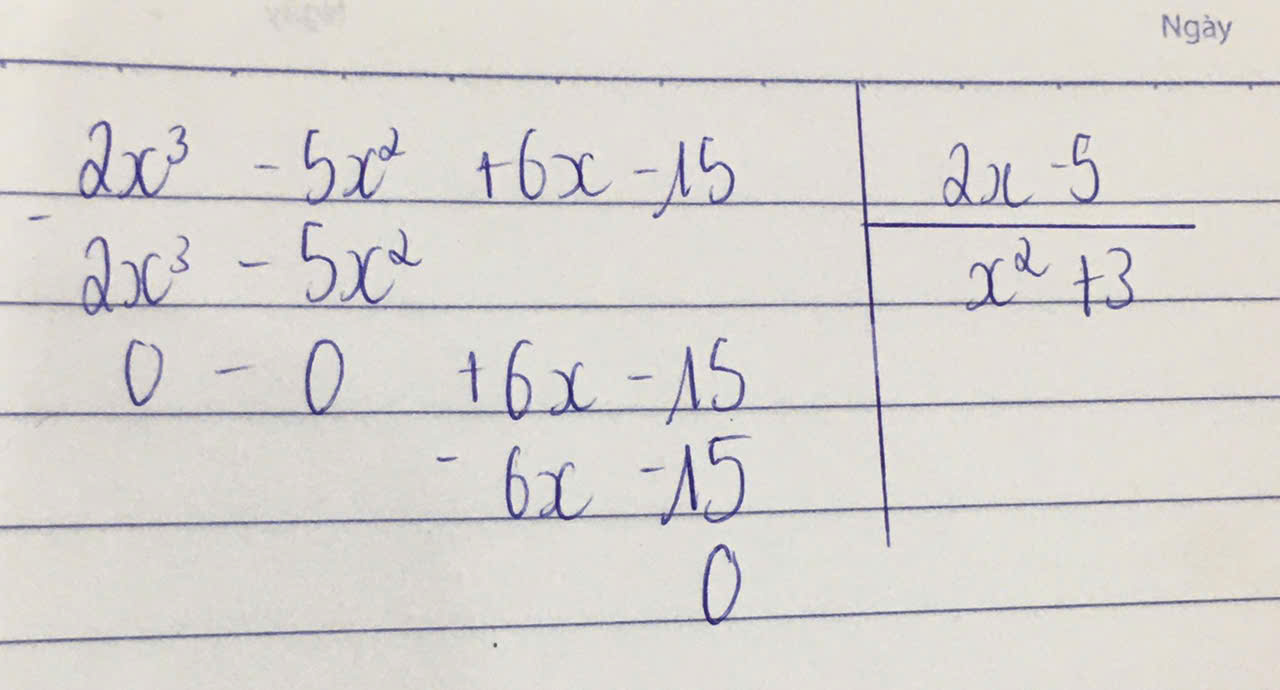
a. x = {3;-3}
b. x thuộc rỗng
c. x2-4=0
x2 = 4
x={2;-2}
d. x2+1=82
x2 =83
x thuộc rỗng
e. (2x)2=6
x thuộc rỗng
f. (x-1)2=9
TH1: x-1=3=>x=4
TH2: x-1=-3=>x=-2
Vậy x={4;-2}
g.(2x+3)2=25
TH1: 2x+3=5=> x=1
Th2: 2x+3=-5=>x=-4
VẬY X={1;-4}
2 tháng 10 2016
a, x^2= 9
=>\(\sqrt{9}=3\)
b,\(x^2=5=>x=\sqrt{5}\)
c, x^2-4=0
=>x^2=4
=>x=2
d, x^2+1=82
=>x^2=81 =>\(\sqrt{81}=9\)
3, 2x^2=6
=>x= \(\sqrt{6}\)
f, {x-1} ^2=9
=> x-1=3
=>x=2
g{ 2x+3}^2=25
=> 2x+3=5
=>2x=2
=>x=1
AM
12 tháng 7 2018
\(4)D=x^2+x+1\)
\(D=x^2+2x.\frac{1}{2}+\left(\frac{1}{2}\right)^2-\left(\frac{1}{2}\right)^2+1\)
\(D=\left(x+\frac{1}{2}\right)^2-\frac{1}{4}+1\)
\(D=\left(x+\frac{1}{2}\right)^2+\frac{3}{4}\)
Vậy biểu thức trên luôn nhận giá trị dương với mọi giá trị của x.
Các câu khác lm tương tự nhé.
Cho góp ý xíu: lần sau bn đưa từng câu một lên diễn đàn thì sẽ có câu trả lời nhanh hơn là đưa cùng một lúc như thế này đấy
hok tốt~
3 tháng 8 2020
\(D=x^2+x+1=x^2+x+\frac{1}{4}+\frac{3}{4}=\left(x+\frac{1}{2}\right)^2+\frac{3}{4}\)
\(\left(x+\frac{1}{2}\right)^2\ge0\forall x\Rightarrow\left(x+\frac{1}{2}\right)^2+\frac{3}{4}\ge\frac{3}{4}>0\forall x\)( đpcm )
\(F=2x^2+4x+3=2\left(x^2+2x+1\right)+1=2\left(x+1\right)^2+1\)
\(2\left(x+1\right)^2\ge0\forall x\Rightarrow2\left(x+1\right)^2+1\ge1>0\forall x\)( đpcm )
\(G=3x^2-5x+3=3\left(x^2-\frac{5}{3}x+\frac{25}{36}\right)+\frac{11}{12}=3\left(x-\frac{5}{6}\right)^2+\frac{11}{12}\)
\(3\left(x-\frac{5}{6}\right)^2\ge0\forall x\Rightarrow3\left(x-\frac{5}{6}\right)^2+\frac{11}{12}\ge\frac{11}{12}>0\forall x\)( đpcm )
\(H=4x^2+4x+2=4\left(x^2+x+\frac{1}{4}\right)+1=4\left(x+\frac{1}{2}\right)^2+1\)
\(4\left(x+\frac{1}{2}\right)^2\ge0\forall x\Rightarrow4\left(x+\frac{1}{2}\right)^2+1\ge1>0\forall x\)( đpcm )
\(K=4x^2+3x+2=4\left(x^2+\frac{3}{4}x+\frac{9}{64}\right)+\frac{23}{16}=4\left(x+\frac{3}{8}\right)^2+\frac{23}{16}\)
\(4\left(x+\frac{3}{8}\right)^2\ge0\forall x\Rightarrow4\left(x+\frac{3}{8}\right)^2+\frac{23}{16}\ge\frac{23}{16}>0\forall x\)( đpcm )
\(L=2x^2+3x+4=2\left(x^2+\frac{3}{2}x+\frac{9}{16}\right)+\frac{23}{8}=2\left(x+\frac{3}{4}\right)^2+\frac{23}{8}\)
\(2\left(x+\frac{3}{4}\right)^2\ge0\forall x\Rightarrow2\left(x+\frac{3}{4}\right)^2+\frac{23}{8}\ge\frac{23}{8}>0\forall x\)( đpcm )
\(\left(2x-1\right)^6=\left(2x-1\right)^8\\ \Rightarrow\left(2x-1\right)^6-\left(2x-1\right)^8=0\\ \Rightarrow\left(2x-1\right)^6\left[1-\left(2x-1\right)^2\right]=0\\ \Rightarrow\left[{}\begin{matrix}2x-1=0\\\left(2x-1\right)^2=1\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\2x-1=1\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=1\end{matrix}\right.\)
\(\left(2x-1\right)^6=\left(2x-1\right)^8\)
\(\Rightarrow\left[{}\begin{matrix}2x-1=-1\\2x-1=1\\2x-1=0\end{matrix}\right.\)\(\Rightarrow\left[{}\begin{matrix}x=0\\x=1\\x=\dfrac{1}{2}\end{matrix}\right.\)