K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 6 2021
\(1\le1+\sqrt{1-x^2}\le2\Rightarrow3\le3^{1+\sqrt{1-x^2}}\le9\)
Đặt \(3^{1+\sqrt{1-x^2}}=t\Rightarrow t\in\left[3;9\right]\)
Phương trình trở thành: \(t^2-\left(m+2\right)t+2m+1=0\)
\(\Leftrightarrow t^2-2t+1=m\left(t-2\right)\Leftrightarrow m=\dfrac{t^2-2t+1}{t-2}\)
Xét hàm \(f\left(t\right)=\dfrac{t^2-2t+1}{t-2}\) trên \(\left[3;9\right]\)
\(f'\left(t\right)=\dfrac{t^2-4t+3}{\left(t-2\right)^2}\ge0\) ; \(\forall t\in\left[3;9\right]\Rightarrow f\left(t\right)\) đồng biến trên khoảng đã cho
\(\Rightarrow f\left(3\right)\le f\left(t\right)\le f\left(9\right)\Rightarrow4\le m\le\dfrac{64}{7}\)
Có 6 giá trị nguyên của m
20 tháng 6 2021
Cho e hỏi tại sao điều kiện lại nằm trong khoảng [1,2] vậy ạ ?
QB
1
LV
1
HT
29 tháng 9 2016
Đk: x khác 0;
pt \(\Leftrightarrow-9\cdot2^{\frac{1}{x}}-5\cdot2^{\frac{1}{x}}\cdot3^{\frac{1}{x}}+4\cdot3^{\frac{2}{x}}=0\)
\(\Leftrightarrow4\cdot\left(3^{\frac{1}{x}}\right)^2-5\cdot2^{\frac{1}{x}}\cdot3^{\frac{1}{x}}-9\cdot2^{\frac{1}{x}}=0\)
xem pt trên là pt bậc hai ẩn 31/x, ta có: \(\Delta=\left(5\cdot2^{\frac{1}{x}}\right)^2-4\cdot4\cdot\left(-9\cdot2^{\frac{2}{x}}\right)=169\cdot2^{\frac{1}{x}}\)
\(3^{\frac{1}{x}}=\frac{5\cdot2^{\frac{1}{x}}-13\cdot2^{\frac{1}{x}}}{2\cdot4}=-2^{\frac{1}{x}}\) (loại)
\(3^{\frac{1}{x}}=\frac{5\cdot2^{\frac{1}{x}}+13\cdot2^{\frac{1}{x}}}{2\cdot4}=\frac{9}{4}\cdot2^{\frac{1}{x}}\Leftrightarrow3^{\frac{1}{x}-2}=2^{\frac{1}{x}-2}\Leftrightarrow\frac{1}{x}-2=0\Leftrightarrow x=\frac{1}{2}\)
TL
6
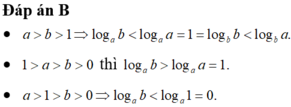
1×1÷1÷1÷1÷1÷1×1×1×1×1×1×0×9= 0
ht
= 0 nha bạn