
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Các câu hỏi dưới đây có thể giống với câu hỏi trên


29 tháng 6 2017
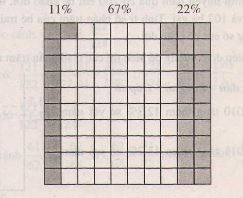
Ta có: tổng = xi măng + cát + sỏi = 1 + 2 + 6 = 9
- Tỉ lệ phần trăm của xi măng = (xi măng . 100 / tổng) % = \(\left( {1.{{100} \over 9}} \right)\% = 11,11\% \)
- Tỉ lệ phần trăm của cát = (cát . 100 / tổng) % = \(\left( {2.{{100} \over 9}} \right)\% = 22,22\% \)
- Tỉ lệ phần trăm của sỏi = (sỏi . 100 / tổng) % = \(\left( {6.{{100} \over 9}} \right)\% = 66,67\% \)
b) Biểu đồ ô vuông:


CG
5

24 tháng 8 2017
285
= 28 . 28 . 28 . 28 . 28
= 784 . 784 . 28
= 614656 . 28
= 17210368

QS
9
























 nữa nè
nữa nè  nữa nè
nữa nè nữa nè
nữa nè  hi hi thấy sao
hi hi thấy sao ha ha các chế tháy sao
ha ha các chế tháy sao thêm nữa nè
thêm nữa nè__25343_std.jpg) nữa nè hi hi
nữa nè hi hi nữa nè
nữa nè 

In my school, there are many teachers.I love all the teachers who taught me but the one i loved the most was teaching English while studying in elemantary school. her name is Tham , Miss Tham is about 29 years old and has about 1m64cm ,she has long hair on her back, long black hair and smooth. Facial oval eyes with large double eyelid clear. While teaching you if you have a fever, she will take you to the medical center immediately. She is sociable and happy with everyone whether her friends and students . She loves us all and we love her very much.
Everyone sat up expectantly. It was a maths period and all of us waited eagerly for our maths teacher, Mrs Huang, to come into the class. She is very popular among us. In fact, she is my favorite teacher.
Mrs Huang has short curly hair and wears thick glasses, which makes her look stern. However, she is actually very approachable and is always ready to lend an ear to our problems. She is motherly and cares very much for us. I remember once when I fell and hurt my knee. Mrs Huang helped me to stop the bleeding and took me to the clinic. I was very grateful to her.
I used to dis maths when I was younger because I found it far too complicated. Mrs Huang always makes maths lessons interesting and easy to understand. She is very patient in explaining maths problems to us and always makes sure everyone understands. Under her guidance, my maths has improved by leaps and bounds.
I have grown to love maths and I always score high marks in my maths tests now. It is all because of my favorite teacher.