K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
H9
HT.Phong (9A5)
CTVHS
23 tháng 1 2024
Áp dụng định lý Py-ta-go cho tam giác ABC vuông tại A ta có:
\(BC^2=AB^2+AC^2\)
\(\Rightarrow BC=\sqrt{AB^2+AC^2}\)
\(\Rightarrow BC=\sqrt{3^2+4^2}=5\left(cm\right)\)
Ta có:
\(\dfrac{AB}{DE}=\dfrac{3}{15}=\dfrac{1}{5}\)
\(\dfrac{AC}{DF}=\dfrac{4}{20}=\dfrac{1}{5}\)
\(\dfrac{BC}{EF}=\dfrac{5}{25}=\dfrac{1}{5}\)
\(\Rightarrow\dfrac{AB}{DE}=\dfrac{AC}{DF}=\dfrac{BC}{EF}=\dfrac{1}{5}\)
Xét hai tam giác ABC và DEF có:
\(\dfrac{AB}{DE}=\dfrac{AC}{DF}=\dfrac{BC}{EF}\left(=\dfrac{1}{5}\right)\)
\(\Rightarrow\Delta ABC\sim\Delta DEF\left(c.c.c\right)\)
H9
HT.Phong (9A5)
CTVHS
23 tháng 1 2024
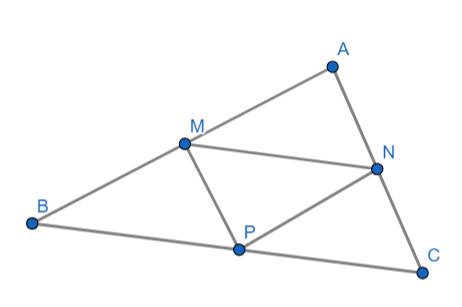
a) Ta có:
M là trung điểm của AB
N là trung điểm của AC
⇒ MN là đường trung bình của tam giác ABC
⇒ MN // BC
\(\Rightarrow\widehat{AMN}=\widehat{ABC}\) (đồng vị)
Xét hai tam giác ABC và AMN có:
\(\widehat{AMN}=\widehat{ABC}\left(cmt\right)\)
\(\widehat{BAC}\) chung
\(\Rightarrow\Delta ABC\sim\Delta AMN\left(g.g\right)\)
b) Chứng minh tương tự như câu a thì ta có:
PN cũng là đường trung bình của tam giác ABC \(\Rightarrow PN=\dfrac{1}{2}AB\)
PM cũng là đường trung bình của tam giác ABC \(\Rightarrow PM=\dfrac{1}{2}AC\)
Mà: \(NM=\dfrac{1}{2}BC\) (NM là đường trung bình ...)
Xét hai tam giác ABC và PNM có:
\(\dfrac{PN}{AB}=\dfrac{PM}{AC}=\dfrac{NM}{BC}=\dfrac{1}{2}\)
\(\Rightarrow\Delta ABC\sim\Delta PNM\left(c.c.c\right)\)
H9
HT.Phong (9A5)
CTVHS
23 tháng 1 2024
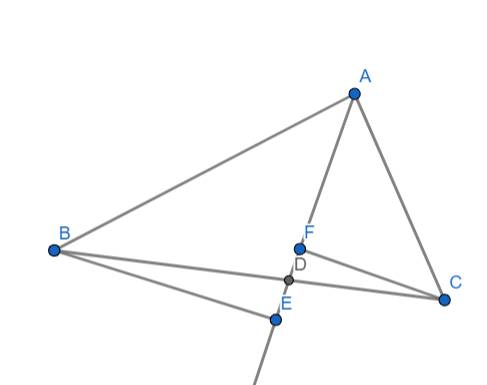
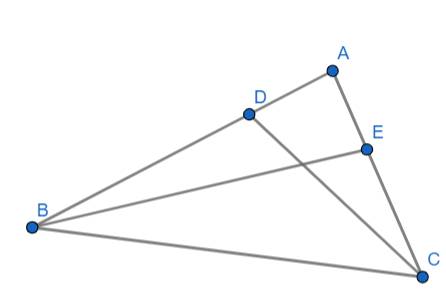
a) Ta có: E,F lần lược là hình chiếu của B,C trên AD
\(\Rightarrow\left\{{}\begin{matrix}\widehat{BEA}=90^o\\\widehat{CFA}=90^o\end{matrix}\right.\)
Xét hai tam giác ABE và ACF có:
\(\widehat{BEA}=\widehat{CFA}\left(=90^o\right)\)
\(\widehat{BAE}=\widehat{CAF}\) (do AD là phân giác của góc A)
\(\Rightarrow\Delta ABE\sim\Delta ACF\left(g.g\right)\)
b) Xét hai tam giác BDE và CDF có:
\(\widehat{BDE}=\widehat{CDF}\) (đối đỉnh)
\(\widehat{BED}=\widehat{CFD}\left(=90^o\right)\)
\(\Rightarrow\Delta BDE\sim\Delta CDF\left(g.g\right)\)
\(\Rightarrow\dfrac{BE}{CF}=\dfrac{DE}{DF}\) (1)
Mà: \(\Delta ABE\sim\Delta ACF\left(cmt\right)\)
\(\Rightarrow\dfrac{BE}{CF}=\dfrac{AE}{AF}\left(2\right)\)
Từ (1) và (2) ta có: \(\dfrac{DE}{DF}=\dfrac{AE}{AF}\Rightarrow AF\cdot DE=AE\cdot DF\)
H9
HT.Phong (9A5)
CTVHS
16 tháng 1 2024
a) Đường thẳng \(y=ax+b\) song song với đường thẳng \(y=4x-3\)
Nên có \(a=4\) đường thẳng có dạng \(y=3x+b\left(b\ne-3\right)\)
Mà \(y=3x+b\) đi qua điểm \(I\left(\dfrac{1}{2};\dfrac{3}{4}\right)\) nên ta thay \(x=\dfrac{1}{2};y=\dfrac{3}{4}\) ta có:
\(\dfrac{3}{4}=3\cdot\dfrac{1}{2}+b\)
\(\Leftrightarrow\dfrac{3}{4}=\dfrac{3}{2}+b\)
\(\Leftrightarrow b=\dfrac{3}{4}-\dfrac{3}{2}\)
\(\Leftrightarrow b=-\dfrac{3}{4}\left(tm\right)\)
Vậy: \(y=4x-\dfrac{3}{4}\)
b) Đường thẳng \(y=ax+b\) có hệ số góc \(a=3\) nên có dạng \(y=3x+b\)
Do đường thẳng cắt trục tung tại điểm có tung độ là - 4 nên ta thay \(x=0;y=-4\)
\(-4=0\cdot3+b\)
\(\Leftrightarrow b=-4\)
Vậy: \(y=3x-4\)
H9
HT.Phong (9A5)
CTVHS
16 tháng 1 2024
\(y=\left(m-3\right)+m^2\) có: \(\left\{{}\begin{matrix}a=m-3\\b=m^2\end{matrix}\right.\)
a) Để \(y=\left(m-3\right)x+m^2\) cắt \(y=3x+5\) thì:
\(a\ne a'\) hay:
\(m-3\ne3\)
\(\Leftrightarrow m\ne3+3\)
\(\Leftrightarrow m\ne6\)
b) Để \(y=\left(m-3\right)x+m^2\) song song với \(y=-2x+1\) thì
\(\left\{{}\begin{matrix}a=a'\\b\ne b'\end{matrix}\right.\) hay:
\(\left\{{}\begin{matrix}m-3=-2\\m^2\ne1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m=-2+3\\m\ne\pm1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m=1\\m\ne\pm1\end{matrix}\right.\) (ktm)
Vậy không có m thỏa mãn
c) Để \(y=\left(m-3\right)x+m^2\) trùng với \(y=-x+4\) thì
\(\left\{{}\begin{matrix}a=a'\\b=b'\end{matrix}\right.\) hay:
\(\left\{{}\begin{matrix}m-3=-1\\m^2=4\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m=-1+3\\m=\pm2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m=2\\m=\pm2\end{matrix}\right.\Leftrightarrow m=2\)
H9
HT.Phong (9A5)
CTVHS
16 tháng 1 2024
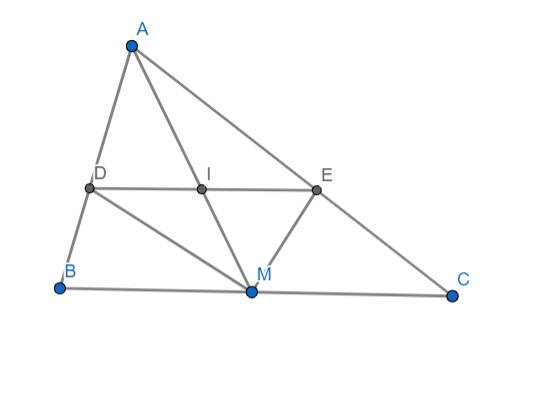
a) Ta có ME là tia phân giác của góc AMC nên:
\(\dfrac{AM}{AE}=\dfrac{MC}{CE}\Rightarrow\dfrac{AM}{MC}=\dfrac{AE}{CE}\) (1)
MD là tia phân giác của góc AMB nên:
\(\dfrac{AM}{AD}=\dfrac{BM}{BD}\Rightarrow\dfrac{AM}{BM}=\dfrac{AM}{CM}=\dfrac{AD}{BD}\) (vì M là trung điểm của BC nên BM = CM) (2)
Từ (1) và (2) ta có: \(\dfrac{AE}{CE}=\dfrac{AD}{BD}\Rightarrow DE//BC\)
b) Ta có: \(\Delta ADE\sim\Delta ABC\left(g.g\right)\) (vì có DE//BC)
\(\Rightarrow\dfrac{DE}{BC}=\dfrac{AE}{AC}\) (3)
\(\Delta AIE\sim\Delta AMC\left(g.g\right)\) (vì có IE//MC)
\(\Rightarrow\dfrac{IE}{MC}=\dfrac{AE}{AC}\) (4)
Từ (3) và (4) ta có: \(\dfrac{DE}{BC}=\dfrac{IE}{MC}\Rightarrow\dfrac{DE}{IE}=\dfrac{BC}{MC}=2\)
\(\Rightarrow DE=2IE\)
Hay I là trung điểm của DE
H9
HT.Phong (9A5)
CTVHS
23 tháng 1 2024
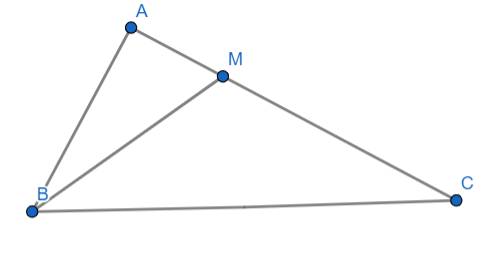
a) Áp dụng định lý Py-ta-go cho tam giác ABC vuông tại A ta có:
\(BC^2=AB^2+AC^2\)
\(\Rightarrow BC=\sqrt{AB^2+AC^2}\)
\(\Rightarrow BC=\sqrt{10^2+20^2}=10\sqrt{5}\left(cm\right)\)
Áp dụng định lý Py-ta-go cho tam giác ABM vuông tại A ta có:
\(BM^2=AB^2+AM^2\)
\(\Rightarrow BM=\sqrt{AB^2+AM^2}\)
\(\Rightarrow BM=\sqrt{10^2+5^2}=5\sqrt{5}\left(cm\right)\)
b) Ta có:
\(\dfrac{AM}{AB}=\dfrac{1}{2}\)
\(\dfrac{BM}{BC}=\dfrac{1}{2}\)
\(\Rightarrow\dfrac{AM}{AB}=\dfrac{MB}{BC}=\dfrac{1}{2}\)
Xét hai tam giác ABC và AMB có:
\(\widehat{BAC}\) chung
\(\dfrac{AM}{AB}=\dfrac{MB}{BC}=\dfrac{1}{2}\)
\(\Rightarrow\Delta ABC\sim\Delta AMB\left(c.g.c\right)\)
H9
HT.Phong (9A5)
CTVHS
23 tháng 1 2024
a) Xét hai tam giác ABE và ACD có:
\(\widehat{ACD}=\widehat{ABE}\left(gt\right)\)
\(\widehat{BAC}\) chung
\(\Rightarrow\Delta ABE\sim\Delta ACD\left(g.g\right)\)
b) Ta có: \(\Delta ABE\sim\Delta ACD\left(cmt\right)\)
\(\Rightarrow\dfrac{AB}{AC}=\dfrac{AE}{AD}\)
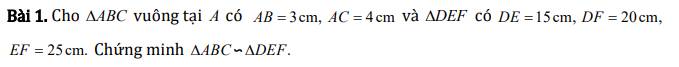
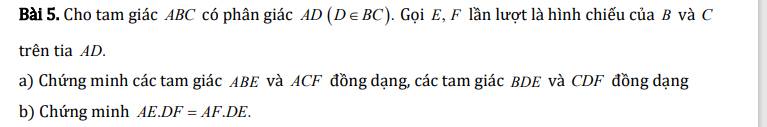
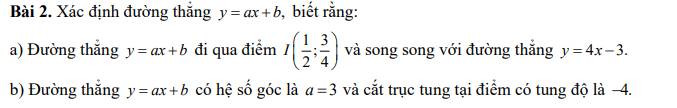
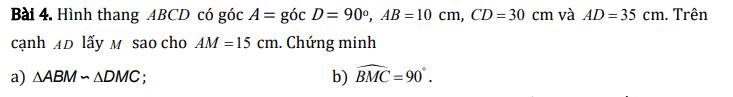
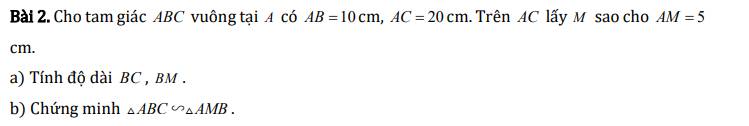
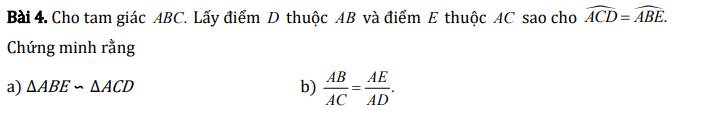
`#3107.101107`
`a)`
`5 - (x - 6) = 4(2x - 3)`
\(\Leftrightarrow5-x+6=8x-12\)
\(\Leftrightarrow-x-8x=-12-5-6\)
\(\Leftrightarrow-9x=-23\)
\(\Leftrightarrow x=\dfrac{-23}{-9}=\dfrac{23}{9}\)
Vậy, \(x=\dfrac{23}{9}\)
`b)`
\(5\left(3x+2\right)-4\left(5-3x\right)=1\)
\(\Leftrightarrow15x+10-20+12x=1\)
\(\Leftrightarrow27x-10=1\)
\(\Leftrightarrow27x=11\)
\(\Leftrightarrow x=\dfrac{11}{27}\)
Vậy, \(x=\dfrac{11}{27}\)
`c)`
\(-4\left(x-3\right)=6x+\left(x-3\right)\)
\(\Leftrightarrow-4x+12=6x+x-3\)
\(\Leftrightarrow-4x-7x=-12-3\)
\(\Leftrightarrow11x=-15\)
\(\Leftrightarrow x=\dfrac{-15}{11}\)
Vậy, \(x=-\dfrac{15}{11}\)
`d)`
\(\dfrac{x}{3}-\dfrac{5x}{6}-\dfrac{5x}{12}=\dfrac{x}{4}-5\)
\(\Leftrightarrow\dfrac{4x}{12}-\dfrac{10x}{12}-\dfrac{5x}{12}=\dfrac{3x}{12}-\dfrac{60}{12}\)
\(\Leftrightarrow4x-10x-5x=3x-60\)
\(\Leftrightarrow-11x-3x=-60\)
\(\Leftrightarrow-14x=-60\)
\(\Leftrightarrow x=\dfrac{-60}{-14}=\dfrac{30}{7}\)
Vậy, \(x=\dfrac{30}{7}\)
`e)`
\(\dfrac{x-1}{2}-\dfrac{x+1}{15}-\dfrac{2x-13}{6}=0\)
\(\Leftrightarrow\dfrac{15\left(x-1\right)}{30}-\dfrac{2\left(x+1\right)}{30}-\dfrac{5\left(2x-13\right)}{30}=0\)
\(\Leftrightarrow15\left(x-1\right)-2\left(x+1\right)-5\left(2x-13\right)=0\)
\(\Leftrightarrow15x-15-2x-2-10x+65=0\)
\(\Leftrightarrow3x+48=0\)
\(\Leftrightarrow3x=-48\)
\(\Leftrightarrow x=-16\)
Vậy, \(x=-16.\)
`f)`
\(\dfrac{3\left(3-x\right)}{8}+\dfrac{2\left(5-x\right)}{3}=\dfrac{1-x}{2}-2\)
\(\Leftrightarrow\dfrac{9\left(3-x\right)}{24}+\dfrac{16\left(5-x\right)}{24}=\dfrac{12\left(1-x\right)}{24}-\dfrac{48}{24}\)
\(\Leftrightarrow27-9x+80-16x=12-12x-48\)
\(\Leftrightarrow-9x-16x+12x=12-48-27-80\)
\(\Leftrightarrow-13x=-143\)
\(\Leftrightarrow x=11\)
Vậy, `x = 11.`
`g)`
\(\dfrac{3\left(5x-2\right)}{4}-2=\dfrac{7x}{3}-5\left(x-7\right)\)
\(\Leftrightarrow\dfrac{9\left(5x-2\right)}{12}-\dfrac{24}{12}=\dfrac{28x}{12}-\dfrac{60\left(x-7\right)}{12}\)
\(\Leftrightarrow45x-18-24=28x-60x+420\)
\(\Leftrightarrow45x-28x+60x=42+420\)
\(\Leftrightarrow77x=462\)
\(\Leftrightarrow x=6\)
Vậy, `x = 6`
`h)`
\(\dfrac{x+5}{2}+\dfrac{3-2x}{4}=x-\dfrac{7+x}{6}\)
\(\Leftrightarrow\dfrac{6\left(x+5\right)}{12}+\dfrac{3\left(3-2x\right)}{12}=\dfrac{12x}{12}-\dfrac{2\left(7+x\right)}{12}\)
\(\Leftrightarrow6x+30+9-6x=12x-14-2x\)
\(\Leftrightarrow-10x=-53\)
\(\Leftrightarrow x=5,3\)
Vậy, `x = 5,3`
`i)`
\(\dfrac{x-3}{11}+\dfrac{x+1}{3}=\dfrac{x+7}{9}-1\)
\(\Leftrightarrow\dfrac{9\left(x-3\right)}{99}+\dfrac{33\left(x+1\right)}{99}=\dfrac{11\left(x+7\right)}{99}-\dfrac{99}{99}\)
\(\Leftrightarrow9x-27+33x+33=11x+77-99\)
\(\Leftrightarrow42x-11x=-22-6\)
\(\Leftrightarrow31x=-28\)
\(\Leftrightarrow x=-\dfrac{28}{31}\)
Vậy, \(x=-\dfrac{28}{31}.\)