Tìm giá trị nhỏ nhất của P= 5x^2 +x +2
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1. Không dịch được đề
2.
\(-1\le cos2x\le1\Rightarrow1\le y\le3\)
3.
a. \(-2\le2sinx\le2\Rightarrow-1\le y\le3\)
\(y_{min}=-1\) khi \(sinx=-1\Rightarrow x=-\dfrac{\pi}{2}+k2\pi\)
\(y_{max}=3\) khi \(sinx=1\Rightarrow x=\dfrac{\pi}{2}+k2\pi\)
b.
\(0\le cos^2x\le1\Rightarrow-1\le y\le2\)
\(y_{min}=-1\) khi \(cos^2x=1\Rightarrow x=k\pi\)
\(y_{max}=2\) khi \(cosx=0\Rightarrow x=\dfrac{\pi}{2}+k\pi\)
4.
\(y=\left(tanx-1\right)^2+2\ge2\)
\(y_{min}=2\) khi \(tanx=1\Rightarrow x=\dfrac{\pi}{4}+k\pi\)

Tham khảo:
Đỉnh S có tọa độ: \({x_S} = \dfrac{{ - b}}{{2a}} = \dfrac{{ - 2}}{{2.1}} = - 1;\,{y_S} = {\left( { - 1} \right)^2} + 2.( - 1) + 3 = 2.\)
Hay \(S\left( { - 1;2} \right).\)
Vì hàm số bậc hai có \(a = 1 > 0\) nên ta có bảng biến thiên sau:
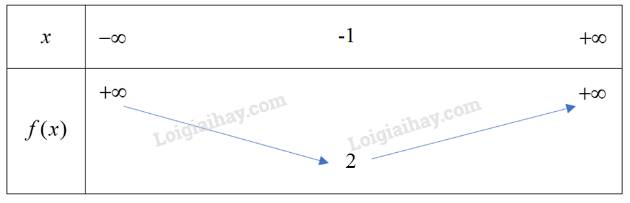
Hàm số đạt giá trị nhỏ nhất bằng \(2\).


Ta có f ' x = - m 2 + m + 1 x + 1 2 > 0
Suy ra f(x) là hàm đồng biến trên [0;1]
Do đó f 0 ≤ f x ≤ f 1 hay
- m 2 + m ≤ f x ≤ 1 2 - m 2 + m + 1
Khi đó
m i n x ∈ 0 ; 1 f x = - m 2 + m = - 2 ⇔ m = - 1 m = 2
Đáp án A
\(P\left(x\right)=5x^2+x+2=5\left(x^2+\dfrac{1}{5}x\right)+2\\ =5\left(x^2+2.x.\dfrac{1}{10}+\left(\dfrac{1}{10}\right)^2\right)-5.\left(\dfrac{1}{10}\right)^2+2\\ =5\left(x+\dfrac{1}{10}\right)^2+\dfrac{39}{20}\)
Nhận xét: \(\left(x+\dfrac{1}{10}\right)^2\ge0\forall x\inℝ\\ \Rightarrow5\left(x+\dfrac{1}{10}\right)^2\ge0\\ \Rightarrow P\left(x\right)=5\left(x+\dfrac{1}{10}\right)^2+\dfrac{39}{20}\ge\dfrac{39}{20}\)
\(Min_{P\left(x\right)}=\dfrac{39}{20}\) tại \(\left(x+\dfrac{1}{10}\right)^2=0\Leftrightarrow x+\dfrac{1}{10}=0\Leftrightarrow x=-\dfrac{1}{10}\)