xác định hệ số a và b sao cho :x^3+ax+b chia hết cho x^2+4x+3
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Ta có x3 + ax + b \(⋮\)x2 - 2x - 3
<=> x3 + ax + b \(⋮\)(x - 3)(x + 1)
=> x = 3 và x = -1 là nghiệm của x3 + ax + b
Khi đó 33 + 3a + b = 0
<=> 3a + b = -27 (1)
Lại có -13 - a + b = 0
<=> -a + b = 1 (2)
Từ (1) và (2) => a = -7 ; b = -6
Vậy a = -7 ; b = -6 thì x3 + ax + b \(⋮\)x2 - 2x - 3

Gỉar sử \(A:B\) được thương là \(4x+c\)
DO \(A⋮B\) nên \(A:B\) được dư bằng 0
Khi đó
\(4x^3+ax^2+bx+5=\left(4x+c\right)\left(x^2-x+1\right)\)
\(=4x^3+cx^2-4x^2-cx+4x+c\)
\(=4x^3+x^2\left(c-4\right)+x\left(4-c\right)+c\)
Áp dụng đồng nhất thức ta có
\(\left\{{}\begin{matrix}a=c-4\\b=4-c\\c=5\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}a=1\\b=-1\end{matrix}\right.\)
Vậy...

Bài 3:
\(\dfrac{f\left(x\right)}{g\left(x\right)}=\dfrac{x^4+ax^2+b}{x^2-3x+2}\)
\(=\dfrac{x^4-3x^3+2x^2+3x^3-9x^2+6x+\left(a+7\right)x^2-3x\left(a+7\right)+2\left(a+7\right)+x\left(-6+3a+7\right)+b-2a-14}{x^2-3x+2}\)
Để đây là phép chia hết thì 3a+1=0 và b-2a-14=0
=>a=-1/3; b=2a+14=-2/3+14=40/3

\(f\left(x\right)=2x^4+ax^2+bx+c\)
\(=2x^4-4x^3+4x^3-8x^2+\left(a+8\right)x^2-x\left(2a+16\right)+\left(2a+16+b\right)x-2\left(2a+16+b\right)+4a+32+2b+c\)
\(=\left(x-2\right)\left(2x^3+4x^2+x\left(a+8\right)+2a+16+b\right)+4a+2b+32+c\)
=>\(\dfrac{f\left(x\right)}{x-2}=2x^3+4x^2+x\left(a+8\right)+2a+16+b+\dfrac{4a+2b+32+c}{x-2}\)
f(x) chia hết cho x-2 nên \(4a+2b+32+c=0\)(1)
\(f\left(x\right)=2x^4+ax^2+bx+c\)
\(=2x^4-4x^3+6x^2+4x^3-16x^2+12x+\left(a+10\right)x^2-4x\left(a+10\right)+3a+30+x\left(4a+28+b\right)+c-3a-30\)
\(=\left(x^2-4x+3\right)\left(2x^2+4x+a+10\right)\)+x(4a+28+b)+c-3a-30
f(x) chia cho x2-4x+3 dư -x+2 nên ta có:
\(\left\{{}\begin{matrix}4a+28+b=-1\\c-3a-30=2\end{matrix}\right.\)(2)
Từ (1),(2) ta có hệ phương trình:
\(\left\{{}\begin{matrix}4a+2b+32+c=0\\4a+b+28=-1\\c-3a=32\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}4a+2b+c=-32\\4a+b=-29\\-3a+c=32\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}b+c=-3\\-3a+c=32\\4a+b=-29\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}b+3a=-35\\4a+b=-29\\b+c=-3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}-a=-6\\4a+b=-29\\b+c=-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=6\\b=-29-4a=-29-4\cdot6=-53\\c=-3-b=-3-\left(-53\right)=50\end{matrix}\right.\)

\(\Leftrightarrow x^3-2x^2-2x^2+4x+2x-4-a+4⋮x-2\)
hay a=4

Lời giải:
Đặt $f(x)=ax^3+bx^2-11x+10$
$x^2+x-2=(x-1)(x+2)$
Do đó để $f(x)\vdots x^2+x-2$ thì $f(x)\vdots x-1$ và $f(x)\vdots x+2$
$\Leftrightarrow f(1)=f(-2)=0$ (theo định lý Bê-du về phép chia đa thức)
$\Leftrightarrow a+b-1=-8a+4b+32=0$
$\Leftrightarrow a=3; b=-2$
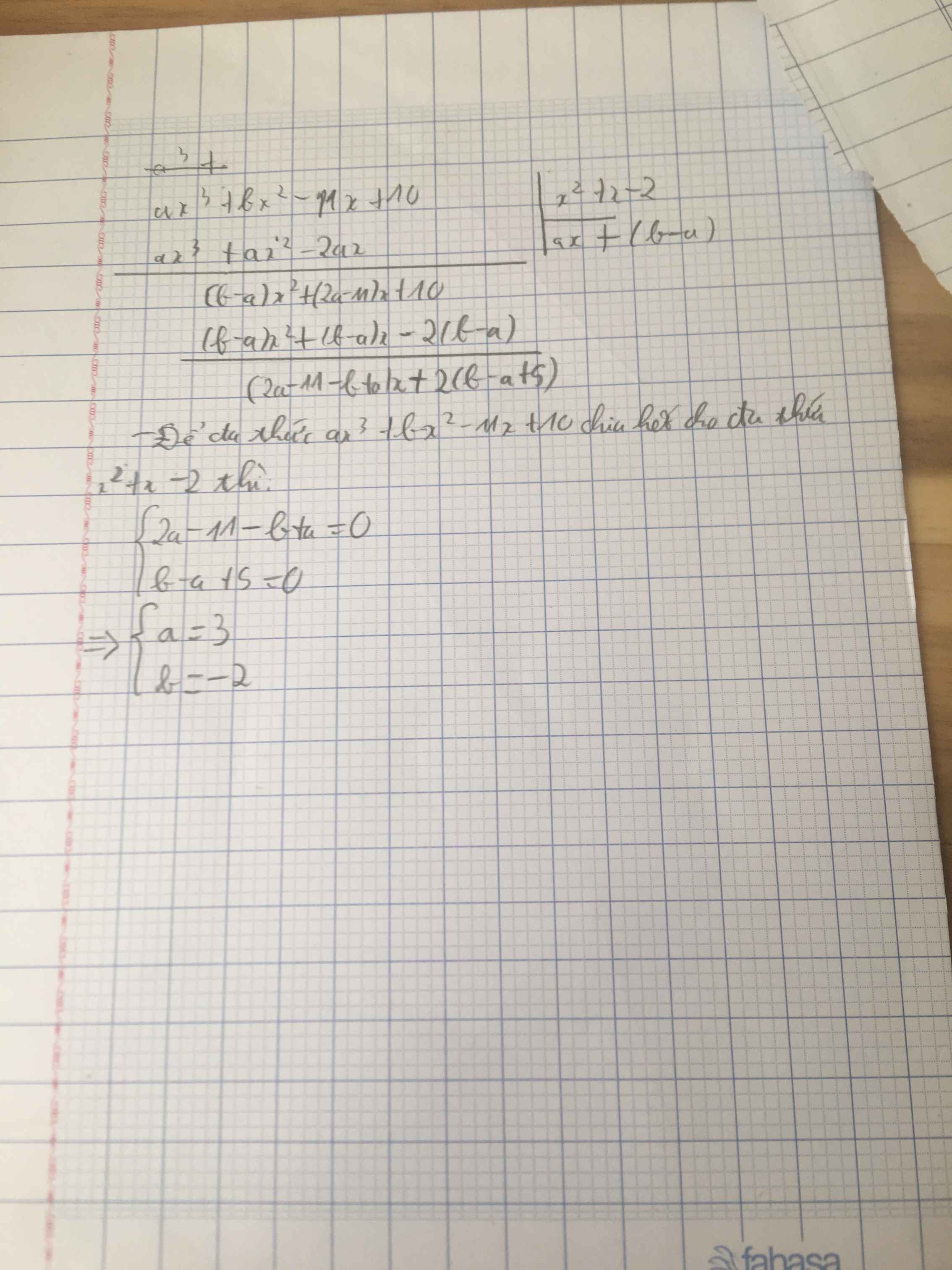
\(x^3+ax+b\\ =\left(x^3+4x^2+3x\right)+\left(-4x^2-16x-12\right)+\left(a+13\right)x+\left(b+12\right)\\ =x\left(x^2+4x+3\right)-4\left(x^2+4x+3\right)+\left(a+13\right)x+\left(b+12\right)\\ =\left(x-4\right)\left(x^2+4x+3\right)+\left(a+13\right)x+\left(b+12\right)\)
Để `x^3+ax+b` chia hết cho `x^2+4x+3` thì:
\(\left\{{}\begin{matrix}a+13=0\\b+12=0\end{matrix}\right.=>\left\{{}\begin{matrix}a=-13\\b=-12\end{matrix}\right.\)