3/1x2 + 3/2x3 + 3/3x4 + ..... 3/2021x2022
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PP
1
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
21 tháng 5 2023
A = \(\dfrac{1}{1\times2}\) + \(\dfrac{1}{2\times3}\) + \(\dfrac{1}{3\times4}\)+...+ \(\dfrac{1}{2021\times2022}\)
A = \(\dfrac{1}{1}\) - \(\dfrac{1}{2}\) + \(\dfrac{1}{2}\) - \(\dfrac{1}{3}\) + \(\dfrac{1}{3}\) - \(\dfrac{1}{4}\)+...+ \(\dfrac{1}{2021}\) - \(\dfrac{1}{2022}\)
A = 1 - \(\dfrac{1}{2022}\)
A = \(\dfrac{2021}{2022}\)
TU
2
29 tháng 9 2018
\(\frac{3}{1.2}+\frac{3}{2.3}+\frac{3}{3.4}+...+\frac{3}{14.15}\)
\(=3.\left(\frac{1}{1.2}+\frac{1}{2.3}+...+\frac{1}{14.15}\right)\)
\(=3.\left(1-\frac{1}{2}+\frac{1}{2}-\frac{1}{3}+\frac{1}{3}-\frac{1}{4}+...+\frac{1}{14}-\frac{1}{15}\right)\)
\(=3.\left(1-\frac{1}{15}\right)\)
\(=3.\frac{14}{15}\)
\(=\frac{14}{5}\)
VP
1
HT
2 tháng 8 2016
\(\frac{3}{1.2}+\frac{3}{2.3}+........+\frac{3}{99.100}\)
\(=3\left(\frac{1}{1.2}+\frac{1}{2.3}+.....+\frac{1}{99.100}\right)\)
\(=3\left(1-\frac{1}{2}+\frac{1}{2}-\frac{1}{3}+.........+\frac{1}{99}-\frac{1}{100}\right)\)
\(=3\left(1-\frac{1}{100}\right)\)
\(=\frac{3.99}{100}=\frac{297}{100}\)
7 tháng 3 2022
\(\Leftrightarrow2\left(x-\dfrac{1}{3}\right)\left(1-\dfrac{1}{2}+\dfrac{1}{2}-\dfrac{1}{3}+...+\dfrac{1}{9}-\dfrac{1}{10}\right)=\dfrac{3}{4}\)
\(\Leftrightarrow2\left(x-\dfrac{1}{3}\right)\left(1-\dfrac{1}{10}\right)=\dfrac{3}{4}\Leftrightarrow\dfrac{9}{10}\left(x-\dfrac{1}{3}\right)=\dfrac{3}{8}\)
\(\Leftrightarrow x-\dfrac{1}{3}=\dfrac{5}{12}\Leftrightarrow x=\dfrac{5}{12}+\dfrac{1}{3}=\dfrac{9}{12}=\dfrac{3}{4}\)
K
2
H9
HT.Phong (9A5)
CTVHS
9 tháng 1
\(A=1\times2+2\times3+3\times4+...+19\times20\)
\(A\times3=3\times\left(1\times2+2\times3+3\times4+...+19\times20\right)\)
\(A\times3=1\times2\times3+2\times3\times3+3\times4\times3+...+19\times20\times3\)
\(A\times3=1\times2\times3+2\times3\times\left(4-1\right)+3\times4\times\left(5-2\right)+....+19\times20\times\left(21-18\right)\)
\(A\times3=1\times2\times3-1\times2\times3+2\times3\times4-2\times3\times4+3\times4\times5+...+19\times20\times21\)
\(A\times3=\left(1\times2\times3-1\times2\times3\right)+\left(2\times3\times4-2\times3\times4\right)+...+\left(18\times19\times20-18\times19\times20\right)+19\times20\times21\)
\(A\times3=19\times20\times21\)
\(A\times3=7980\)
HV
5
AK
4 tháng 3 2018
a ) 3/5 + 3/16 + 13/16
= 3/5 + ( 3/16 + 13/16 )
= 3/5 + 16/16
= 3/5 + 1
= 3/5 + 5/5
= 8/5
b ) 1/1 x 2 + 1/ 2 x 3 + 1/ 3 x 4
= 1 - 1/2 + 1/2 - 1/3 + 1/3 - 1/4
= 1 - 1/4
= 4/4 - 1/4
= 3/4
26 tháng 3 2018
=3.(1/1.2+1/2.3+....+1/9.10)+11.(1/2.9+1/9.16+...+1/93.100)
=3.(1-1/2+1/2-1/3+1/3 - ... - 1/10)+11.(1/2-1/9+1/9-...-1/100)
= 3.(1-1/10) + 11.( 1/2-1/100)
= 3. 9/10+11.49/100
chỗ này bạn tự tính máy tính nha
LP
1
KV
30 tháng 10 2023
A = 1.2 + 2.3 + 3.4 + ... + 2017.2018
⇒ 3A = 1.2.3 + 2.3.(4 - 1) + 3.4.(5 - 2) + ... + 2017.218.(2019 - 2016)
= 1.2.3 + 2.3.4 - 1.2.3 + 3.4.5 - 2.3.4 + ... + 2017.2018.2019 - 2016.2017.2018
= 2017.2018.2019
= 2017.2018.2019
B = 2018³/3 ⇒ 3B = 2018³
Ta có:
2017.2019 = (2018 - 1).(2018 + 1)
= 2018² - 1²
= 2018.2018 - 1 < 2018.2018
⇒ 2017.2018.2019 < 2018.2018.2018
⇒ 3A < 3B
⇒ A < B
H9
HT.Phong (9A5)
CTVHS
15 tháng 7 2023
\(\dfrac{2}{1\cdot2}+\dfrac{2}{2\cdot3}+\dfrac{2}{3\cdot4}+...+\dfrac{2}{19\cdot20}\)
\(=2\cdot\left(1-\dfrac{1}{2}+\dfrac{1}{2}-\dfrac{1}{3}+\dfrac{1}{3}-\dfrac{1}{4}+...+\dfrac{1}{19}-\dfrac{1}{20}\right)\)
\(=2\cdot\left(1-\dfrac{1}{20}\right)\)
\(=2\cdot\dfrac{19}{20}\)
\(=\dfrac{19}{10}\)
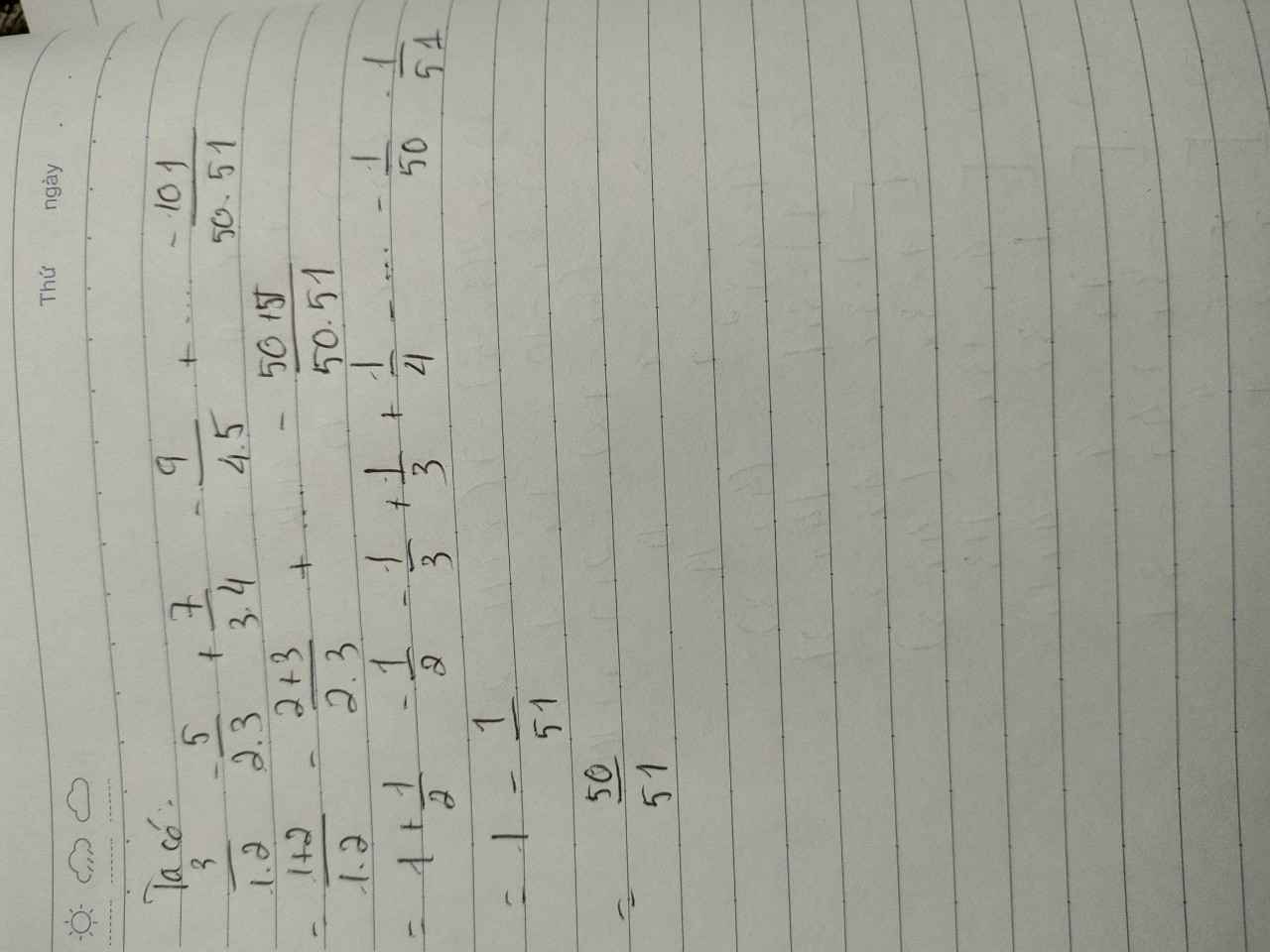
\(\dfrac{3}{1.2}+\dfrac{3}{2.3}+\dfrac{3}{3.4}+...+\dfrac{3}{2021.2022}\)
\(=3\left(\dfrac{1}{1.2}+\dfrac{1}{2.3}+...+\dfrac{1}{2021.2022}\right)\)
\(=3.\left(1-\dfrac{1}{2}+\dfrac{1}{2}-\dfrac{1}{3}+...+\dfrac{1}{2021}-\dfrac{1}{2022}\right)\)
\(=3.\left(1-\dfrac{1}{2022}\right)\)
\(=\dfrac{2021}{674}\)