Cho đa thức
A(x)=12x3+2ax+a2
B(x)=2x2-|2a+3|x+a2
Tìm a biết A(1)=B(-2)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
16 tháng 4 2021
b, Đặt \(B\left(x\right)=x^2-\dfrac{x}{2}=x\left(x-\dfrac{1}{2}\right)=0\)
\(\Leftrightarrow x=0;x=\dfrac{1}{2}\)Vậy nghiệm đa thức B(x) là x = 0 ; x = 1/2
c, Đặt \(C\left(x\right)=2x^2+4=2\left(x^2+2\right)=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}2\ne0\\x^2=-2\left(voli\right)\end{matrix}\right.\)Vậy đa thức C(x) vô nghiệm
d, Đặt \(D\left(x\right)=3x^4+7=0\Leftrightarrow x^4=-\dfrac{7}{3}\left(voli\right)\)
Vậy đa thức D(x) vô nghiệm
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 3 2022
Đa thức có nghiệm \(\Rightarrow\Delta'=a^2-\left(2a^2+b^2-5\right)\ge0\)
\(\Rightarrow a^2+b^2\le5\)
\(P=\left(a+1\right)\left(b+1\right)=ab+a+b+1=\dfrac{\left(a+b\right)^2-\left(a^2+b^2\right)}{2}+a+b+1\)
\(P\ge\dfrac{\left(a+b\right)^2-5}{2}+a+b+1=\dfrac{1}{2}\left(a+b+1\right)^2-2\ge-2\)
\(P_{min}=-2\) khi \(\left\{{}\begin{matrix}a^2+b^2=5\\a+b+1=0\end{matrix}\right.\) \(\Rightarrow\left(a;b\right)=\left(2;-1\right);\left(-1;2\right)\)
5 tháng 10 2019
Để phương trình có nghiệm thì :
\(\Delta_x=a^2-\left(2a^2+b^2-5\right)\ge0\)
\(\Leftrightarrow a^2+b^2\le5\)
\(\Leftrightarrow\left(a+b\right)^2\le5+2ab\)
\(\Leftrightarrow ab\ge\frac{\left(a+b\right)^2-5}{2}\)
Ta có :
\(P=\left(a+1\right)\left(b+1\right)=ab+a+b+1\)
\(\ge\frac{\left(a+b\right)^2-5}{2}+\left(a+b\right)+1=\frac{1}{2}\left(a+b+1\right)^2-2\ge-2\)
Dấu " = " xảy ra khi \(\hept{\begin{cases}a=-2\\b=1\end{cases}}\)
14 tháng 7 2021
a) Ta có: \(\dfrac{P}{x+2}=\dfrac{x^2+5x+6}{x^2+4x+4}\)
\(\Leftrightarrow\dfrac{P}{x+2}=\dfrac{\left(x+2\right)\left(x+3\right)}{\left(x+2\right)^2}=\dfrac{x+3}{x+2}\)
hay P=x+3
CM
17 tháng 11 2019
a) (x - 1)(x + l)(x - 2)(x - 4). b) (x - 2)( x 2 + 4).
c) 2y(3 x 2 + y 2 ). d) 2(x + y + z) ( a - b ) 2 .
24 tháng 8 2021
a. \(x^2\left(x-3\right)^2-\left(x-3\right)^2-x^2+1\)
\(=\left(x-3\right)^2\left(x^2-1\right)-\left(x^2-1\right)\)
\(=\left[\left(x-3\right)^2-1\right]\left(x^2-1\right)\)
\(=\left(x-3+1\right)\left(x-3-1\right)\left(x+1\right)\left(x-1\right)\)
\(=\left(x-2\right)\left(x-4\right)\left(x+1\right)\left(x-1\right)\)
b. \(x^3-2x^2+4x-8\)
\(=\left(x^3+4x\right)-\left(2x^2+8\right)\)
\(=x\left(x^2+4\right)-2\left(x^2+4\right)\)
\(=\left(x-2\right)\left(x^2+4\right)\)
c. \(\left(x+y\right)^3-\left(x-y\right)^3\)
\(=\left(x^3+3x^2y+3xy^2+y^3\right)-\left(x^3-3x^2y+3xy^2-y^3\right)\)
\(=x^3+3x^2y+3xy^2+y^3-x^3+3x^2y-3xy^2+y^3\)
\(=6x^2y+2y^3\)
\(=2y\left(3x^2+y^2\right)\)
d. \(2a^2\left(x+y+z\right)-4ab\left(x+y+z\right)+2b^2\left(x+y+z\right)\)
\(=\left(2a^2-4ab+2b^2\right)\left(x+y+z\right)\)
\(=2\left(a^2-2ab+b^2\right)\left(x+y+z\right)\)
\(=2\left(a-b\right)^2\left(x+y+z\right)\)
19 tháng 9 2023
a) Ta có:
B = (A + B) – A
= (x3 + 3x + 1) – (x4 + x3 – 2x – 2)
= x3 + 3x + 1 – x4 - x3 + 2x + 2
= – x4 + (x3 – x3) + (3x + 2x) + (1 + 2)
= – x4 + 5x + 3.
b) C = A - (A – C)
= x4 + x3 – 2x – 2 – x5
= – x5 + x4 + x3 – 2x – 2.
c) D = (2x2 – 3) . A
= (2x2 – 3) . (x4 + x3 – 2x – 2)
= 2x2 . (x4 + x3 – 2x – 2) + (-3) .(x4 + x3 – 2x – 2)
= 2x2 . x4 + 2x2 . x3 + 2x2 . (-2x) + 2x2 . (-2) + (-3). x4 + (-3) . x3 + (-3). (-2x) + (-3). (-2)
= 2x6 + 2x5 – 4x3 – 4x2 – 3x4 – 3x3 + 6x + 6
= 2x6 + 2x5 – 3x4 + (-4x3 – 3x3) – 4x2+ 6x + 6
= 2x6 + 2x5 – 3x4 – 7x3 – 4x2+ 6x + 6.
d) P = A : (x+1) = (x4 + x3 – 2x – 2) : (x + 1)
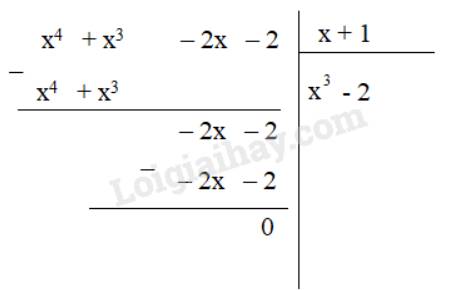
Vậy P = x3 - 2
e) Q = A : (x2 + 1)
Nếu A chia cho đa thức x2 + 1 không dư thì có một đa thức Q thỏa mãn
Ta thực hiện phép chia (x4 + x3 – 2x – 2) : (x2 + 1)
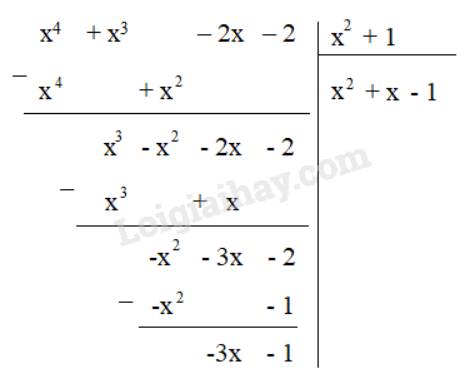
Do phép chia có dư nên không tồn tại đa thức Q thỏa mãn
22 tháng 10 2021
\(a,=\left(xy-1-x-y\right)\left(xy-1+x+y\right)\\ b,Sửa:a^3+2a^2+2a+1\\ =a^3+a^2+a^2+a+a+1=\left(a+1\right)\left(a^2+a+1\right)\\ c,=1-4a^2-a\left(a^2-4\right)=1-4a^2-a^3+4a\\ =\left(1-a\right)\left(1+a+a^2\right)+4a\left(1-a\right)\\ =\left(1-a\right)\left(1+5a+a^2\right)\\ d,=\left(a^2-a^2b^2\right)+\left(b^2-b\right)+\left(ab-a\right)\\ =a^2\left(1-b\right)\left(1+b\right)+b\left(b-1\right)+a\left(b-1\right)\\ =\left(b-1\right)\left(-a^2-ab+b+a\right)\\ =\left(b-1\right)\left(b-1\right)\left(a+b\right)\left(1-a\right)\)
\(e,=x^2y+xy^2-yz\left(y+z\right)+x^2z-xz^2\\ =\left(x^2y+x^2z\right)+\left(xy^2-xz^2\right)-yz\left(y+z\right)\\ =x^2\left(y+z\right)+x\left(y-z\right)\left(y+z\right)-yz\left(y+z\right)\\ =\left(y+z\right)\left(x^2+xy-xz-yz\right)\\ =\left(y+z\right)\left(x+y\right)\left(x-z\right)\)
\(f,=xyz-xy-yz-xz+x+y+z-1\\ =xy\left(z-1\right)-y\left(z-1\right)-x\left(z-1\right)+\left(x-1\right)\\ =\left(z-1\right)\left(xy-y-x+1\right)=\left(z-1\right)\left(x-1\right)\left(y-1\right)\)
Ta có \(A\left(1\right)=B\left(-2\right)\Leftrightarrow12+2a+a^2=8-\left|2a+3\right|\left(-2\right)+a^2\)
\(\Leftrightarrow4+2a=2\left|2a+3\right|\)
đk a >= -2
\(\left[{}\begin{matrix}4a+6=4+2a\\4a+6=-2a-4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}a=-1\left(tm\right)\\a=-\dfrac{5}{3}\left(ktm\right)\end{matrix}\right.\)