Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
\(f\left(x\right)=2x+\dfrac{3}{2x-4}-\left(3+\dfrac{3}{2x-4}\right)\)
\(f\left(x\right)=2x+\dfrac{3}{2x-4}-3-\dfrac{3}{2x-4}\)
\(f\left(x\right)=2x-3\)
Để f(x) âm thì :
\(2x-3< 0\)
\(\Leftrightarrow2x< 3\)
\(\Leftrightarrow x< \dfrac{3}{2}\)
Vậy C đúng
Have a nice day!
Chọn C
Ta có
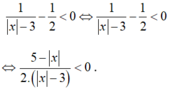
Đặt 
bpt trở thành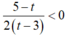 
Bảng xét dấu
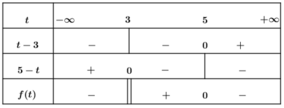
Căn cứ bảng xét dấu ta được
Chọn D.
Để f(x) = x(5x + 2) - x( x 2 + 6) không dương thì
x(5x + 2) - x(x2 + 6) ≤ 0 ⇔ x( x 2 - 5x + 4) ≥ 0
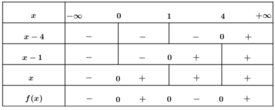
Vậy x ∈ [0;1] ∪ [4; + ∞ ).
\(f\left(x\right)=\dfrac{x^2-1}{x^2}=1-\dfrac{1}{x^2}\)
\(\int f\left(x\right)dx=\int\left(1-\dfrac{1}{x^2}\right)dx=\int1dx-\int x^{-2}dx\)
=\(x-\dfrac{x^{-2+1}}{-2+1}+C=x-\dfrac{x^{-1}}{-1}+C=x+\dfrac{1}{x}+C\)
C=-1 ta được phương án A(ko tm câu hỏi)
C=0 ta được phương án B(ko tm câu hỏi)
C=2 ta được phương án C(ko tm câu hỏi)
=>chọn D
a) Để \(\sqrt{\dfrac{x}{3}}\) có nghĩa thì \(\dfrac{x}{3}\ge0\Leftrightarrow x\ge0\)
b) Để \(\sqrt{-5x}\) có nghĩa thì \(-5x\ge0\Leftrightarrow x\le0\)
c) Để \(\sqrt{4-x}\) có nghĩa thì \(4-x\ge0\Leftrightarrow x\le4\)
d) Để \(\sqrt{3x+7}\) có nghĩa thì \(3x+7\ge0\Leftrightarrow x\ge-\dfrac{7}{3}\)
e) Để \(\sqrt{-3x+4}\) có nghĩa thì \(-3x+4\ge0\Leftrightarrow x\le\dfrac{4}{3}\)
f) Để \(\sqrt{\dfrac{1}{-1+x}}\) có nghĩa thì \(\left\{{}\begin{matrix}\dfrac{1}{-1+x}\ge0\\-1+x\ne0\end{matrix}\right.\)
\(\Leftrightarrow-1+x>0\Leftrightarrow x>1\)
g) Để \(\sqrt{1+x^2}\) có nghĩa thì \(1+x^2\ge0\left(đúng\forall x\right)\)
h) \(\sqrt{\dfrac{5}{x-2}}\) có nghĩ thì \(\left\{{}\begin{matrix}\dfrac{5}{x-2}\ge0\\x-2\ne0\end{matrix}\right.\)
\(\Leftrightarrow x-2>0\Leftrightarrow x>2\)
Xét f(x) = \(x\left(x^2-1\right)=x\left(x-1\right)\left(x+1\right)\)
f(x) = 0 khi x = 0 hoặc x = 1 hoặc x = -1
Ta có bảng
x \(-\infty\) -1 0 1 \(+\infty\)
x - | - 0 + | +
x-1 - | - | - 0 +
x+1 - 0 + | + | +
f(x) - 0 + 0 - 0 +
=> f(x) \(\ge0\Leftrightarrow x\in\left[-1;0\right]\cup\left[1;+\infty\right]\)
2: ĐKXĐ: x<>1
\(f'\left(x\right)=\dfrac{\left(x^2-3x+3\right)'\left(x-1\right)-\left(x^2-3x+3\right)\left(x-1\right)'}{\left(x-1\right)^2}\)
\(=\dfrac{\left(2x-3\right)\left(x-1\right)-\left(x^2-3x+3\right)}{\left(x-1\right)^2}\)
\(=\dfrac{2x^2-5x+3-x^2+3x-3}{\left(x-1\right)^2}=\dfrac{x^2-2x}{\left(x-1\right)^2}\)
f'(x)=0
=>x^2-2x=0
=>x(x-2)=0
=>\(\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
1:
\(f\left(x\right)=\dfrac{1}{3}x^3-2\sqrt{2}\cdot x^2+8x-1\)
=>\(f'\left(x\right)=\dfrac{1}{3}\cdot3x^2-2\sqrt{2}\cdot2x+8=x^2-4\sqrt{2}\cdot x+8=\left(x-2\sqrt{2}\right)^2\)
f'(x)=0
=>\(\left(x-2\sqrt{2}\right)^2=0\)
=>\(x-2\sqrt{2}=0\)
=>\(x=2\sqrt{2}\)
Ý D
tại sao vậy ạ ???