Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Chọn C
Ta có
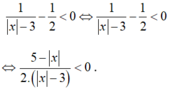
Đặt ![]() bpt trở thành
bpt trở thành 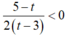
Bảng xét dấu
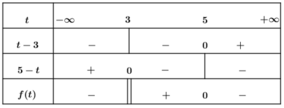
Căn cứ bảng xét dấu ta được
![]()

\(\left|2x-5\right|-3\le0\)
\(\Leftrightarrow\left|2x-5\right|\le3\)
\(\Leftrightarrow-3\le2x-5\le3\)
\(\Leftrightarrow1\le x\le4\)

\(\left|2x-5\right|-3\le0\)
\(\Leftrightarrow\left|2x-5\right|\le3\)
\(\Leftrightarrow-3\le2x-5\le3\)
\(\Leftrightarrow1\le x\le4\)
\(\Rightarrow x\in\left[1;4\right]\) (và các tập con của nó)

Chọn D.
Để f(x) = x(5x + 2) - x( x 2 + 6) không dương thì
x(5x + 2) - x(x2 + 6) ≤ 0 ⇔ x( x 2 - 5x + 4) ≥ 0
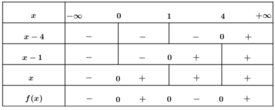
Vậy x ∈ [0;1] ∪ [4; + ∞ ).

(-1; +\infty )(−1;+∞)
(-\infty ; -2)(−∞;−2)
(-2; +\infty )(−2;+∞)
(-2; -1 )(−2;−1)
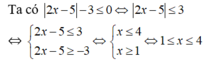
Xét f(x) = \(x\left(x^2-1\right)=x\left(x-1\right)\left(x+1\right)\)
f(x) = 0 khi x = 0 hoặc x = 1 hoặc x = -1
Ta có bảng
x \(-\infty\) -1 0 1 \(+\infty\)
x - | - 0 + | +
x-1 - | - | - 0 +
x+1 - 0 + | + | +
f(x) - 0 + 0 - 0 +
=> f(x) \(\ge0\Leftrightarrow x\in\left[-1;0\right]\cup\left[1;+\infty\right]\)