a) S= 1/1*2 + 1/2*3 + 1/3*4 + ...............+ 1/99*100
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
13 tháng 7 2018
Ta có :
\(A=\dfrac{1}{2\sqrt{1}+1\sqrt{2}}+\dfrac{1}{3\sqrt{2}+2\sqrt{3}}+\dfrac{1}{4\sqrt{3}+3\sqrt{4}}+.....+\dfrac{1}{100\sqrt{99}+99\sqrt{100}}\)
\(=\dfrac{1}{\sqrt{1}}-\dfrac{1}{\sqrt{2}}+\dfrac{1}{\sqrt{2}}-\dfrac{1}{\sqrt{3}}+\dfrac{1}{\sqrt{3}}-\dfrac{1}{\sqrt{4}}+........+\dfrac{1}{\sqrt{99}}-\dfrac{1}{\sqrt{100}}\) \(=1-\dfrac{1}{\sqrt{100}}< 1\)
Vậy \(A< 1\)
HT
1
HD
28 tháng 7 2018
a, S= 1/1*2 + 1/2*3 + 1/3*4 +...+1/99*100
S= 1/1 - 1/2 + 1/2 - 1/3 + 1/3 - 1/4 +...+ 1/99 - 1/100
S= 1/1 - 1/100
S= 100/100 - 1/100
S= 99/100
b, S= 1/1*3 + 1/3*5 + 1/5*7 +...+1/99*101
S= 1/2* (2/1*3 + 2/3*5 + 2/5*7 +...+ 2/99*101)
S= 1/2* (1/1 - 1/3 + 1/3 - 1/5 + 1/5 - 1/7 +...+ 1/99 - 1/101)
S= 1/2* (1/1 - 1/101)
S= 1/2* (101/101 - 1/101)
S= 1/2* 100/101
S= 50/101
Chúc bạn học tốt nha
HB
0
17 tháng 7 2021
Ta có: \(S=\dfrac{1}{2+\sqrt{2}}+\dfrac{1}{3\sqrt{2}+2\sqrt{3}}+\dfrac{1}{4\sqrt{3}+3\sqrt{4}}+...+\dfrac{1}{100\sqrt{99}+99\sqrt{100}}\)
\(=1-\dfrac{1}{\sqrt{2}}+\dfrac{1}{\sqrt{2}}-\dfrac{1}{\sqrt{3}}+...+\dfrac{1}{\sqrt{99}}-\dfrac{1}{10}\)
\(=1-\dfrac{1}{10}=\dfrac{9}{10}\)
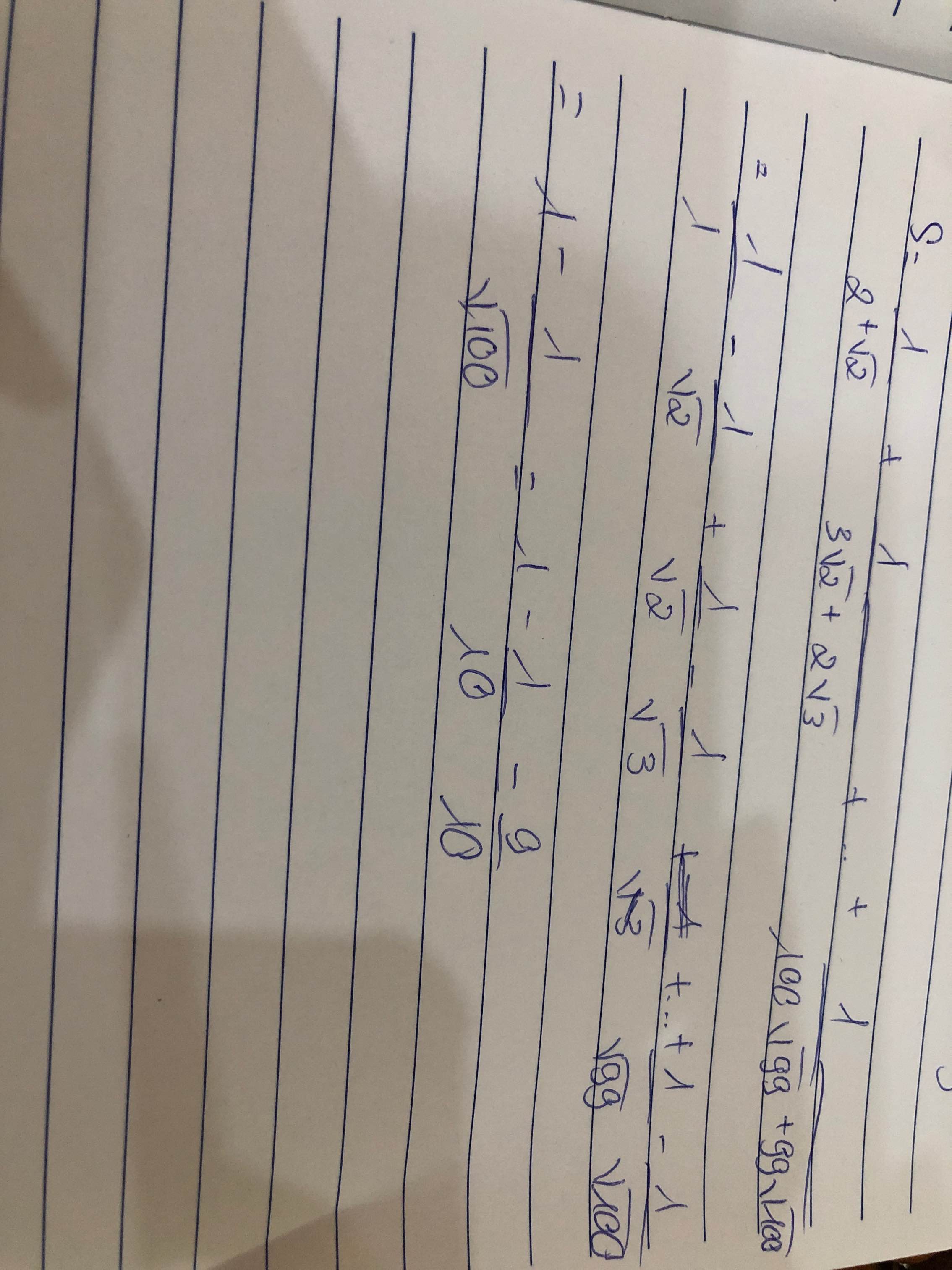
S=1/1*2+1/2*3+1/3*4+......+1/99*100
S=1-1/2+1/2-1/3+1/3-1/4+......+1/99-1/00
S=1-1/100
S=99/1000
vậy S=99/100