giúp mik 2 câu này với ạ, mik cảm ơn nhiều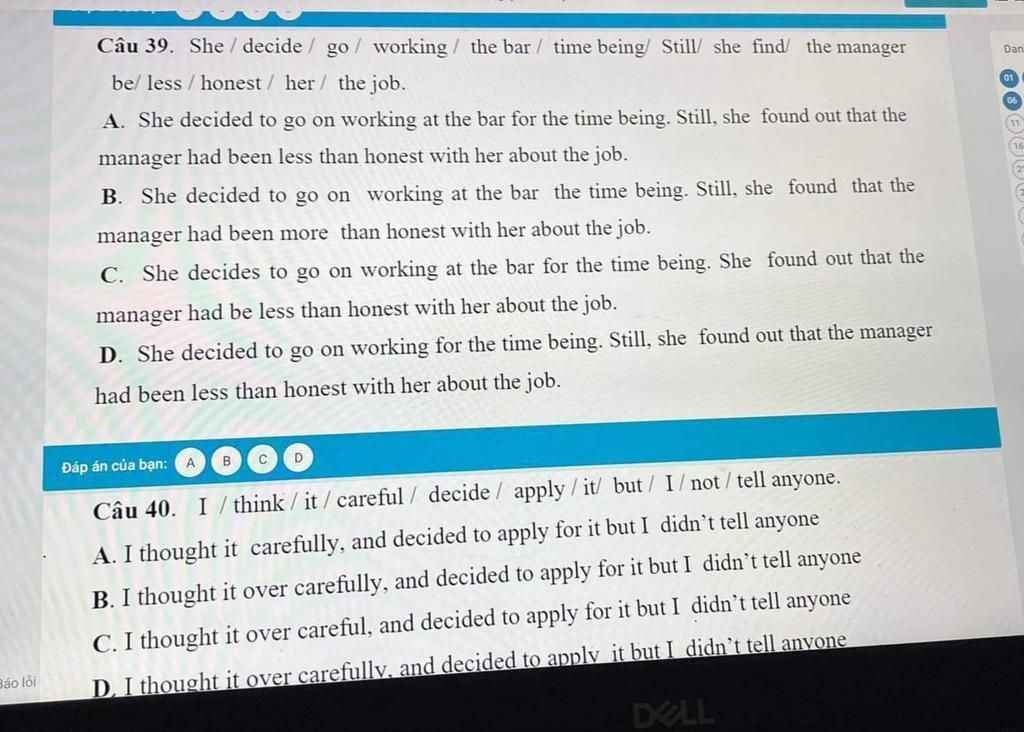
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1 It was such a difficult climb that we stopped to rest several times
2 She didn't ran fast enough to win the race
3 It was such a heavy bag that I had to ask for help
4 The house is too small for us to live in
5 Jack's suit was so elegant that everyone complimented him
6 My sister is not old enough to watch horror films
7 My mother is such a wise person that people often aske her for advice
8 The package is not light enough for you to lift by yourself
9 This book is too old for the children to eat
10 It was such an interesting book that I couldn't put it down
11 It is such a weak bird that it can't fly
12 These boyss aren't old enough to watch that film
13 They are such small sandals that they don't fit me

\(25.24+25.48+75.16+75.56\)
\(=25.\left(24+48\right)+75.\left(16+56\right)\)
\(=25.72+75.72\)
\(=\left(25+75\right).72\)
\(=100.72\)
\(=7200\)

19.
\(\left(a+b\right)^2\le2\left(a^2+b^2\right)=4\Rightarrow-2\le a+b\le2\)
\(P=3\left(a+b\right)+ab=3\left(a+b\right)+\dfrac{\left(a+b\right)^2-\left(a^2+b^2\right)}{2}=\dfrac{1}{2}\left(a+b\right)^2+3\left(a+b\right)-1\)
Đặt \(a+b=x\Rightarrow-2\le x\le2\)
\(P=\dfrac{1}{2}x^2+3x-1=\dfrac{1}{2}\left(x+2\right)\left(x+4\right)-5\ge-5\) (đpcm)
Dấu "=" xảy ra khi \(x=-2\) hay \(a=b=-1\)
20.
Đặt \(P=2a+2ab+abc\)
\(P=2a+ab\left(2+c\right)\le2a+\dfrac{a}{4}\left(b+2+c\right)^2=2a+\dfrac{a}{4}\left(7-a\right)^2\)
\(P\le\dfrac{1}{4}\left(a^3-14a^2+57a-72\right)+18=18-\dfrac{1}{4}\left(8-a\right)\left(a-3\right)^2\le18\) (đpcm)
Dấu "=" xảy ra khi \(\left(a;b;c\right)=\left(3;2;0\right)\)

3 . ( 2x - 1 ) - 2 = 13
3 . ( 2x - 1 ) = 12 + 3
3 . ( 2x - 1 ) = 15
2x - 1 = 15 : 3
2x - 1 = 5
2x = 5 + 1 = 6
x = 6 : 2 = 3
Vậy x = 3
\(3\left(2x-1\right)-2=13\)
\(3\left(2x-1\right)=15\)
\(2x-1=5\)
\(2x=6\)
\(x=3\)

a: \(Q=\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}+2\right)-2\sqrt{x}\left(\sqrt{x}-2\right)-5\sqrt{x}-2}{x-4}:\dfrac{\sqrt{x}\left(3-\sqrt{x}\right)}{\left(\sqrt{x}+2\right)^2}\)
\(=\dfrac{x+3\sqrt{x}+2-2x+4\sqrt{x}-5\sqrt{x}-2}{x-4}\cdot\dfrac{\left(\sqrt{x}+2\right)^2}{\sqrt{x}\left(3-\sqrt{x}\right)}\)
\(=\dfrac{-x+2\sqrt{x}}{\sqrt{x}-2}\cdot\dfrac{\sqrt{x}+2}{\sqrt{x}\left(3-\sqrt{x}\right)}\)
\(=\dfrac{-\sqrt{x}\left(\sqrt{x}-2\right)}{\sqrt{x}\left(\sqrt{x}-2\right)\cdot\left(-1\right)}\cdot\dfrac{\sqrt{x}+2}{\sqrt{x}-3}=\dfrac{\sqrt{x}+2}{\sqrt{x}-3}\)
b: Khi x=4-2căn 3 thì \(Q=\dfrac{\sqrt{3}-1+2}{\sqrt{3}-1-3}=\dfrac{\sqrt{3}+1}{\sqrt{3}-4}=\dfrac{-7-5\sqrt{3}}{13}\)
c: Q>1/6
=>Q-1/6>0
=>\(\dfrac{\sqrt{x}+2}{\sqrt{x}-3}-\dfrac{1}{6}>0\)
=>\(\dfrac{6\sqrt{x}+12-\sqrt{x}+3}{6\left(\sqrt{x}-3\right)}>0\)
=>\(\dfrac{5\sqrt{x}+9}{6\left(\sqrt{x}-3\right)}>0\)
=>căn x-3>0
=>x>9

Câu 2:
Ta có: \(x^2-2\left(m+1\right)x+m^2+4=0\)
a=1; b=-2m-2; \(c=m^2+4\)
\(\text{Δ}=b^2-4ac\)
\(=\left(-2m-2\right)^2-4\cdot\left(m^2+4\right)\)
\(=4m^2+8m+4-4m^2-16\)
=8m-12
Để phương trình có hai nghiệm phân biệt thì Δ>0
\(\Leftrightarrow8m>12\)
hay \(m>\dfrac{3}{2}\)
Áp dụng hệ thức Vi-et, ta được:
\(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)=2m+2\\x_1x_2=m^2+4\end{matrix}\right.\)
Vì x1 là nghiệm của phương trình nên ta có:
\(x_1^2-2\left(m+1\right)\cdot x_1+m^2+4=0\)
\(\Leftrightarrow x_1^2=2\left(m+1\right)x_1-m^2-4\)
Ta có: \(x_1^2+2\left(m+1\right)x_2=2m^2+20\)
\(\Leftrightarrow2\left(m+1\right)x_1-m^2-4+2\left(m+1\right)x_2-2m^2-20=0\)
\(\Leftrightarrow2\left(m+1\right)\left(x_1+x_2\right)-3m^2-24=0\)
\(\Leftrightarrow2\left(m+1\right)\cdot\left(2m+2\right)-3m^2-24=0\)
\(\Leftrightarrow4m^2+8m+4-3m^2-24=0\)
\(\Leftrightarrow m^2+8m-20=0\)
Đến đây bạn tự tìm m là xong rồi

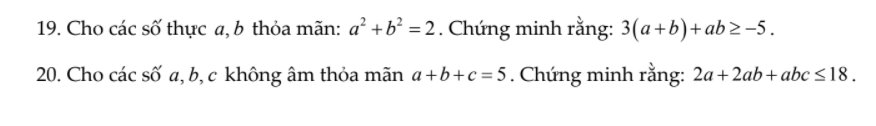
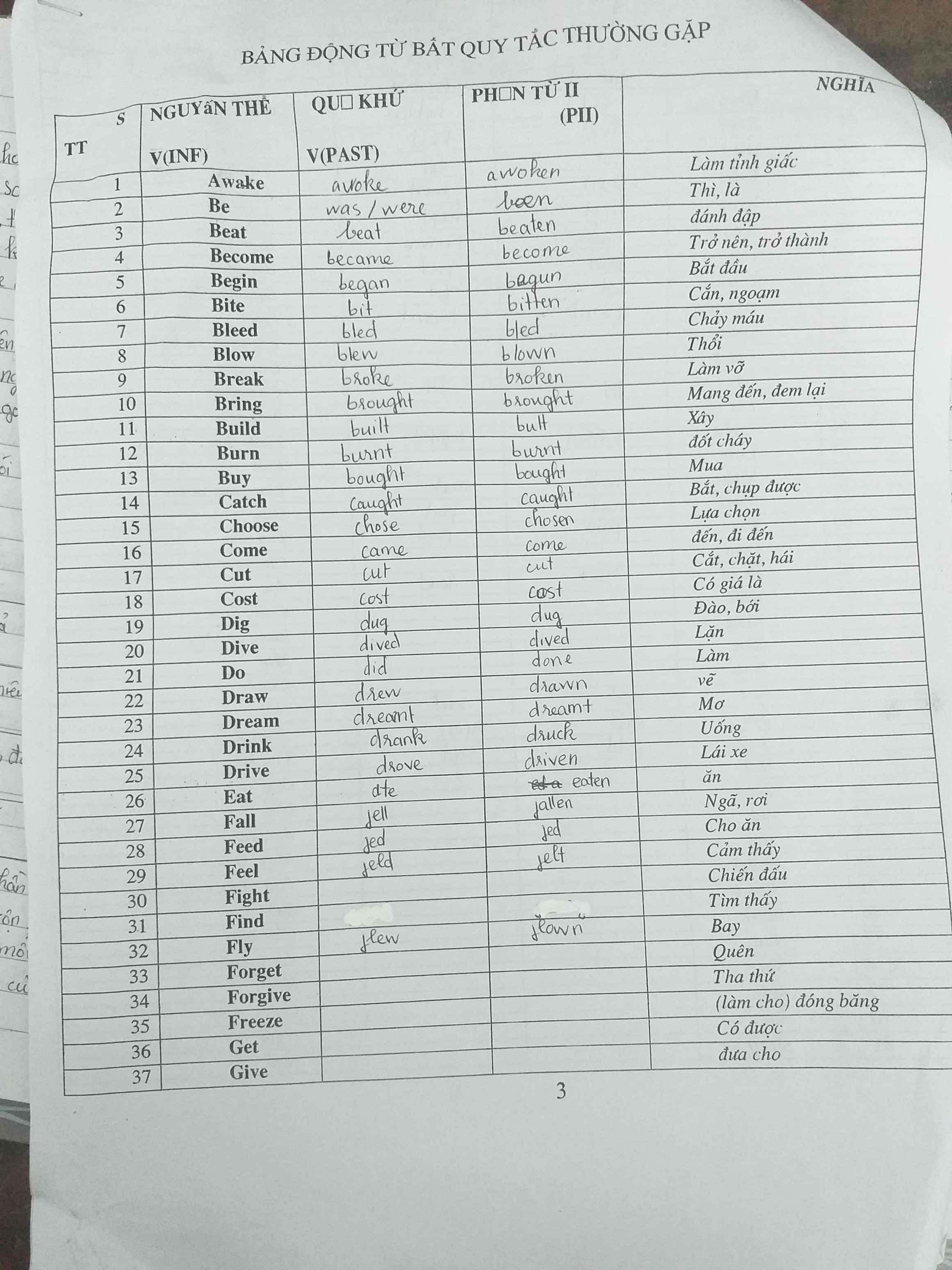

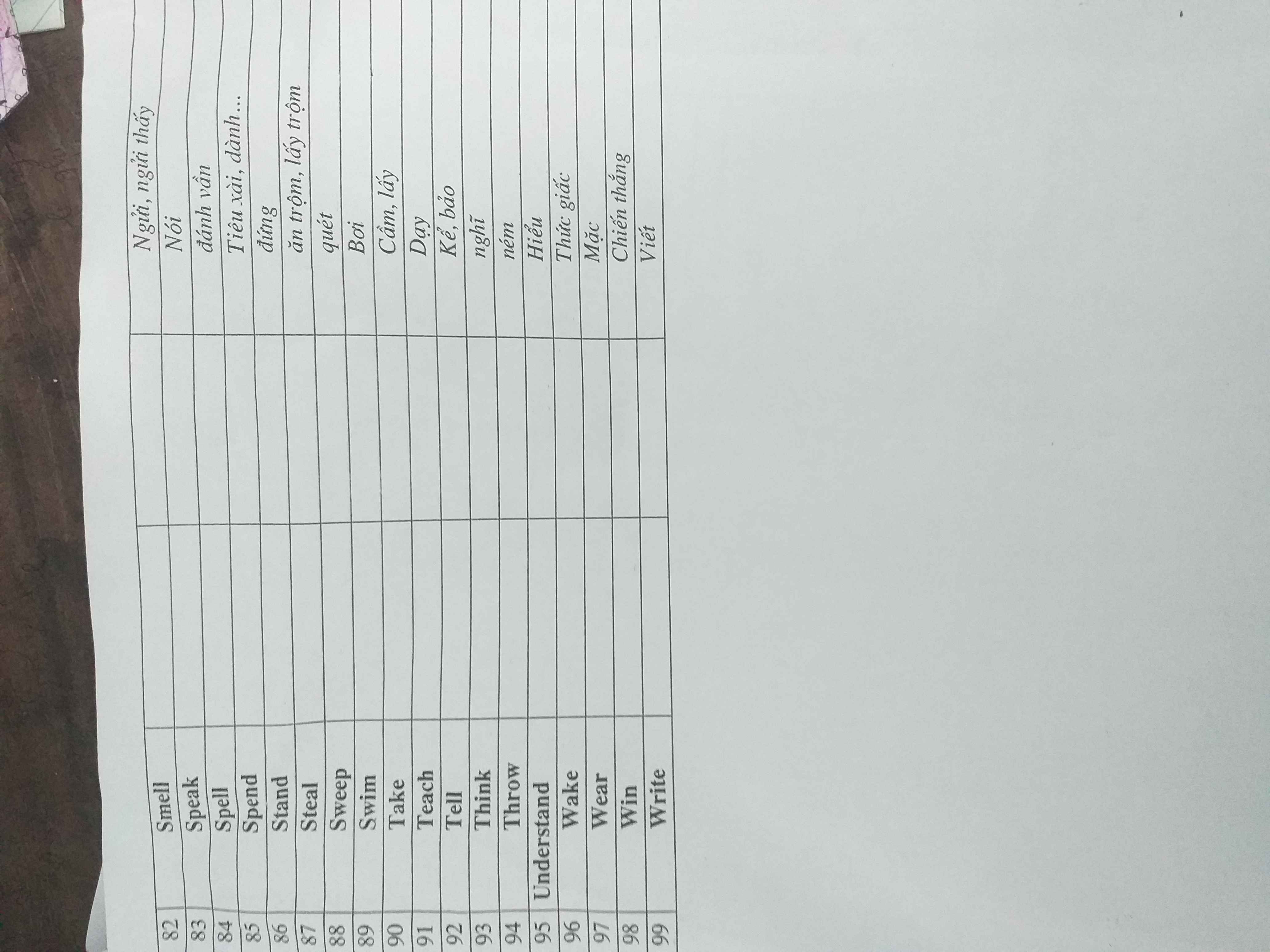
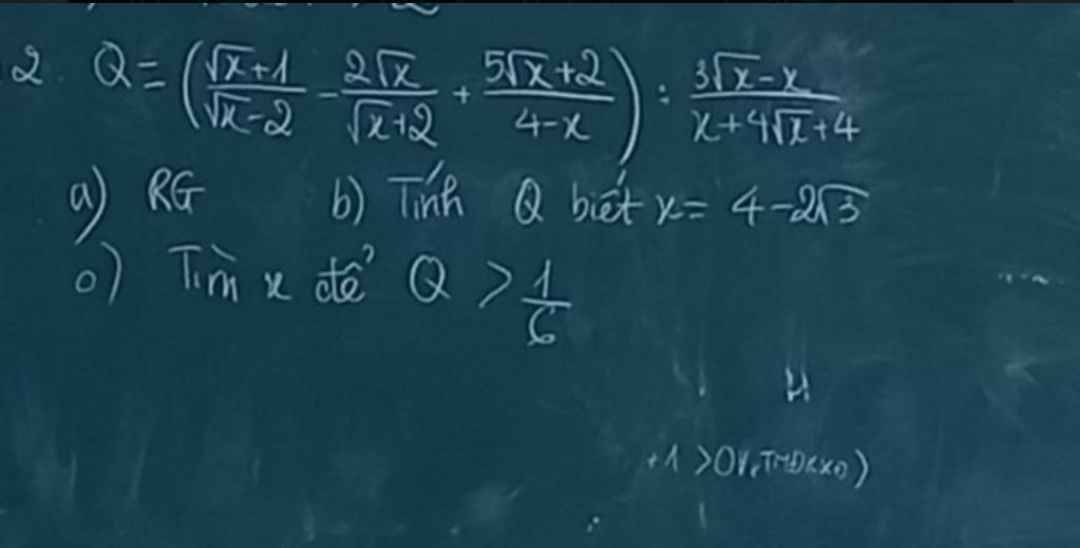
 Mn ơi, giải hộ mik câu này với! Tối nay mik phải nộp rồi ạ! Cảm ơn mn nhiều lắm!
Mn ơi, giải hộ mik câu này với! Tối nay mik phải nộp rồi ạ! Cảm ơn mn nhiều lắm!
