Tính giá trị của biểu thức P = ln ( 2 cos 1 0 ) . ln ( 2 cos 2 0 ) . ln ( 2 cos 3 0 ) . . . ln ( 2 cos 89 0 ) với tích đã cho bao gồm 89 thừa số có dạng ln ( 2 cos a 0 ) với 1 ≤ a ≤ 89 và a ∈ Z
A. P = -1
B. P = 0
C. P = 1
D. P = 2 89 89 !
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

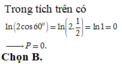

\(A=\int\limits^{0.5}_{-0.5}cos\left[ln\left(\frac{1-x}{1+x}\right)\right]dx\) hay \(A=\int\limits^{0.5}_{-0.5}cos\left[\frac{ln\left(1-x\right)}{1+x}\right]dx\)
Dù thế nào thì có lẽ người ra đề cũng nhầm lẫn, đây là 1 bài toán ko thể giải quyết trong chương trình phổ thông, nếu hàm là hàm sin chứ ko phải cos thì còn có cơ hội làm được trong chương trình 12
Tích phân sửa lại như sau thì giải quyết được bằng phương pháp thông thường:
\(A=\int\limits^{0.5}_{-0.5}sin\left[ln\left(\frac{1-x}{1+x}\right)\right]dx\)
Vì hàm dưới dấu tích phân là hàm lẻ nên chỉ cần đặt \(x=-t\) sau đó đổi biến và cộng lại là suy ra ngay lập tức \(A=0\)
\(B=\int\limits^{\frac{\pi}{2}}_0\frac{cos^3x}{cos^3x+sin^3x}dx\) (1)
Đặt \(\frac{\pi}{2}-x=t\Rightarrow dx=-dt;\left\{{}\begin{matrix}x=0\Rightarrow t=\frac{\pi}{2}\\x=\frac{\pi}{2}\Rightarrow t=0\end{matrix}\right.\)
\(B=\int\limits^0_{\frac{\pi}{2}}\frac{sin^3t}{sin^3t+cos^3t}\left(-dt\right)=\int\limits^{\frac{\pi}{2}}_0\frac{sin^3t}{sin^3t+cos^3t}dt=\int\limits^{\frac{\pi}{2}}_0\frac{sin^3x}{sin^3x+cos^3x}dx\) (2)
Cộng vế với vế của (1) và (2):
\(2B=\int\limits^{\frac{\pi}{2}}_0\frac{sin^3x+cos^3x}{sin^3x+cos^3x}dx=\int\limits^{\frac{\pi}{2}}_0dx=\frac{\pi}{2}\Rightarrow B=\frac{\pi}{4}\)
c/ \(C=\int\limits^{\frac{\pi}{2}}_0\left(\sqrt{sinx}-\sqrt{cosx}\right)dx\) (1)
Đặt \(\frac{\pi}{2}-x=t\Rightarrow dx=-dt;\left\{{}\begin{matrix}x=0\Rightarrow t=\frac{\pi}{2}\\x=\frac{\pi}{2}\Rightarrow t=0\end{matrix}\right.\)
\(C=\int\limits^0_{\frac{\pi}{2}}\left(\sqrt{cost}-\sqrt{sint}\right)\left(-dt\right)=\int\limits^{\frac{\pi}{2}}_0\left(\sqrt{cost}-\sqrt{sint}\right)dt=\int\limits^{\frac{\pi}{2}}_0\left(\sqrt{cosx}-\sqrt{sinx}\right)dx\left(2\right)\)
Cộng vế với vế của (1) và (2):
\(2C=\int\limits^{\frac{\pi}{2}}_0\left(\sqrt{sinx}-\sqrt{cosx}+\sqrt{cosx}-\sqrt{sinx}\right)dx=0\)
\(\Rightarrow C=0\)
//Các dạng bài này đều giống nhau, nếu biểu thức đối xứng sin, cos và cận \(0;\frac{\pi}{2}\) thì đặt \(\frac{\pi}{2}-x=t\) rồi biến đổi và cộng lại

\(P=4\left[\left(cos^21^0+cos^289^0\right)+\left(cos^22^0+cos^288^0\right)+...+\left(cos^244^0+cos^246^0\right)+cos^245^0\right]\)
\(=4\left[\left(cos^21^0+sin^21^0\right)+\left(cos^22^0+sin^22^0\right)+...+\left(cos^244^0+sin^244^0\right)+cos^245^0\right]\)
\(=4\left(1+1+...+1+\frac{\sqrt{2}}{2}\right)\)

\(\cos^21^o+\cos^289^o=\cos^21^o+\cos^2\left(90^o-1^o\right)=\cos^21^o+\sin^21^o=1\)
\(\cos^22^o+\cos^288^o=\cos^22^o+\cos^2\left(90^o-2^o\right)=\cos^22^o+\sin^22^o=1\)
.......
\(\cos^244^o+\cos^246^o=\cos^244^o+\cos^2\left(90^o-44^o\right)=\cos^244^o+\sin^244^o=1\)
\(\cos^245^o=\left(\frac{\sqrt{2}}{2}\right)^2=\frac{1}{2}\)
=> \(A=1.44+\frac{1}{2}-\frac{1}{2}=44\)

Ta có \(y'=\frac{\cos\left(\ln x\right)-\sin\left(\ln x\right)}{x}\)
\(\Rightarrow y"=\frac{x.\frac{-\sin\left(\ln x\right)-\cos\left(\ln x\right)}{x}-\left[\cos\left(\ln x\right)-\sin\left(\ln x\right)\right]}{x^2}=\frac{-2\cos\left(\ln x\right)}{x^2}\)
Ta có :
\(y+xy'+x^2y"=\sin\left(\ln x\right)+\cos\left(\ln x\right)+\cos\left(\ln x\right)-\sin\left(\ln x\right)-2\cos\left(\ln x\right)=0\)

Ta có : \(y=\sin\left(\ln x\right)+\cos\left(\ln x\right)\Rightarrow\begin{cases}y'=\frac{1}{x}\cos\left(\ln x\right)-\frac{1}{x}\sin\left(\ln x\right)=\frac{\cos\left(\ln x\right)-\sin\left(\ln x\right)}{x}\\y"=\frac{\left[-\frac{1}{x}\sin\left(\ln x\right)-\frac{1}{x}\cos\left(\ln x\right)\right]x-\left[\cos\left(\ln x\right)-\sin\left(\ln x\right)\right]}{x^2}=\frac{-2\cos\left(\ln x\right)}{x^2}\end{cases}\)
\(\Rightarrow y+xy'+x^2y"=\sin\left(\ln x\right)+\cos\left(\ln x\right)+\cos\left(\ln x\right)-\sin\left(\ln x\right)-2\cos\left(\ln x\right)=0\)
=> Điều cần chứng minh

a)
Đặt \(A = \left( {2\sin {{30}^o} + \cos {{135}^o} - 3\tan {{150}^o}} \right).\left( {\cos {{180}^o} - \cot {{60}^o}} \right)\)
Ta có: \(\left\{ \begin{array}{l}\cos {135^o} = - \cos {45^o};\cos {180^o} = - \cos {0^o}\\\tan {150^o} = - \tan {30^o}\end{array} \right.\)
\( \Rightarrow A = \left( {2\sin {{30}^o} - \cos {{45}^o} + 3\tan {{30}^o}} \right).\left( { - \cos {0^o} - \cot {{60}^o}} \right)\)
Sử dụng bảng giá trị lượng giác của một số góc đặc biệt, ta có:
\(\left\{ \begin{array}{l}\sin {30^o} = \frac{1}{2};\tan {30^o} = \frac{{\sqrt 3 }}{3}\\\cos {45^o} = \frac{{\sqrt 2 }}{2};\cos {0^o} = 1;\cot {60^o} = \frac{{\sqrt 3 }}{3}\end{array} \right.\)
\( \Rightarrow A = \left( {2.\frac{1}{2} - \frac{{\sqrt 2 }}{2} + 3.\frac{{\sqrt 3 }}{3}} \right).\left( { - 1 - \frac{{\sqrt 3 }}{3}} \right)\)
\(\begin{array}{l} \Leftrightarrow A = - \left( {1 - \frac{{\sqrt 2 }}{2} + \sqrt 3 } \right).\left( {1 + \frac{{\sqrt 3 }}{3}} \right)\\ \Leftrightarrow A = - \frac{{2 - \sqrt 2 + 2\sqrt 3 }}{2}.\frac{{3 + \sqrt 3 }}{3}\\ \Leftrightarrow A = - \frac{{\left( {2 - \sqrt 2 + 2\sqrt 3 } \right)\left( {3 + \sqrt 3 } \right)}}{6}\\ \Leftrightarrow A = - \frac{{6 + 2\sqrt 3 - 3\sqrt 2 - \sqrt 6 + 6\sqrt 3 + 6}}{6}\\ \Leftrightarrow A = - \frac{{12 + 8\sqrt 3 - 3\sqrt 2 - \sqrt 6 }}{6}.\end{array}\)
b)
Đặt \(B = {\sin ^2}{90^o} + {\cos ^2}{120^o} + {\cos ^2}{0^o} - {\tan ^2}60 + {\cot ^2}{135^o}\)
Ta có: \(\left\{ \begin{array}{l}\cos {120^o} = - \cos {60^o}\\\cot {135^o} = - \cot {45^o}\end{array} \right. \Rightarrow \left\{ \begin{array}{l}{\cos ^2}{120^o} = {\cos ^2}{60^o}\\{\cot ^2}{135^o} = {\cot ^2}{45^o}\end{array} \right.\)
\( \Rightarrow B = {\sin ^2}{90^o} + {\cos ^2}{60^o} + {\cos ^2}{0^o} - {\tan ^2}60 + {\cot ^2}{45^o}\)
Sử dụng bảng giá trị lượng giác của một số góc đặc biệt, ta có:
\(\left\{ \begin{array}{l}\cos {0^o} = 1;\;\;\cot {45^o} = 1;\;\;\cos {60^o} = \frac{1}{2}\\\tan {60^o} = \sqrt 3 ;\;\;\sin {90^o} = 1\end{array} \right.\)
\( \Rightarrow B = {1^2} + {\left( {\frac{1}{2}} \right)^2} + {1^2} - {\left( {\sqrt 3 } \right)^2} + {1^2}\)
\( \Leftrightarrow B = 1 + \frac{1}{4} + 1 - 3 + 1 = \frac{1}{4}.\)
c
Đặt \(C = \cos {60^o}.\sin {30^o} + {\cos ^2}{30^o}\)
Sử dụng bảng giá trị lượng giác của một số góc đặc biệt, ta có:
\(\sin {30^o} = \frac{1}{2};\;\;\cos {30^o} = \frac{{\sqrt 3 }}{2};\;\cos {60^o} = \frac{1}{2}\;\)
\( \Rightarrow C = \frac{1}{2}.\frac{1}{2} + {\left( {\;\frac{{\sqrt 3 }}{2}} \right)^2} = \frac{1}{4} + \frac{3}{4} = 1.\)

\(a,y'=8x^3-9x^2+10x\\ \Rightarrow y''=24x^2-18x+10\\ b,y'=\dfrac{2}{\left(3-x\right)^2}\\ \Rightarrow y''=\dfrac{4}{\left(3-x\right)^3}\)
\(c,y'=2cos2xcosx-sin2xsinx\\ \Rightarrow y''=-5sin\left(2x\right)cos\left(x\right)-4cos\left(2x\right)sin\left(x\right)\\ d,y'=-2e^{-2x+3}\\ \Rightarrow y''=4e^{-2x+3}\)

\(P=\dfrac{2sin\alpha-3cos\alpha}{3sin\alpha+2cos\alpha}\\ =\dfrac{\dfrac{2sin\alpha}{cos\alpha}-\dfrac{3cos\alpha}{cos\alpha}}{\dfrac{3sin\alpha}{cos\alpha}+\dfrac{2cos\alpha}{cos\alpha}}\\ =\dfrac{2tan\alpha-3}{3tan\alpha+2}=\dfrac{2.3-3}{3.3+2}=\dfrac{3}{11}\)
Ta có: \(1 + {\tan ^2}\alpha = \frac{1}{{{{\cos }^2}\alpha }}\quad (\alpha \ne {90^o})\)
\( \Rightarrow \frac{1}{{{{\cos }^2}\alpha }} = 1 + {3^2} = 10\)
\( \Leftrightarrow {\cos ^2}\alpha = \frac{1}{{10}} \Leftrightarrow \cos \alpha = \pm \frac{{\sqrt {10} }}{{10}}\)
Vì \({0^o} < \alpha < {180^o}\) nên \(\sin \alpha > 0\).
Mà \(\tan \alpha = 3 > 0 \Rightarrow \cos \alpha > 0 \Rightarrow \cos \alpha = \frac{{\sqrt {10} }}{{10}}\)
Lại có: \(\sin \alpha = \cos \alpha .\tan \alpha = \frac{{\sqrt {10} }}{{10}}.3 = \frac{{3\sqrt {10} }}{{10}}.\)
\( \Rightarrow P = \dfrac{{2.\frac{{3\sqrt {10} }}{{10}} - 3.\frac{{\sqrt {10} }}{{10}}}}{{3.\frac{{3\sqrt {10} }}{{10}} + 2.\frac{{\sqrt {10} }}{{10}}}} = \dfrac{{\frac{{\sqrt {10} }}{{10}}\left( {2.3 - 3} \right)}}{{\frac{{\sqrt {10} }}{{10}}\left( {3.3 + 2} \right)}} = \dfrac{3}{{11}}.\)