Thực hiện phép tính
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

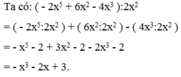

Bài 1:
b: \(=\dfrac{x+3-4-x}{x-2}=\dfrac{-1}{x-2}\)
Bài 2:
a: \(=\dfrac{x+1}{2\left(x+3\right)}+\dfrac{2x+3}{x\left(x+3\right)}\)
\(=\dfrac{x^2+x+4x+6}{2x\left(x+3\right)}=\dfrac{x^2+5x+6}{2x\left(x+3\right)}=\dfrac{x+2}{2x}\)
d: \(=\dfrac{3}{2x^2y}+\dfrac{5}{xy^2}+\dfrac{x}{y^3}\)
\(=\dfrac{3y^2+10xy+2x^3}{2x^2y^3}\)
e: \(=\dfrac{x^2+2xy+x^2-2xy-4xy}{\left(x+2y\right)\left(x-2y\right)}=\dfrac{2x^2-4xy}{\left(x+2y\right)\cdot\left(x-2y\right)}=\dfrac{2x}{x+2y}\)

Bài 3:
3: \(6x\left(x-y\right)-9y^2+9xy\)
\(=6x\left(x-y\right)+9xy-9y^2\)
\(=6x\left(x-y\right)+9y\left(x-y\right)\)
\(=\left(x-y\right)\left(6x+9y\right)\)
\(=3\left(2x+3y\right)\left(x-y\right)\)
Bài 4:
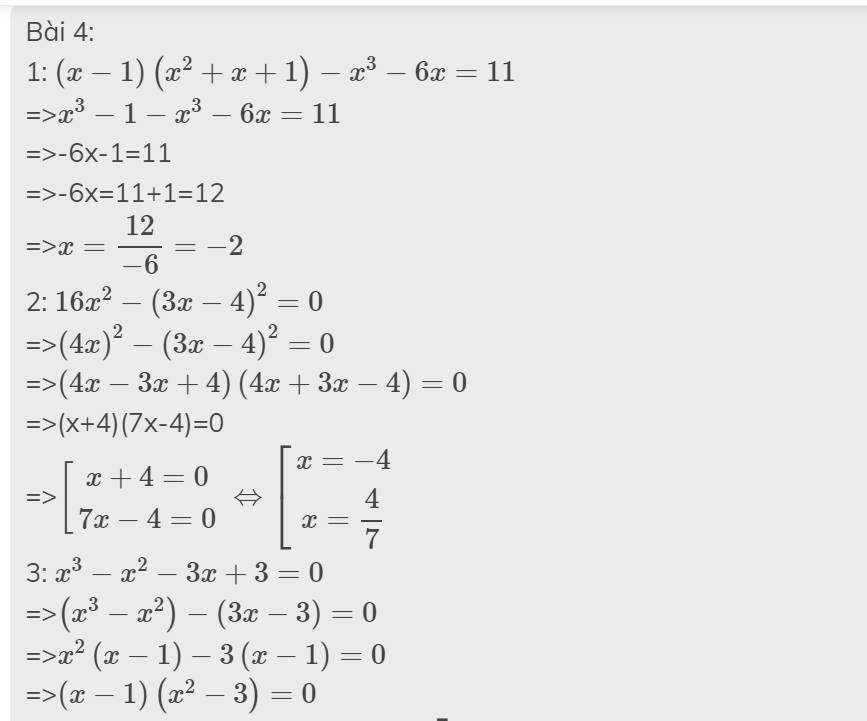

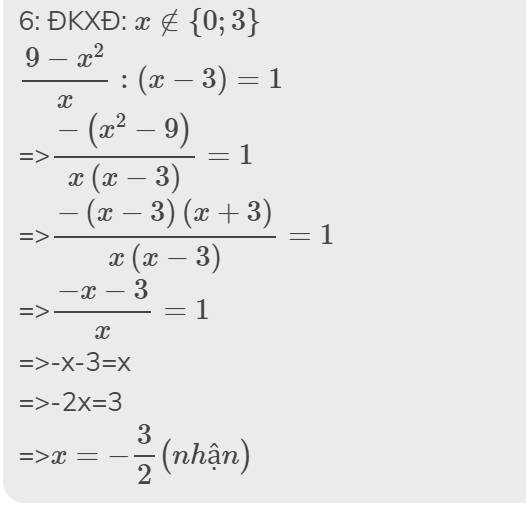

Bài 2:
1: \(A=\left(x+2\right)\left(x^2-2x+4\right)+2\left(x+1\right)\left(1-x\right)\)
\(=\left(x+2\right)\left(x^2-x\cdot2+2^2\right)-2\left(x+1\right)\left(x-1\right)\)
\(=x^3+2^3-2\left(x^2-1\right)\)
\(=x^3+8-2x^2+2=x^3-2x^2+10\)
\(B=\left(2x-y\right)^2-2\left(4x^2-y^2\right)+\left(2x+y\right)^2+4\left(y+2\right)\)
\(=\left(2x-y\right)^2-2\cdot\left(2x-y\right)\left(2x+y\right)+\left(2x+y\right)^2+4\left(y+2\right)\)
\(=\left(2x-y-2x-y\right)^2+4\left(y+2\right)\)
\(=\left(-2y\right)^2+4\left(y+2\right)\)
\(=4y^2+4y+8\)
2: Khi x=2 thì \(A=2^3-2\cdot2^2+10=8-8+10=10\)
3: \(B=4y^2+4y+8\)
\(=4y^2+4y+1+7\)
\(=\left(2y+1\right)^2+7>=7>0\forall y\)
=>B luôn dương với mọi y
Bài 1:
5: \(x^2\left(x-y+1\right)+\left(x^2-1\right)\left(x+y\right)\)
\(=x^3-x^2y+x^2+x^3+x^2y-x-y\)
\(=2x^3-x+x^2-y\)
6: \(\left(3x-5\right)\left(2x+11\right)-6\left(x+7\right)^2\)
\(=6x^2+33x-10x-55-6\left(x^2+14x+49\right)\)
\(=6x^2+23x-55-6x^2-84x-294\)
=-61x-349

1.(2x+3).(x-5)+2x(3-x)+x-10
=2x^2 -10x+3x-15+6x-2x^2+x-10
=2x-25
2.(-x-2)3+(2x-4).(x2+2x+4)-x2.(x-6)
=-x^3+6x^2-12x-8+2x^3+4x^2+8x-4x^2+8x-16-x^3+6x^2

Đáp án:
a.3x³−5x²+7xa.3x³−5x²+7x
b.−4x²y−10x²y+2xyb.−4x²y−10x²y+2xy
c.−x³+2x²+29x+20c.−x³+2x²+29x+20
d.2x⁴−3x³+2x²+3x−4d.2x⁴−3x³+2x²+3x−4
e.x²−4y²e.x²−4y²
h.2x²−6x+13h.2x²−6x+13
g.3xy⁴

a) \({x^2} + \dfrac{1}{4}{x^2} - 5{x^2} = (1 + \dfrac{1}{4} - 5){x^2} = - \dfrac{{15}}{4}{x^2}\);
b) \({y^4} + 6{y^4} - \dfrac{2}{5}{y^4} = (1 + 6 - \dfrac{2}{5}){y^4} = \dfrac{{33}}{5}{y^4}\).

\(\dfrac{5x+2}{x^2-4}+\dfrac{x-5}{x-2}=\dfrac{5x+2+x^2-3x-10}{\left(x-2\right)\left(x+2\right)}\\ =\dfrac{x^2+2x-8}{\left(x-2\right)\left(x+2\right)}=\dfrac{\left(x+4\right)\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}=\dfrac{x+4}{x+2}\\ \left(x+4\right)^2-\left(x+3\right)\left(x-2\right)=-13\\ \Leftrightarrow x^2+8x+16-x^2+x+6=-13\\ \Leftrightarrow9x=-13-22=-35\\ \Leftrightarrow x=-\dfrac{35}{9}\)

Trả lời:
Bài 4:
b, B = ( x + 1 ) ( x7 - x6 + x5 - x4 + x3 - x2 + x - 1 )
= x8 - x7 + x6 - x5 + x4 - x3 + x2 - x + x7 - x6 + x5 - x4 + x3 - x2 + x - 1
= x8 - 1
Thay x = 2 vào biểu thức B, ta có:
28 - 1 = 255
c, C = ( x + 1 ) ( x6 - x5 + x4 - x3 + x2 - x + 1 )
= x7 - x6 + x5 - x4 + x3 - x2 + x + x6 - x5 + x4 - x3 + x2 - x + 1
= x7 + 1
Thay x = 2 vào biểu thức C, ta có:
27 + 1 = 129
d, D = 2x ( 10x2 - 5x - 2 ) - 5x ( 4x2 - 2x - 1 )
= 20x3 - 10x2 - 4x - 20x3 + 10x2 + 5x
= x
Thay x = - 5 vào biểu thức D, ta có:
D = - 5
Bài 5:
a, A = ( x3 - x2y + xy2 - y3 ) ( x + y )
= x4 + x3y - x3y - x2y2 + x2y2 + xy3 - xy3 - y4
= x4 - y4
Thay x = 2; y = - 1/2 vào biểu thức A, ta có:
A = 24 - ( - 1/2 )4 = 16 - 1/16 = 255/16
b, B = ( a - b ) ( a4 + a3b + a2b2 + ab3 + b4 )
= a5 + a4b + a3b2 + a2b3 + ab4 - ab4 - a3b2 - a2b3 - ab4 - b5
= a5 + a4b - ab4 - b5
Thay a = 3; b = - 2 vào biểu thức B, ta có:
B = 35 + 34.( - 2 ) - 3.( - 2 )4 - ( - 2 )5 = 243 - 162 - 48 + 32 = 65
c, ( x2 - 2xy + 2y2 ) ( x2 + y2 ) + 2x3y - 3x2y2 + 2xy3
= x4 + x2y2 - 2x3y - 2xy3 + 2x2y2 + 2y4 + 2x3y - 3x2y2 + 2xy3
= x4 + 2y4
Thay x = - 1/2; y = - 1/2 vào biểu thức trên, ta có:
( - 1/2 )4 + 2.( - 1/2 )4 = 1/16 + 2. 1/16 = 1/16 + 1/8 = 3/16

Bài 3:
Gọi số nhóm là x
Theo đề, ta có: \(x\in\left\{1;2;3;4;6;9;12;18;36\right\}\)
mà 2<x<6
nên \(x\in\left\{3;4\right\}\)
Vậy: Có 2 cách chia nhóm
a) 25 - x = 12 + 6 =18
x=25-18=7 Vậy x=7
b) 7 + 2 x ( x -3 ) = 11
2.(x-3)=11-7=4
x-3=4:2=2
x=3+2=5
c) 102 : ( 2.x + 13) : 4) = 6
(2.x+13):4=102:6=17
2.x+13=17.4=68
2.x=68-13=55
x=27,5 Vậy x=27,5
Bài 3:
Gọi số nhóm là x
Theo đề, ta có: x∈{1;2;3;4;6;9;12;18;36}x∈{1;2;3;4;6;9;12;18;36}
mà 2<x<6
nên x∈{3;4}x∈{3;4}
Vậy: Có 2 cách chia nhóm
còn bài 1 chắc bn làm đc nha tick mk nha

\(\left(x-2\right)\left(x^2+2x+4\right)-\left(x^3+5\right)\)
\(=x^3-8-x^3-5\)
=-13
\(\left(x-2\right)\cdot\left(x^2+2x+4\right)-\left(x^3+5\right)\\ =x^2-8-x^3-5\\ =-13\)