mik chụp rõ hơn rồi, giúp mik câu 5, 6 với
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
NN
5
Những câu hỏi liên quan
10 tháng 1 2023
a: Xét tứ giác ADHE có
góc ADH=góc AEH=góc DAE=90 độ
nên ADHE là hình chữ nhật
b: \(HD=\sqrt{10^2-8^2}=6\left(cm\right)\)
\(S_{ADHE}=6\cdot8=48\left(cm^2\right)\)
c: Để ADHE là hình vuông thì AH là phân giác của góc BAC
=>góc B=45 độ
TS
2
5 tháng 11 2023
OM\(\perp\)AB
=>\(\widehat{MOA}=\widehat{MOB}=90^0\)
Trên cùng một nửa mặt phẳng bờ chứa tia OA, ta có: \(\widehat{AOE}< \widehat{AOM}\)
nên tia OE nằm giữa hai tia OA và OM
=>\(\widehat{AOE}+\widehat{MOE}=\widehat{AOM}=90^0\)
Trên cùng một nửa mặt phẳng bờ chứa tia OB, ta có: \(\widehat{BOF}< \widehat{BOM}\)
nên tia OF nằm giữa hai tia OB và OM
=>\(\widehat{BOF}+\widehat{MOF}=\widehat{BOM}=90^0\)
=>\(\widehat{AOE}+\widehat{MOE}=\widehat{BOF}+\widehat{MOF}\)
mà \(\widehat{AOE}=\widehat{BOF}\)
nên \(\widehat{MOE}=\widehat{MOF}\)
=>OM là phân giác của \(\widehat{EOF}\)
BS
19 tháng 12 2022
\(\dfrac{x+9}{x^2-9}-\dfrac{3}{x^2+3x}\)
\(=\dfrac{x+9}{\left(x-3\right)\left(x+3\right)}-\dfrac{3}{x\left(x+3\right)}\)
\(=\dfrac{x\left(x+9\right)}{x\left(x-3\right)\left(x+3\right)}-\dfrac{3\left(x-3\right)}{x\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{x^2+9x-3x+9}{x\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{x^2+6x+9}{x\left(x-3\right)\left(x+3\right)}=\dfrac{\left(x+3\right)^2}{x\left(x-3\right)\left(x+3\right)}=\dfrac{x+3}{x\left(x-3\right)}\)
______________________________________________________
\(\dfrac{x+1}{2x+6}-\dfrac{x-6}{2x^2+6x}\)
\(=\dfrac{x+1}{2\left(x+3\right)}-\dfrac{x-6}{2x\left(x+3\right)}\)
\(=\dfrac{x\left(x+1\right)}{2x\left(x+3\right)}-\dfrac{x-6}{2x\left(x+3\right)}\)
\(=\dfrac{x^2+x-x+6}{2x\left(x+3\right)}=\dfrac{x^2+6}{2x\left(x+3\right)}\)
10 tháng 1 2023
a: \(=\dfrac{x^3+2x+2x-2-x^2-x-1}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{x^3-x^2+3x-3}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^2+3}{x^2+x+1}\)
b: \(=\dfrac{x^2-2x-3+x^2+2x-3+2x-2x^2}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{2x-6}{\left(x-3\right)\left(x+3\right)}=\dfrac{2}{x+3}\)
c: \(=\dfrac{6-7+x}{3\left(x-1\right)}=\dfrac{x-1}{3\left(x-1\right)}=\dfrac{1}{3}\)
d: \(=\dfrac{x^3+2x+2x-2-x^2-x-1}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^3-x^2+3x-3}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^2+3}{x^2+x+1}\)
9 tháng 1 2023
a: Xét ΔABC có BM/BC=BD/BA
nên MD//AC
=>MM' vuông góc AB
=>M đối xứngM' qua AB
b: Xét tứ giác AMBM' có
D là trung điểm chung của AB và MM'
MA=MB
Do đó: AMBM' là hình thoi
6 tháng 1 2023
a: ĐKXĐ: x<>2; x<>-3
b: \(P+\dfrac{x^2-4-5-x-3}{\left(x-2\right)\left(x+3\right)}=\dfrac{\left(x-4\right)\left(x+3\right)}{\left(x-2\right)\left(x+3\right)}=\dfrac{x-4}{x-2}\)
c: Để P=-3/4 thì x-4/x-2=-3/4
=>4x-8=-3x+6
=>7x=14
=>x=2(loại)
e: x^2-9=0
=>x=3 (nhận) hoặc x=-3(loại)
Khi x=3 thì \(P=\dfrac{3-4}{3-2}=-1\)
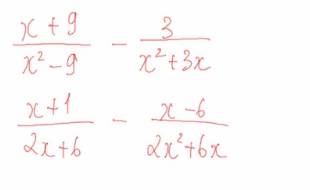
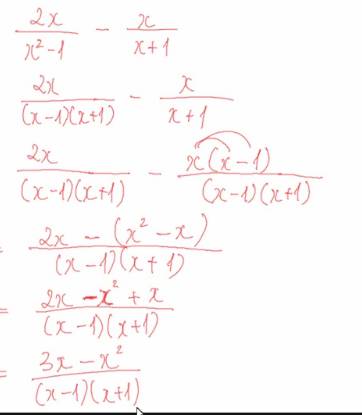
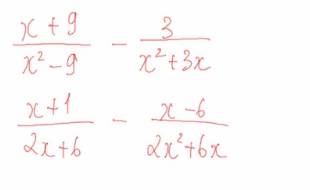
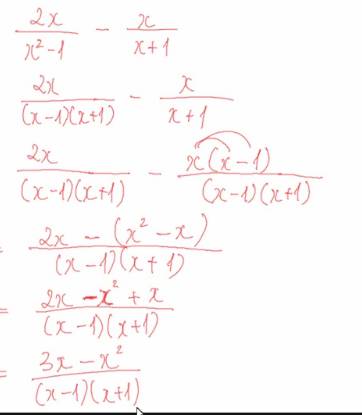
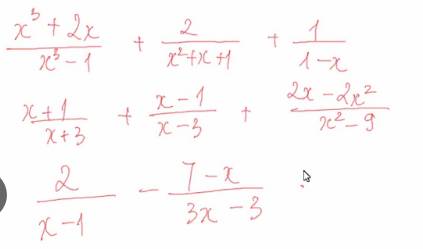
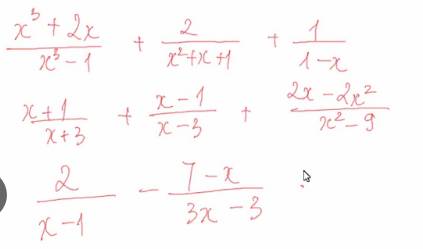
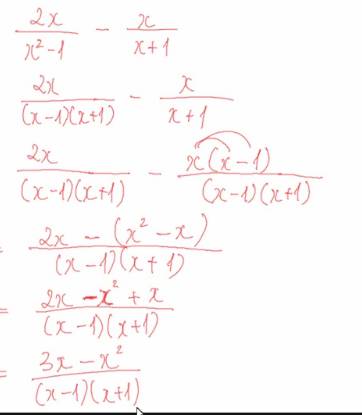
ừ
Big => large
Beat => best
bài mấy