 Giúp em vs.Em cần gấp.Cảm ơn trc ạ
Giúp em vs.Em cần gấp.Cảm ơn trc ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Ta có: ΔABC đều
mà BP,CM là các đường trung tuyến
nên BP,CM là các đường cao
Xét tứ giác BMPC có
\(\widehat{BMC}=\widehat{BPC}=90^0\)
nên BMPC là tứ giác nội tiếp
hay B,M,P,C cùng thuộc 1 đường tròn

a,\(P\left(x\right)=x^3-2x^4+x^5-\dfrac{1}{2}x^2+=x^5-2x^4+x^3-\dfrac{1}{2}x^2+1\)
bậc :5
b,\(Q\left(x\right)=-x^3+3x^2-5x^4-x^2+3x^3-\dfrac{1}{2}=-5x^4+2x^3+2x^2-\dfrac{1}{2}\)
bậc :4
b,\(P\left(x\right)-Q\left(x\right)=\left(x^5-2x^4+x^3-\dfrac{1}{2}x^2+1\right)-\left(-5x^4+2x^3+2x^2-\dfrac{1}{2}\right)\)
\(=\text{}\text{}\text{}\text{}x^5+3x^4-x^3-\dfrac{5}{2}x^2+\dfrac{3}{2}\)

b.
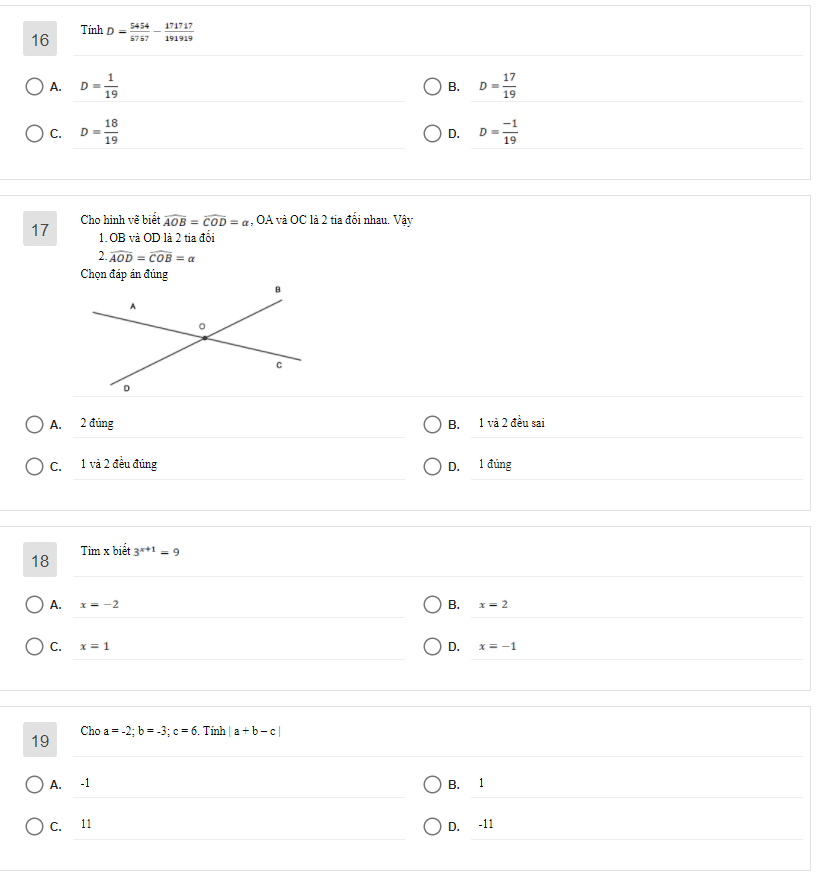
\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x< 2\\x>\dfrac{9}{2}\end{matrix}\right.\\-\dfrac{1}{3}< x< 7\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}-\dfrac{1}{3}< x< 2\\\dfrac{9}{2}< x< 7\end{matrix}\right.\)
Hay \(S=\left(-\dfrac{1}{3};2\right);\left(\dfrac{9}{2};7\right)\)
d.
\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x\le-\dfrac{11}{5}\\x\ge7\end{matrix}\right.\\-\dfrac{1}{2}< x< 3\end{matrix}\right.\) \(\Rightarrow x\in\varnothing\) hay BPT vô nghiệm

a, Theo tc 2 tt cắt nhau: \(AE=EC;BF=CF\)
Vậy \(AE+BF=EC+CF=EF\)
b, Vì \(\left\{{}\begin{matrix}AE=EC\\\widehat{EAO}=\widehat{ECO}=90^0\\OE.chung\end{matrix}\right.\) nên \(\Delta AOE=\Delta COE\)
\(\Rightarrow\widehat{AOE}=\widehat{EOC}\) hay OE là p/g \(\widehat{AOC}\)
Cmtt: \(\Delta BOF=\Delta COF\Rightarrow\widehat{BOF}=\widehat{COF}\) hay OF là p/g \(\widehat{BOC}\)
Vậy \(\widehat{EOF}=\widehat{COF}+\widehat{COE}=\dfrac{1}{2}\left(\widehat{AOC}+\widehat{BOC}\right)=90^0\) hay OE⊥OF

a: Xét ΔHAO vuông tại A và ΔHIO vuông tại I có
OH chung
góc AOH=góc IOH
=>ΔHAO=ΔHIO
b: H là tâm đường tròn nội tiếp ΔKOB
=>d(H,BK)=HA=4cm

Bài 6:
\(\Leftrightarrow6n+4⋮2n-1\)
\(\Leftrightarrow2n-1\in\left\{1;-1;7;-7\right\}\)
hay \(n\in\left\{1;0;4;-3\right\}\)


nó bị lỗi nên mình làm câu khác rồi
câu hỏi đây nè bạn
Tìm GTLN của \(A=\dfrac{\left(x+2\right)^2}{2}\times\left(1-\dfrac{x^2}{x+2}\right)-\dfrac{x^2+6x+4}{x}\)
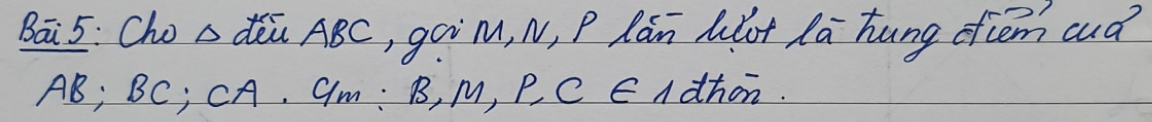


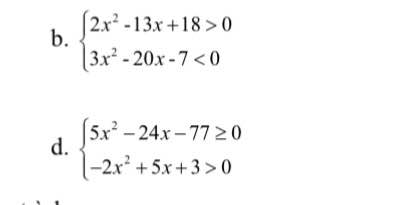
 Vẽ hình giúp mình với,mình cần gấp.
Vẽ hình giúp mình với,mình cần gấp.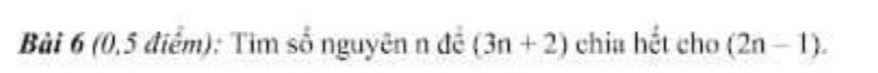 mn giúp em với ạ, em đang cần gấp lắm ạ
mn giúp em với ạ, em đang cần gấp lắm ạ
Bài 1:
\(a,VT=\dfrac{3x-1}{\left(x+2\right)\left(3x-1\right)}=\dfrac{1}{x+2}=VP\\ b,VT=\dfrac{x^2+2x+4}{\left(x-2\right)\left(x^2+2x+4\right)}=\dfrac{1}{x-2}\\ VP=\dfrac{x+3}{\left(x+3\right)\left(x-2\right)}=\dfrac{1}{x-2}\\ \Rightarrow VT=VP\\ c,VT=\dfrac{\left(x-2\right)\left(x^2+2x+4\right)}{2\left(x-2\right)}=\dfrac{x^2+2x+4}{2}=VP\)
Bài 2:
\(a,A=\dfrac{\left(x-4\right)\left(x+4\right)}{x-4}=x+4=2019+4=2023\\ b,2x-1=0\Leftrightarrow x=\dfrac{1}{2}\\ B=\dfrac{2x\left(x-1\right)}{\left(x-1\right)\left(x-2\right)}=\dfrac{2x}{x-2}=\dfrac{2\cdot\dfrac{1}{2}}{\dfrac{1}{2}-2}=\dfrac{1}{-\dfrac{3}{2}}=-\dfrac{2}{3}\\ c,x^2-9=0\Leftrightarrow\left[{}\begin{matrix}x=3\left(ktm\right)\\x=-3\left(tm\right)\end{matrix}\right.\Leftrightarrow x=-3\\ P=\dfrac{x-3}{\left(x-3\right)\left(x-2\right)}=\dfrac{1}{x-2}=\dfrac{1}{-3-2}=-\dfrac{1}{5}\)
Bài 3:
\(a,A=\dfrac{2\left(2x-3\right)}{2x^2-7x+6}=\dfrac{2\left(2x-3\right)}{\left(x-2\right)\left(2x-3\right)}=\dfrac{2}{x-2}\\ b,A=\dfrac{\left(x^2+2x\right)\left(2x^2-3x-2\right)}{x^2-2x}=\dfrac{x\left(x+2\right)\left(x-2\right)\left(2x+1\right)}{x\left(x-2\right)}=\left(x+2\right)\left(2x+1\right)\)