(giải phương trình)
x2 + 3x - 4 = 0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
thanks
PT
1
PT
1
HT
1
NV
Nguyễn Việt Lâm
Giáo viên
26 tháng 3 2022
\(f\left(x\right)=\dfrac{\left(3x-4\right)\left(2x-3\right)}{\left(x^2-5x+6\right)\left(5-x\right)}>0\)
\(\Leftrightarrow\dfrac{\left(3x-4\right)\left(2x-3\right)}{\left(x-2\right)\left(x-3\right)\left(5-x\right)}>0\)
Bảng xét dấu:
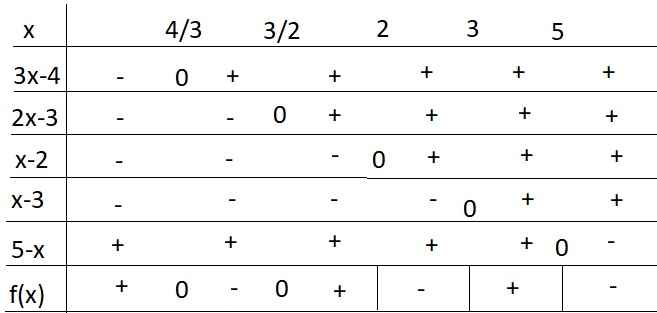
Từ bảng xét dấu ta thấy nghiệm của BPT là: \(\left[{}\begin{matrix}x< 5\\\dfrac{3}{2}< x< 2\\3< x< 5\end{matrix}\right.\)
PT
1
CM
25 tháng 6 2017
a) 2 x 2 - 3x + 1 = 0
a = 2; b = - 3; c = 1 ⇒ a + b + c = 0
Do đó phương trình có nghiệm x 1 = 1; x 2 = 1/2
\(x^2+3x-4=0\Leftrightarrow x^2-x+4x-4=0\)
\(\Leftrightarrow x.\left(x-1\right)+4.\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right).\left(x+4\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-1=0\\x+4=0\end{cases}\Leftrightarrow}\orbr{\begin{cases}x=1\\x=-4\end{cases}}\)
Vậy phương trình có nghiệm \(x=\left\{1,-4\right\}\)