20192 - 20182 + 20172 - 20162 + ... + 32 - 22 + 12
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
C
3
H
3 tháng 7 2021
a) \(153^2-53^2=\left(153-53\right)\left(153+53\right)=100.206=20600\)
b)
\(\left(2020^2-2019^2\right)+\left(2018^2-2017^2\right)+...+\left(2^2-1^2\right)\\ =\left(2020+2019\right)\left(2020-2019\right)+\left(2018+2017\right)\left(2018-2017\right)+...+\left(2+1\right)\left(2-1\right)\\ =2020+2019+2018+2017+...+2+1\\ =\dfrac{\left(2020+1\right)2020}{2}=2041210\)
AH
Akai Haruma
Giáo viên
3 tháng 7 2021
Lời giải:
a. $153^2-53^2=(153-53)(153+53)=100.206=20600$
b.
$2020^2-2019^2+2018^2-2017^2+...+2^2-1^2$
$=(2020^2-2019^2)+(2018^2-2017^2)+...+(2^2-1^2)$
$=(2020-2019)(2020+2019)+(2018-2017)(2018+2017)+...+(2-1)(2+1)$
$=2020+2019+2018+2017+...+2+1$
$=\frac{2020.2021}{2}=2041210$
AH
Akai Haruma
Giáo viên
23 tháng 6 2023
1.
$=153^2+2.47.153+47^2=(153+47)^2=200^2=40000$
2.
$=1,24^2-2.1,24.0,24+0,24^2=(1,24-0,24)^2=1^2=1$
3. Không phù hợp để tính nhanh
4.
$=15^8-(15^8-1)=1$
5.
$=(1^2-2^2)+(3^2-4^2)+(5^2-6^2)+...+(2019^2-2020^2)$
$=(1-2)(1+2)+(3-4)(3+4)+(5-6)(5+6)+...+(2019-2020)(2019+2020)$
$=(-1)(1+2)+(-1)(3+4)+(-1)(5+6)+....+(-1)(2019+2020)$
$=(-1)(1+2+3+4+....+2019+2020)=(-1).2020(2020+1):2=-2041210$
DT
Dang Tung
CTVHS
23 tháng 6 2023
6:
\(\left(2+1\right)\left(2^2+1\right)\left(2^4+1\right)....\left(2^{2020}+1\right)+1\\ =1.\left(2+1\right)\left(2^2+1\right)\left(2^4+1\right)....\left(2^{2020}+1\right)+1\\ =\left(2-1\right)\left(2+1\right)\left(2^2+1\right)\left(2^4+1\right)....\left(2^{2020}+1\right)+1\\ =\left(2^2-1\right)\left(2^2+1\right)\left(2^4+1\right)....\left(2^{2020}+1\right)+1\\ =\left(2^4-1\right)\left(2^4+1\right)....\left(2^{2020}+1\right)+1\\ =\left(2^8-1\right)....\left(2^{2020}+1\right)+1\\ =\left(2^{2020}-1\right)\left(2^{2020}+1\right)+1\\ =2^{4040}-1+1=2^{4040}\)
CM
21 tháng 9 2018
Đáp án D
Ta có 1 2 + 2 2 + 3 2 + ... + n 2 = n n + 1 2 n + 1 6
và 1 + 2 + 3 + ... + n 2 = n n + 1 2
Xét 1 + x 1 + 2 x ... 1 + n x ⇒ Hệ số của x 2 là
a 2 = 1. 2 + 3 + ... + n + 2. 3 + 4 + ... + n + ... + n − 1 n
= 1. 1 + 2 + ... + n − 1 + 2. 1 + 2 + ... + n − 1 + 2 + ... + n − 1 . 1 + 2 + ... + n − 1 + 2 + ... + n − 1
= ∑ k = 1 n k × n n + 1 2 − k k + 1 2
= 1 2 ∑ k = 1 n k × n 2 + n − k 2 + k
= 1 2 ∑ k = 1 n n 2 + n k − k 3 + k 2
= 1 2 = n 2 + n 2 8 − n n + 1 2 n + 1 12
n 2 + n 2 2 − n 2 + n 2 4 − n n + 1 2 n + 1 6
Vậy T = n 2 + n 2 8
→ n − 2017 T = 2017.2018 2 8 = 1 2 2017.2018 2 2
26 tháng 7 2023
a, $5^{3} =5\times5\times5=125$
$3^{5} =3\times3\times3=27$
$125>27=>5^{3}>3^{5}$
$3^{2}=3\times3=9$
$2^{3}=2\times2\times2=8$
$9>8=>3^{2}>2^{3}$
$2^{6} =2\times2\times2\times2\times2\times2=64$
$6^{2}=6\times6=36$
$64>36=>2^{6}>6^{2}$
b, $2015\times2017=2015\times(2016+1)=2015\times2016+2015$
$2016^{2}=2016\times2016=2016\times(2015+1)=2016\times2015+2016$
$2015\times2016+2015<2016\times2015+2016=>2015\times2017<2016^{2}$
c, $199^{20}=199^{4\times5}=(199^{4})^{5}= 1568239201^{5}$
$2003^{15}=2003^{3\times5}=(2003^{3})^5 =8036054027^{5}$
$1568239201<8036054027=>199^{20}<2003^{15}$
d, $3^99 =3^{3\times33}=(3^{3})^{33}=27^{33}>27^{21}$
$11^{21}<27^{21}=>3^{99}>11^{21}$
$3^{2n}=9^n$
$2^{3n}=8^n$
$9>8=>3^{2n}>2^{3n}$
LM
Lê Minh Vũ
CTVHS
26 tháng 7 2023
So sánh các số sau
a) 53 và 35
53 = 125
35 = 243
=> 53 < 35
32 và 23
32 = 9
23 = 8
=> 32 > 23
26 và 62
26 = 64
62 = 36
=> 26 > 62
b) 2015 x 2017 và 20162
2015 x 2017
= 2015 x ( 2016 + 1 )
= 2015 x 2016 + 2015
20162
= 2016 x 2016
= 2016 x ( 2015 + 1 )
= 2016 x 2015 + 2016
Vì: 2015 < 2016
=> 2015 x 2017 < 20162
c) 19920 và 200315
19920 < 20020 = ( 23 x 52 )20 = 260 x 540
200315 > 200015 = ( 2 x 103 )15 = ( 24 x 53 )15 = 260 x 545
=> 200315 > 19920
d) 399 và 1121
399 = ( 33 )33 = 2733 > 2721
Vì: 27 > 11
=> 2721 > 1121
=> 399 > 1121
32n và 23n
32n = ( 32 )n = 9n
23n = ( 23 )n = 8n
Vì 9 > 8
=> 9n > 8n
=> 32n > 23n
Vậy 32n > 23n
CM
26 tháng 1 2018
Ta có 12 + 22 + 32 + …102 = 385
Suy ra ( 12 +22 + 32 +…+102 ) .32 = 385.32
Do đó ta tính được A = 32 + 62 + 92 + …+302 = 3465
HA
2
21 tháng 6 2017
12=9+19+2=42
28+12+22+18=80
79+1+71+9=160
32+22+32+22=108
Chúc bn hok giỏi
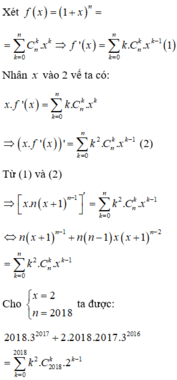
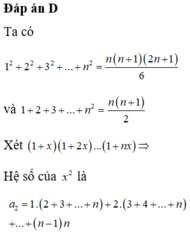
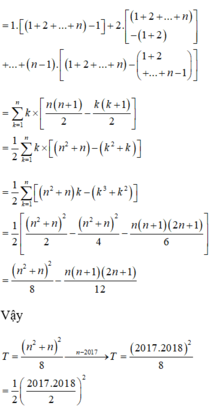
= (2019 - 2018)(2019 + 2018) + (2017 - 2016)(2017 + 2016) + ... + (3-2)(3+2) + 1
= 4037 + 4033 + ... + 5 + 1
= \(\left[\frac{4037-1}{4}+1\right]\left(4037+1\right):2\) =2039190