tan2x+cot2x+2cot2x=2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
16 tháng 10 2023
a: \(VT=\dfrac{cot^2x}{1+cot^2x}\cdot\dfrac{1+tan^2x}{tan^2x}\)
\(=\dfrac{cot^2x}{\dfrac{1}{sin^2x}}\cdot\dfrac{\dfrac{1}{cos^2x}}{tan^2x}\)
\(=\dfrac{cot^2x}{tan^2x}\cdot\dfrac{1}{cos^2x}:\dfrac{1}{sin^2x}\)
\(=\dfrac{cot^2x}{tan^2x}\cdot\dfrac{sin^2x}{cos^2x}\)
\(=cot^2x\)
\(VP=\dfrac{tan^2x+cot^2x}{1+tan^4x}=\dfrac{\dfrac{sin^2x}{cos^2x}+\dfrac{cos^2x}{sin^2x}}{1+\dfrac{sin^4x}{cos^4x}}\)
\(=\dfrac{sin^4x+cos^4x}{sin^2x\cdot cos^2x}:\dfrac{cos^4x+sin^4x}{cos^4x}\)
\(=\dfrac{sin^4x+cos^4x}{sin^2x\cdot cos^2x}\cdot\dfrac{cos^4x}{cos^4x+sin^4x}=\dfrac{cos^2x}{sin^2x}=cot^2x\)
=>VT=VP
b:
\(\dfrac{tan^2x-cos^2x}{sin^2x}+\dfrac{cot^2x-sin^2x}{cos^2x}\)
\(=\dfrac{\left(\dfrac{sinx}{cosx}\right)^2-cos^2x}{sin^2x}+\dfrac{\left(\dfrac{cosx}{sinx}\right)^2-sin^2x}{cos^2x}\)
\(=\dfrac{sin^2x-cos^4x}{cos^2x\cdot sin^2x}+\dfrac{cos^2x-sin^4x}{sin^2x\cdot cos^2x}\)
\(=\dfrac{sin^2x+cos^2x-cos^4x-sin^4x}{cos^2x\cdot sin^2x}\)
\(=\dfrac{1-\left(cos^2x+sin^2x\right)^2+2\cdot cos^2x\cdot sin^2x}{cos^2x\cdot sin^2x}\)
\(=\dfrac{2\cdot cos^2x\cdot sin^2x}{cos^2x\cdot sin^2x}=2\)
LN
0
2
1
NV
Nguyễn Việt Lâm
Giáo viên
10 tháng 4 2019
\(\frac{2}{sin4x}-tan2x=\frac{2}{2sin2x.cos2x}-\frac{sin2x}{cos2x}=\frac{1}{cos2x}\left(\frac{1}{sin2x}-sin2x\right)\)
\(=\frac{1}{cos2x}\left(\frac{1-sin^22x}{sin2x}\right)=\frac{1}{cos2x}\frac{cos^22x}{sin2x}=\frac{cos2x}{sin2x}=cot2x\)
CM
14 tháng 10 2018
Chọn D
Vậy trong khoảng (0,2π), phương trình có các nghiệm là π 4 ; 3 π 4 ; 5 π 4 ; 7 π 4 nên tổng các nghiệm là 4π
27 tháng 6 2021
\(a,\tan10.\tan11......\)
\(=\left(\tan10.tan80\right)\left(tan11.tan79\right)....\left(tan44.tan46\right).tan45\)
Mà 10 và 80, 11 và 79, ... là các góc phụ nhau .
\(=tan10.cot10....tan45=1\)
b, Ta có : \(\tan x+\cot x=2\)
\(\Rightarrow\tan^2x+2\tan x\cot x+\cot^2x=4\)
\(\Rightarrow\tan^2x+\cot^2x=4-2=2\)
Ta có : \(\tan^3x+\cot^3x=\left(\tan x+\cot x\right)\left(\tan^2x-\tan x\cot x+\cot^2x\right)=2\)
NT
0
NV
Nguyễn Việt Lâm
Giáo viên
16 tháng 4 2019
a/
\(\left(\frac{sin2x}{cos2x}-\frac{sinx}{cosx}\right)cos2x=\left(\frac{sin2x.cosx-cos2x.sinx}{cos2x.cosx}\right).cos2x\)
\(=\frac{sin\left(2x-x\right)}{cosx}=\frac{sinx}{cosx}=tanx\)
b/
\(2\left(1-sinx\right)\left(1+cosx\right)=2+2cosx-2sinx-2sinxcosx\)
\(=1+sin^2x+cos^2x-2sinx+2cosx-2sinx.cosx\)
\(=\left(1-sinx+cosx\right)^2\)
c/
\(1+cotx+cot^2x+cot^3x=1+cotx+cot^2x\left(1+cotx\right)\)
\(=\left(1+cotx\right)\left(1+cot^2x\right)=\left(1+\frac{cosx}{sinx}\right)\left(1+\frac{cos^2x}{sin^2x}\right)=\frac{sinx+cosx}{sin^3x}\)
d/
\(\frac{cos3x}{sinx}+\frac{sin3x}{cosx}=\frac{cos3x.cosx+sin3x.sinx}{sinx.cosx}=\frac{cos\left(3x-x\right)}{\frac{1}{2}2sinx.cosx}=\frac{2cos2x}{sin2x}=2cot2x\)
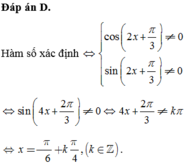
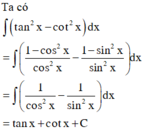
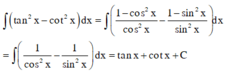
\(\Leftrightarrow tan^2x-2+cot^2x+\frac{2}{tan2x}=0\)
\(\Leftrightarrow\left(tanx-cotx\right)^2+\frac{1-tan^2x}{tanx}=0\)
\(\Leftrightarrow\left(\frac{1-tan^2x}{tanx}\right)^2+\frac{1-tan^2x}{tanx}=0\)
\(\Leftrightarrow t^2+t=0\)