Tìm x biết
x(x - 3) - 5x + 15 = 0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
AN
4
30 tháng 1 2022
\(x=\dfrac{7}{25}+\dfrac{-1}{5}=\dfrac{7}{25}-\dfrac{1}{5}=\dfrac{2}{25}.\\ x=\dfrac{5}{11}+\dfrac{4}{-9}=\dfrac{5}{11}-\dfrac{4}{9}=\dfrac{1}{99}.\\ \dfrac{5}{9}-\dfrac{x}{-1}=\dfrac{-1}{3}\Leftrightarrow\dfrac{5}{9}+x=-\dfrac{1}{3}.\Leftrightarrow x=-\dfrac{8}{9}.\)
N
30 tháng 1 2022
\(x=\dfrac{7}{25}+-\dfrac{1}{5}=>\dfrac{7}{25}+-\dfrac{5}{25}=>x=\dfrac{2}{25}\)
\(x=\dfrac{5}{11}+\dfrac{4}{-9}=>\dfrac{-45}{-99}+\dfrac{44}{-99}=>x=\dfrac{-1}{-99}=\dfrac{1}{99}\)
\(\dfrac{5}{9}-\dfrac{x}{-1}=-\dfrac{1}{3}=>-\dfrac{1}{3}-\dfrac{5}{9}=>\dfrac{x}{-1}=-\dfrac{8}{9}=>x=-\dfrac{8}{9}\)
DT
19 tháng 3 2022
\(x=\dfrac{15}{13}+\dfrac{11}{13}=\dfrac{15+11}{13}=\dfrac{26}{13}\)
N
0
N
0
DT
Dang Tung
CTVHS
17 tháng 12 2023
b) \(\left(x+3\right)^2-5x-15=0\\ \Leftrightarrow\left(x+3\right)^2-5\left(x+3\right)=0\\ \Leftrightarrow\left(x+3\right)\left(x+3-5\right)=0\\ \Rightarrow\left[{}\begin{matrix}x+3=0\\x-2=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-3\\x=2\end{matrix}\right.\)
Vậy tập nghiệm của phương trình là : \(S=\left\{-3;2\right\}\)
c) \(2x^5-4x^3+2x=0\\ \Leftrightarrow2x\left(x^4-2x^2+1\right)=0\\ \Leftrightarrow2x\left(x^2-1\right)^2=0\\ \Rightarrow\left[{}\begin{matrix}2x=0\\\left(x^2-1\right)^2=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x^2=1\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\\x=-1\end{matrix}\right.\)
Vậy tập nghiệm của pt là : \(S=\left\{0;1;-1\right\}\)
LV
0
DN
2
22 tháng 6 2021
\(3xy-2y+6x=0\)
\(\Leftrightarrow3xy+6x-2y-4+4=0\)
\(\Leftrightarrow3x\left(y+2\right)-2\left(y+2\right)+4=0\)
\(\Leftrightarrow\left(y+2\right)\left(3x-2\right)=-4\)
Vì x,y là các số nguyên nên y+2 và 3x-2 cũng là các số nguyên
\(\Leftrightarrow\left(y+2\right)\left(3x-2\right)=1.\left(-4\right)=\left(-1\right).4\)
Ta có bảng sau:
| y+2 | -1 | 4 | -4 | 1 |
| y | -3 | 2 | -6 | -1 |
| 3x-2 | 4 | -1 | 1 | -4 |
| 3x | 6 | 1 | 3 | -2 |
| x | 2 | \(\dfrac{1}{3}\)(loại) | 1 | \(\dfrac{-2}{3}\)(loại) |
TH1: \(y=-3\) ;\(x=2\) thì \(x+y=2+\left(-3\right)=-1\)
TH2: \(y=-6;x=1\) thì \(x+y=-6+1=-5\)
Vậy \(x+y=-1\) khi \(y=-3\) và \(x=2\)
\(x+y=-5\) khi \(y=-6;x=1\)
22 tháng 6 2021
Giải:
Ta có:
\(3xy-2y+6x=0\)
\(\Rightarrow3x.\left(y+2\right)-2y-4=-4\)
\(\Rightarrow3x.\left(y+2\right)-2.\left(y+2\right)=-4\)
\(\Rightarrow\left(3x-2\right).\left(y+2\right)=-4\)
\(\Rightarrow\left(3x-2\right)\) và \(\left(y+2\right)\inƯ\left(-4\right)=\left\{\pm1;\pm2;\pm4\right\}\)
Ta có bảng giá trị:
| 3x-2 | -4 | -2 | -1 | 1 | 2 | 4 |
| y+2 | 1 | 2 | 4 | -4 | -2 | -1 |
| x | \(\dfrac{-2}{3}\) (loại) | 0 (t/m) | \(\dfrac{1}{3}\) (loại) | 1 (t/m) | \(\dfrac{4}{3}\) (loại) | 2 (t/m) |
| y | -1 | 0 | 2 | -6 | -4 | -3 |
Vậy \(\left(x;y\right)=\left\{\left(0;0\right);\left(1;-6\right);\left(2;-3\right)\right\}\)
\(\left(+\right)TH1:x+y=0+0=0\)
\(\left(+\right)TH2:x+y=1+-6=-5\)
\(\left(+\right)TH3:x+y=2+-3=-1\)
Chúc bạn học tốt!
PH
4
8 tháng 9 2021
\(\dfrac{x}{3}=x+y=20\Rightarrow x=60\Rightarrow60+y=20\Rightarrow y=-40\)
NH
2
12 tháng 8 2023
\(\left(x-3\right)=\left(3-x\right)^2\)
\(\Leftrightarrow x-3=\left(x-3\right)^2\)
\(\Leftrightarrow\left(x-3\right)-\left(x-3\right)^2=0\)
\(\Leftrightarrow\left(x-3\right)\left[1-\left(x-3\right)\right]=0\)
\(\Leftrightarrow\left(x-3\right)\left(4-x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=0\\4-x=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=4\end{matrix}\right.\)
___________
\(x^3+\dfrac{3}{2}x^2+\dfrac{3}{4}x+\dfrac{1}{8}=\dfrac{1}{64}\)
\(\Leftrightarrow x^3+3\cdot\dfrac{1}{2}\cdot x^2+3\cdot\left(\dfrac{1}{2}\right)^2\cdot x+\left(\dfrac{1}{2}\right)^3=\dfrac{1}{64}\)
\(\Leftrightarrow\left(x+\dfrac{1}{2}\right)^3=\left(\dfrac{1}{4}\right)^3\)
\(\Leftrightarrow x+\dfrac{1}{2}=\dfrac{1}{4}\)
\(\Leftrightarrow x=\dfrac{1}{4}-\dfrac{1}{2}\)
\(\Leftrightarrow x=-\dfrac{1}{4}\)
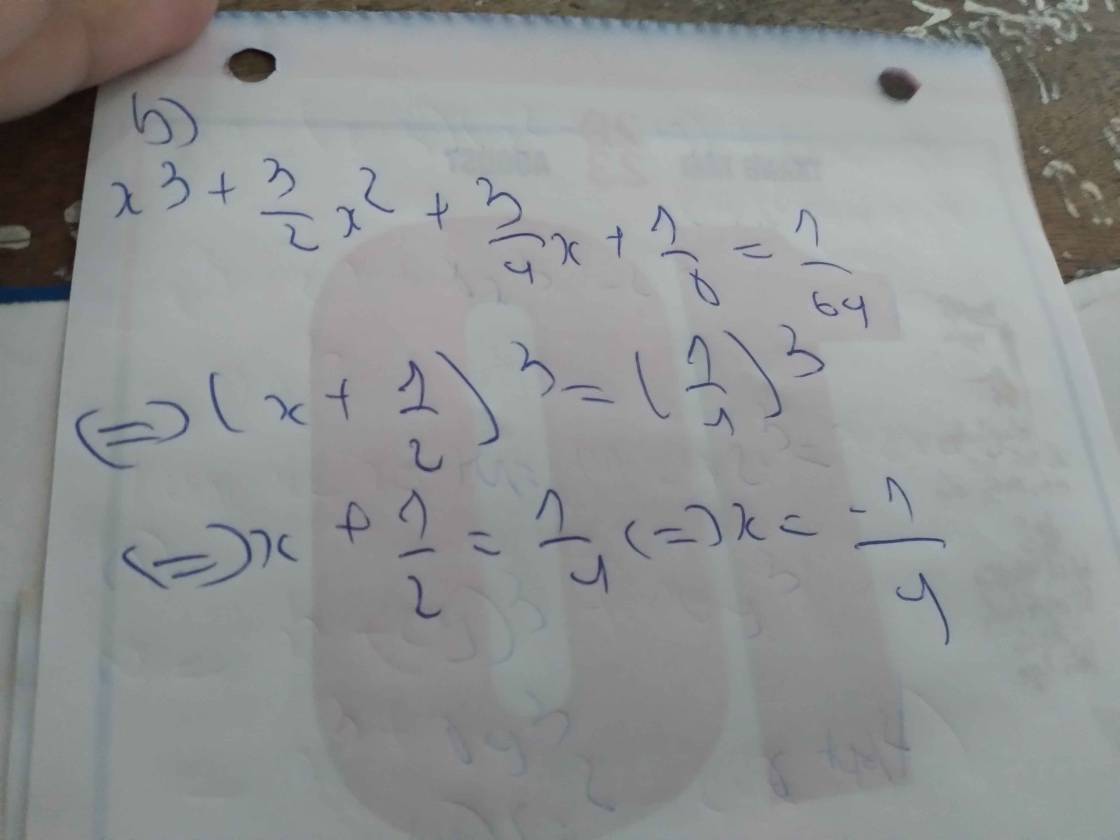
\(x\left(x-3\right)-5x+15=0\)
\(\Rightarrow x\left(x-3\right)-\left(5x-15\right)=0\)
\(\Rightarrow x\left(x-3\right)-5\left(x-3\right)=0\)
\(\Rightarrow\left(x-5\right)\left(x-3\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x-5=0\\x-3=0\end{cases}}\Rightarrow\orbr{\begin{cases}x=5\\x=3\end{cases}}\)