sin2x.tan2x+4sin2x-tan2x+3cos2x=3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
8 tháng 10 2023
1:
a: ĐKXĐ: \(x< >\dfrac{\Omega}{2}+k\Omega\)
=>TXĐ: \(D=R\backslash\left\{\dfrac{\Omega}{2}+k\Omega\right\}\)
b: ĐKXĐ: \(x< >k\Omega\)
=>TXĐ: \(D=R\backslash\left\{k\Omega\right\}\)
c: ĐKXĐ: \(2x< >\dfrac{\Omega}{2}+k\Omega\)
=>\(x< >\dfrac{\Omega}{4}+\dfrac{k\Omega}{2}\)
TXĐ: \(D=R\backslash\left\{\dfrac{\Omega}{4}+\dfrac{k\Omega}{2}\right\}\)
d: ĐKXĐ: \(3x< >\Omega\cdot k\)
=>\(x< >\dfrac{k\Omega}{3}\)
TXĐ: \(D=R\backslash\left\{\dfrac{k\Omega}{3}\right\}\)
e: ĐKXĐ: \(x+\dfrac{\Omega}{3}< >\dfrac{\Omega}{2}+k\Omega\)
=>\(x< >\dfrac{\Omega}{6}+k\Omega\)
TXĐ: \(D=R\backslash\left\{\dfrac{\Omega}{6}+k\Omega\right\}\)
f: ĐKXĐ: \(x-\dfrac{\Omega}{6}< >\Omega\cdot k\)
=>\(x< >k\Omega+\dfrac{\Omega}{6}\)
TXĐ: \(D=R\backslash\left\{k\Omega+\dfrac{\Omega}{6}\right\}\)
CM
1 tháng 1 2017
Chọn C.
Từ giả thiết ta suy ra:
B = tan2x (sin2x - 1) + sin2 x = -tan2x.cos2x + sin2x
MA
1
30 tháng 6 2021
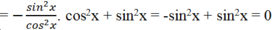
Pt\(\Leftrightarrow3\left(cos^2x-sin^2x\right)-8.sinx.cosx=sin^2x+cos^2x\)
\(\Leftrightarrow2cos^2x-8sinx.cosx-4sin^2x=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=\left(2+\sqrt{6}\right)sinx\\cosx=\left(2-\sqrt{6}\right)sinx\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx=\dfrac{1}{2+\sqrt{6}}\\tanx=\dfrac{1}{2-\sqrt{6}}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=arc.tan\left(\dfrac{1}{2+\sqrt{6}}\right)+k\pi\\x=arc.tan\left(\dfrac{1}{2-\sqrt{6}}\right)+k\pi\end{matrix}\right.\), k nguyên
Vậy...
NV
Nguyễn Việt Lâm
Giáo viên
10 tháng 10 2020
\(\Leftrightarrow\frac{4sin2x+cos2x+17}{3cos2x+sin2x+m+1}-2\ge0\) (tất nhiên là với mọi x)
\(\Leftrightarrow\frac{2sin2x-5cos2x-2m+15}{3cos2x+sin2x+m+1}\ge0\)
TH1: \(\left\{{}\begin{matrix}2sin2x-5cos2x-2m+15\ge0\\3cos2x+sin2x+m+1>0\end{matrix}\right.\) ;\(\forall x\)
\(\Leftrightarrow\left\{{}\begin{matrix}\frac{2}{\sqrt{29}}sin2x-\frac{5}{\sqrt{29}}cos2x\ge\frac{2m-15}{\sqrt{29}}\\\frac{1}{\sqrt{10}}sin2x+\frac{3}{\sqrt{10}}cos2x>\frac{-m-1}{\sqrt{10}}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sin\left(2x-a\right)\ge\frac{2m-15}{\sqrt{29}}\\sin\left(2x+b\right)>\frac{-m-1}{\sqrt{10}}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\frac{2m-15}{\sqrt{29}}\le-1\\\frac{-m-1}{\sqrt{10}}< -1\end{matrix}\right.\) tới đây chắc bạn tự giải được
TH2: tương tự:
\(\left\{{}\begin{matrix}2sin2x-5cos2x-2m+15\le0\\3cos2x+sin2x+m+1< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\frac{2m-15}{\sqrt{29}}\ge1\\\frac{-m-1}{\sqrt{10}}>1\end{matrix}\right.\) \(\Leftrightarrow...\)
NV
Nguyễn Việt Lâm
Giáo viên
17 tháng 10 2019
a/ \(-1\le sin2x\le1\Rightarrow-7\le y\le-1\)
b/ \(-1\le cos2x\le1\Rightarrow1\le y\le7\)
c/ \(-1\le sin2x\le1\Rightarrow3\le y\le11\)
d/ \(-1\le cos\left(3x+\frac{\pi}{3}\right)\le1\Rightarrow8\le y\le12\)
NV
Nguyễn Việt Lâm
Giáo viên
7 tháng 11 2019
ĐKXĐ: ...
a/ \(\frac{sin2x}{cos2x}+\frac{cosx}{sinx}=8cos^2x\)
\(\Leftrightarrow sin2x.sinx+cos2x.cosx=8cos^2x.sinx.cos2x\)
\(\Leftrightarrow cosx=4sin2x.cos2x.cosx\)
\(\Leftrightarrow cosx=2sin4x.cosx\)
\(\Leftrightarrow cosx\left(2sin4x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\sin4x=\frac{1}{2}\end{matrix}\right.\) \(\Leftrightarrow...\)
b/ \(\frac{cosx}{sinx}-\frac{sinx}{cosx}+4sin2x=\frac{1}{sinx.cosx}\)
\(\Leftrightarrow cos^2x-sin^2x+4sin2x.sinx.cosx=1\)
\(\Leftrightarrow cos2x+2sin^22x=1\)
\(\Leftrightarrow cos2x+2\left(1-cos^22x\right)=1\)
\(\Leftrightarrow-2cos^22x+cos2x+1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=1\\cos2x=-\frac{1}{2}\end{matrix}\right.\) \(\Leftrightarrow...\)
NV
Nguyễn Việt Lâm
Giáo viên
7 tháng 11 2019
1c/
\(5sinx-2=3\left(1-sinx\right)\frac{sin^2x}{1-sin^2x}\)
\(\Leftrightarrow5sinx-2=\frac{3sin^2x}{1+sinx}\)
\(\Leftrightarrow\left(5sinx-2\right)\left(1+sinx\right)=3sin^2x\)
\(\Leftrightarrow5sin^2x+3sinx-2=3sin^2x\)
\(\Leftrightarrow2sin^2x+3sinx-2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=\frac{1}{2}\\sinx=-2\left(l\right)\end{matrix}\right.\) \(\Rightarrow x=...\)
Bài 2:
a/ \(\Leftrightarrow\frac{\left(m+1\right)\left(1-cos2x\right)}{2}-sin2x+cos2x=0\)
\(\Leftrightarrow2sin2x+\left(m-1\right)cos2x=m+1\)
Theo điều kiện có nghiệm của pt lượng giác bậc nhất:
\(4+\left(m-1\right)^2\ge\left(m+1\right)^2\)
\(\Leftrightarrow4m\le4\Rightarrow m\le1\)
PT
1
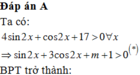
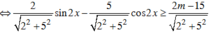
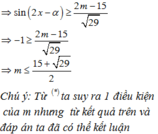
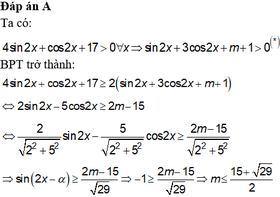
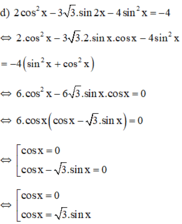
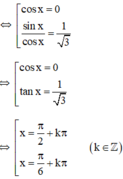
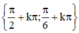(k ∈ Z)
đề bài đâu bạn, gpt hay Cm vậy?