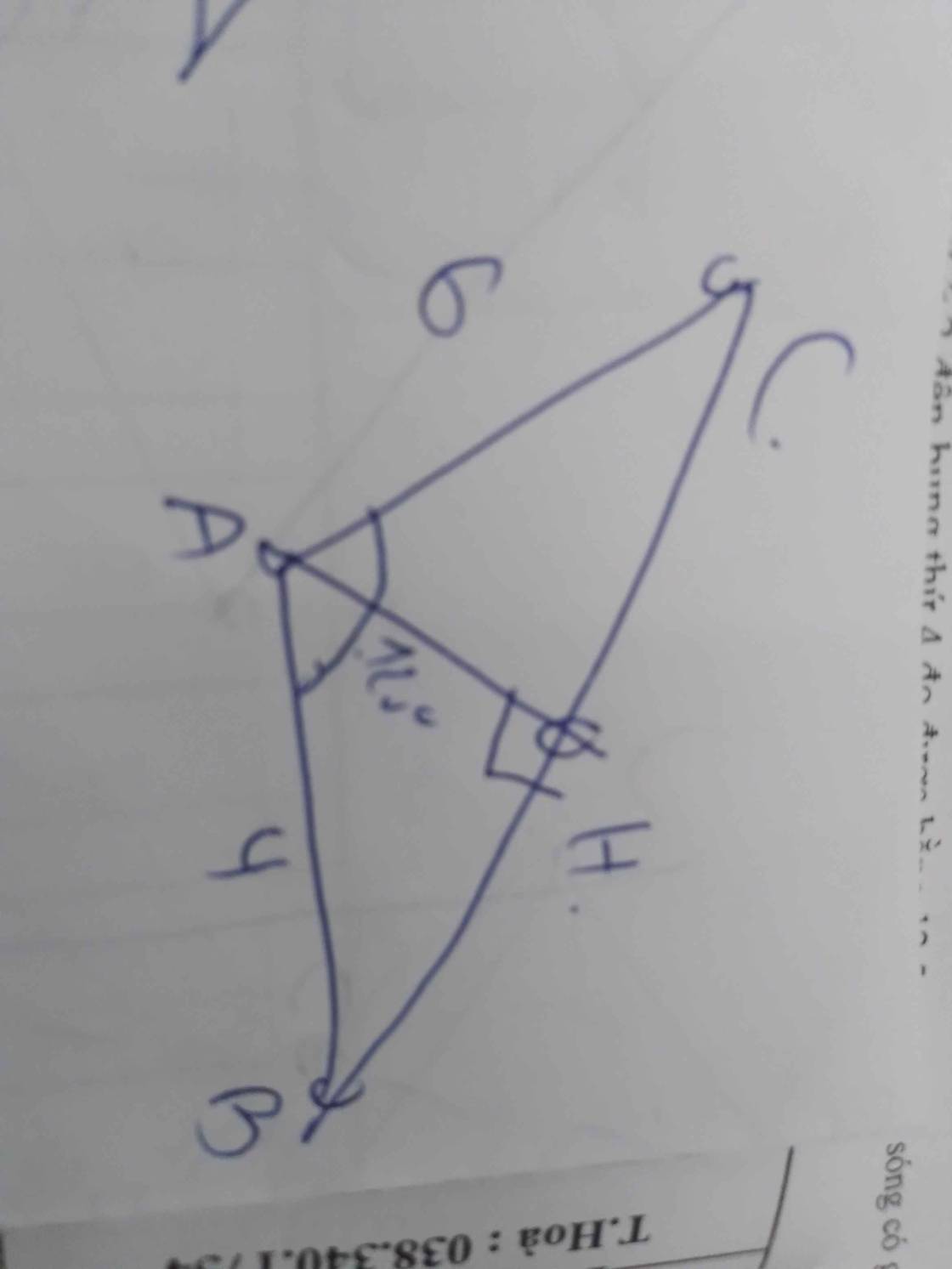
Cho tam giác abc biết a=6, c=8 , góc B=120 độ tính: b, S, r
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NH
1
1 tháng 12 2023
Diện tích tam giác ABC là:
\(S_{ABC}=\dfrac{1}{2}\cdot BA\cdot BC\cdot sinABC\)
\(=\dfrac{1}{2}\cdot5\cdot7\cdot sin120=\dfrac{35\sqrt{3}}{4}\)
Xét ΔABC có \(cosB=\dfrac{BA^2+BC^2-AC^2}{2\cdot BA\cdot BC}\)
=>\(\dfrac{5^2+7^2-AC^2}{2\cdot5\cdot7}=cos120=\dfrac{-1}{2}\)
=>\(25+49-AC^2=-35\)
=>\(AC^2=25+49+35=109\)
=>\(AC=\sqrt{109}\)
Kẻ AH\(\perp\)BC
=>\(h_A=AH\)
\(S_{ABC}=\dfrac{1}{2}\cdot AH\cdot BC\)
=>\(\dfrac{1}{2}\cdot AH\cdot7=\dfrac{35\sqrt{3}}{4}\)
=>\(AH\cdot3,5=\dfrac{35\sqrt{3}}{4}\)
=>\(AH=\dfrac{10\sqrt{3}}{4}=\dfrac{5}{2}\sqrt{3}\)
Xét ΔABC có \(\dfrac{AC}{sinB}=2R\)
=>\(2R=\dfrac{\sqrt{109}}{sin120}=\sqrt{109}\cdot\dfrac{2}{\sqrt{3}}\)
=>\(R=\sqrt{\dfrac{109}{3}}=\dfrac{\sqrt{327}}{3}\)
27 tháng 10 2023
a: Xét ΔABC có \(\widehat{A}+\widehat{B}+\widehat{C}=180^0\)
=>\(\widehat{C}=180^0-60^0-45^0=75^0\)
Xét ΔABC có \(\dfrac{BC}{sinA}=\dfrac{AC}{sinB}=\dfrac{AB}{sinC}\)
=>\(\dfrac{BC}{sin60}=\dfrac{4}{sin45}=\dfrac{AB}{sin75}\)
=>\(BC=2\sqrt{6};AB=2+2\sqrt{3}\)
b: Xét ΔABC có
\(\dfrac{BC}{sinA}=2R\)
=>\(2R=6:sin60=4\sqrt{3}\)
=>\(R=2\sqrt{3}\)
27 tháng 1 2016
Ta có: góc C = 70 độ
=> góc BCI = 35 độ
=> góc IBC = 25
=> góc B = 50 độ
=> góc A = 60 độ
Vậy tam giác ABC có góc A = 60 độ; góc B = 50 độ; góc C = 70 độ
PG
2
OI
1
13 tháng 10 2021
\(\widehat{B}=\widehat{E}=65^0\)
\(\widehat{C}=\widehat{F}=55^0\)
\(\widehat{A}=\widehat{D}=60^0\)