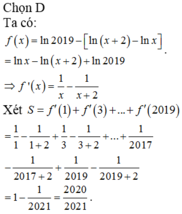Cho hàm số \(f\left(x\right)=ln2017-ln\dfrac{x+1}{x}\) . Tính tổng S= f'(1)+f'(2)+f'(3)+...+f'(2018)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
11 tháng 3 2022
\(F\left(x\right)=\int\left(e^x.ln\left(ax\right)+\dfrac{e^x}{x}\right)dx=\int e^xln\left(ax\right)dx+\int\dfrac{e^x}{x}dx=\int e^xlnxdx+\int\dfrac{e^x}{x}dx+\int e^x.lna.dx\)
Xét \(I=\int e^xlnxdx\)
Đặt \(\left\{{}\begin{matrix}u=lnx\\dv=e^xdx\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}du=\dfrac{dx}{x}\\v=e^x\end{matrix}\right.\)
\(\Rightarrow I=lnx.e^x-\int\dfrac{e^x}{x}dx\)
\(\Rightarrow F\left(x\right)=e^x.lnx+e^x.lna+C\)
\(F\left(\dfrac{1}{a}\right)=e^{\dfrac{1}{a}}ln\left(\dfrac{1}{a}\right)+e^{\dfrac{1}{a}}.lna+C=0\Rightarrow C=0\)
\(F\left(2020\right)=e^{2020}ln\left(2020\right)+e^{2020}.lna=e^{2020}\)
\(\Rightarrow ln\left(2020a\right)=1\Rightarrow a=\dfrac{e}{2020}\)
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 2 2021
Làm xuôi thì đơn giản, tính \(F'\left(x\right)\) là xong (chịu khó biến đổi)
Làm ngược thì nhìn biểu thức hơi thiếu thân thiện
\(\int\dfrac{2\sqrt{2}\left(x^2-1\right)}{x^4+1}dx=\int\dfrac{2\sqrt{2}\left(x^2-1\right)}{\left(x^2-x\sqrt{2}+1\right)\left(x^2+x\sqrt{2}+1\right)}dx\)
Phân tách hệ số bất định:
\(\dfrac{2\sqrt{2}\left(x^2-1\right)}{\left(x^2-x\sqrt{2}+1\right)\left(x^2+x\sqrt{2}+1\right)}=\dfrac{a\left(2x-\sqrt{2}\right)}{x^2-x\sqrt{2}+1}+\dfrac{b\left(2x+\sqrt{2}\right)}{x^2+x\sqrt{2}+1}\)
Quan tâm tử số: \(a\left(2x-\sqrt{2}\right)\left(x^2+x\sqrt{2}+1\right)+b\left(2x+\sqrt{2}\right)\left(x^2-x\sqrt{2}+1\right)\)
\(=2\left(a+b\right)x^3+\sqrt{2}\left(a-b\right)x^2+\sqrt{2}\left(b-a\right)\)
Đồng nhất 2 tử số: \(\left\{{}\begin{matrix}a+b=0\\a-b=2\\\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}a=1\\b=-1\end{matrix}\right.\)
Do đó:
\(\dfrac{2\sqrt{2}\left(x^2-1\right)}{x^4+1}=\dfrac{2x-\sqrt{2}}{x^2-x\sqrt{2}+1}-\dfrac{2x+\sqrt{2}}{x^2+x\sqrt{2}+1}\)
HT
21 tháng 2 2021
Cái tìm hệ số bất định ấy ạ, tại sao lại tách về 2x- căn 2 vậy anh?
CM
24 tháng 12 2018
Đáp án B
ta có f ' x = − x x + 1 − 1 x 2 = 1 x + 1 x = 1 x − 1 x + 1
⇒ S = 1 − 1 2 + 1 2 − 1 3 + 1 3 − ... + 1 2018 − 1 2019 = 2018 2019
KS
30 tháng 3 2022
Cho hàm số y=f(x)y=f(x) có đạo hàm và liên tục trên [0;π2][0;π2]thoả mãn f(x)=f′(x)−2cosxf(x)=f′(x)−2cosx. Biết f(π2)=1f(π2)=1, tính giá trị f(π3)f(π3)
A. √3+1/2 B. √3−1/2 C. 1−√3/2 D. 0
GV
Giáo viên Toán
Giáo viên
4 tháng 5 2017
Để kiểm tra một hàm F(x) có phải là một nguyên hàm của f(x) không thì ta chỉ cần kiểm tra F'(x) có bằng f(x) không?
a) \(F\left(x\right)\) là hằng số nên \(F'\left(x\right)=0\ne f\left(x\right)\)
b) \(G'\left(x\right)=2.\dfrac{1}{2}.\dfrac{1}{\cos^2x}=1+\tan^2x\)
c) \(H'\left(x\right)=\dfrac{\cos x}{1+\sin x}\)
d) \(K'\left(x\right)=-2.\dfrac{-\left(\dfrac{1}{2}.\dfrac{1}{\cos^2\dfrac{x}{2}}\right)}{\left(1+\tan\dfrac{x}{2}\right)^2}=\dfrac{\dfrac{1}{\cos^2\dfrac{x}{2}}}{\left(\dfrac{\cos\dfrac{x}{2}+\sin\dfrac{x}{2}}{\cos\dfrac{x}{2}}\right)^2}\)
\(=\dfrac{1}{\left(\cos\dfrac{x}{2}+\sin\dfrac{x}{2}\right)^2}=\dfrac{1}{1+2\cos\dfrac{x}{2}\sin\dfrac{x}{2}}\)
\(=\dfrac{1}{1+\sin x}\)
Vậy hàm số K(x) là một nguyên hàm của f(x).
