(4x2 - 7x - 50)2 - 16x4 - 56x3 - 49x2
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) Ta có: \(2x^2\left(3x^2-7x-5\right)\)
\(=2x^2\cdot3x^2-2x^2\cdot7x-2x^2\cdot5\)
\(=6x^4-14x^3-10x^2\)
c) Ta có: \(\left(16x^4-20x^2y^3-4x^5y\right):\left(-4x^2\right)\)
\(=16x^4:\left(-4x^2\right)+20x^2y^3:4x^2+4x^5y:4x^2\)
\(=-4x^3+5y^3+x^3y\)


a) 6x³ : (-3x²) = [6 : (-3)] . (x³ : x²)
= -2x
b) (-9x²) : 6x
= (-9 : 6) . (x² : x)
= -3/2 x
c) (-16x⁴) : (-12x³)
= [-16 : (-12)] . (x⁴ : x³)
= 4/3 x
d) (8x³ + 4x² - 6x) : 2x
= 8x³ : 2x + 4x² : 2x - 6x : 2x
= 4x² + 2x - 3

1)
`7x^2 -49x=0`
`<=>x(7x-49)=0`
\(< =>\left[{}\begin{matrix}x=0\\7x-49=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=0\\x=7\end{matrix}\right.\)
2)
`8x^2 -16x=0`
`<=>x(8x-16)=0`
\(< =>\left[{}\begin{matrix}x=0\\8x-16=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
3)
`2x^3 +40x=0`
`<=>x(2x^2 +40)=0`
`<=>x=0` hoặc`2x^2 +40=0`
`<=>x=0` hoặc `2x^2 =-40` (vô lí vì `2x^2` luôn lớn hơn hoặc bằng 0)
`<=>x=0`
4)
`-x^3 +16x=0`
`<=>x^3 -16x=0`
`<=>x(x^2 -16)=0`
\(< =>\left[{}\begin{matrix}x=0\\x^2-16=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=0\\x^2=16\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=0\\x=4\\x=-4\end{matrix}\right.\)

\(=\dfrac{\left(7x-y\right)\left(7x+y\right)}{7x-y}=7x+y\)

Đáp án là B.
Đặt t = x - 2 x Đạo hàm t , = 1 + 2 x 2 > 0
Do đó t ( 1 ) ≤ t ≤ t ( 2 ) , ∀ x ∈ [ 1 ; 2 ] , suy ra - 1 ≤ t ≤ 1
Ta có x 2 + 4 x 2 = t 2 + 4 , x 4 + 16 x 4 = ( x 2 + 4 x 2 ) 2 - 8 = ( t 2 + 4 ) 2 - 8 = t 4 + 8 t 2 + 8
Phương trình đã cho trở thành
t 4 + 8 t 2 + 8 - 4 ( t 2 + 4 ) - 12 t = m ⇔ t 4 + 4 t 2 - 12 t = m + 8 ( * )
Phương trình đã cho có nghiệm trong đoạn [1;2] khi và chỉ khi phương trình (*) có nghiệm trong [-1;1] Xét hàm số y=f(t)= t 4 + 4 t 2 - 12 t trên [-1;1]
Đạo hàm y , = 4 t 8 + 8 t - 12 , t ∈ ( - 1 ; 1 ) . y , = 4 ( t - 1 ) ( t 2 + t + 3 ) < 0 , ∀ t ∈ ( - 1 ; 1 )
Bảng biến thiên:
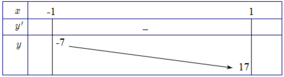
Do đó để phương trình đã cho có nghiệm trên [1;2] thì - 7 ≤ m + 8 ≤ 17 ⇔ - 15 ≤ m ≤ 9

\(\left[{}\begin{matrix}x=\dfrac{7-\sqrt{17}}{8}\\x=\dfrac{7+\sqrt{17}}{8}\end{matrix}\right.\)
\(\Leftrightarrow\left(x-2\right)\left(4x+1\right)=0\)
\(\Leftrightarrow x-2=0\) hay \(4x+1=0\)
\(\Leftrightarrow x=2\) \(\Leftrightarrow\) \(4x=-1\)
\(\Leftrightarrow x=-\dfrac{1}{4}\)
Vậy \(S=\left\{2,-\dfrac{1}{4}\right\}\)
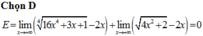
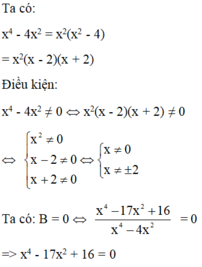



\(=\left(4x^2-7x-50\right)^2-x^2\left(16x^2+56x+49\right)\)
\(=\left(4x^2-7x-50\right)^2-x^2\left(4x+7\right)^2\)
\(=\left(4x^2-7x-50-4x^2-7x\right)\left(4x^2-7x-50+4x^2+7x\right)\)
\(=\left(-14x-50\right)\left(8x^2-50\right)\)
\(=-4\left(7x+25\right)\left(2x-5\right)\left(2x+5\right)\)