Giải pt: \(\left(x+3\sqrt{x}\right)\left(x+9\sqrt{x}+18\right)=168\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
30 tháng 4 2017
\(pt\Leftrightarrow\left(9+4\sqrt{5}\right)^{\dfrac{x}{2}}+\left(9-4\sqrt{5}\right)^{\dfrac{x}{2}}=18\)
Thấy rằng \(9-4\sqrt{5}\) là nghịch đảo của \(9+4\sqrt{5}\)
Do vậy \(\left(9+4\sqrt{5}\right)^{\dfrac{x}{2}}\left(9-4\sqrt{5}\right)^{\dfrac{x}{2}}=1\)
Đặt \(\left(9-4\sqrt{5}\right)^{\dfrac{x}{2}}=t\) ta có pt:
\(t+\dfrac{1}{t}=18\Rightarrow t^2-18t+1=0\Rightarrow t=9\pm4\sqrt{5}\)
Vì vậy \(t=9\pm4\sqrt{5}=\left(9-4\sqrt{5}\right)^{\pm1}=\left(9-4\sqrt{5}\right)^{\dfrac{x}{2}}\)
\(\Rightarrow\dfrac{x}{2}=\pm1\Rightarrow x=\pm2\)
30 tháng 4 2017
\(\sqrt{\left(9+4\sqrt{5}\right)^x}+\sqrt{\left(9-4\sqrt{5}\right)^x}=18\)
<=>\(\sqrt{\left(5+2.2\sqrt{5}+4\right)^x}+\sqrt{\left(5-2.2.\sqrt{5}+4\right)^x}=18\)
<=>\(\sqrt{\left(\sqrt{5}+2\right)^{2x}}+\sqrt{\left(\sqrt{5}-2\right)^{2x}}=18\)
<=>\(\left(\sqrt{5}+2\right)^x+\left(\sqrt{5}-2\right)^x=18\)
Nhận xét:
x>2 thì VT>18=VP
x<2 thì VT<18=VP
x=2 thì VT=VP
Vậy S={2}
27 tháng 11 2021
\(a,ĐK:1\le x\le3\)
Đặt \(\left\{{}\begin{matrix}\sqrt{x-1}=a\\\sqrt{3-x}=b\end{matrix}\right.\left(a,b\ge0\right)\)
\(PT\Leftrightarrow a+b-ab=1\Leftrightarrow a+b-ab-1=0\\ \Leftrightarrow\left(a-1\right)\left(1-b\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}a=1\\b=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x-1=1\\3-x=1\end{matrix}\right.\Leftrightarrow x=2\left(tm\right)\)
\(b,ĐK:0\le x\le9\\ PT\Leftrightarrow9+2\sqrt{x\left(9-x\right)}=-x^2+9x+9\\ \Leftrightarrow2\sqrt{-x^2+9x}-\left(-x^2+9x\right)=0\\ \Leftrightarrow\sqrt{-x^2+9x}\left(2-\sqrt{-x^2+9x}\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}-x^2+9x=0\\\sqrt{-x^2+9x}=2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=9\\x^2-9x+4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\left(n\right)\\x=9\left(n\right)\\x=\dfrac{9+\sqrt{65}}{2}\left(n\right)\\x=\dfrac{9-\sqrt{65}}{2}\left(n\right)\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
26 tháng 2 2021
\(\left\{{}\begin{matrix}xy\left(x+y\right)=2\\\left(x+y\right)^3-3xy\left(x+y\right)+\left(xy\right)^3+7\left(xy+x+y+1\right)=31\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}xy\left(x+y\right)=2\\\left(x+y\right)^3+\left(xy\right)^3+7\left(xy+x+y\right)=30\end{matrix}\right.\)
Đặt \(\left\{{}\begin{matrix}x+y=u\\xy=v\end{matrix}\right.\) với \(u^2\ge4v\)
\(\Rightarrow\left\{{}\begin{matrix}uv=2\\u^3+v^3+7\left(u+v\right)=30\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}uv=2\\\left(u+v\right)^3-3uv\left(u+v\right)+7\left(u+v\right)=30\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}uv=2\\\left(u+v\right)^3+\left(u+v\right)-30=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}uv=2\\u+v=3\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}u=2\\v=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+y=2\\xy=1\end{matrix}\right.\) \(\Leftrightarrow\left(x;y\right)=\left(1;1\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
26 tháng 2 2021
2.
ĐKXĐ: \(0\le x\le\dfrac{3}{2}\)
\(\Leftrightarrow9x\left(3-2x\right)+81+54\sqrt{x\left(3-2x\right)}=49x+25\left(3-2x\right)+70\sqrt{x\left(3-2x\right)}\)
\(\Leftrightarrow9x^2-14x-3+8\sqrt{x\left(3-2x\right)}=0\)
\(\Leftrightarrow9\left(x^2-2x+1\right)-4\left(3-x-2\sqrt{x\left(3-2x\right)}\right)=0\)
\(\Leftrightarrow9\left(x-1\right)^2-\dfrac{36\left(x-1\right)^2}{3-x+2\sqrt{x\left(3-2x\right)}}=0\)
\(\Leftrightarrow9\left(x-1\right)^2\left(1-\dfrac{4}{3-x+2\sqrt{x\left(3-2x\right)}}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\3-x+2\sqrt{x\left(3-2x\right)}=4\left(1\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow2\sqrt{x\left(3-2x\right)}=x+1\)
\(\Leftrightarrow4x\left(3-2x\right)=x^2+2x+1\)
\(\Leftrightarrow9x^2-10x+1=0\Rightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{1}{9}\end{matrix}\right.\)
24 tháng 9 2018
Xửa đề:
\(\left(x+1\right)\left(x+4\right)+3\left(x+4\right)\sqrt{\frac{x+1}{x+4}}-18=0\)
Xet \(x+4>0\)
\(\Rightarrow\left(x+1\right)\left(x+4\right)+3\sqrt{\left(x+1\right)\left(x+4\right)}-18=0\)
Đặt \(\sqrt{\left(x+1\right)\left(x+3\right)}=a\)
\(\Rightarrow a^2+3a-18=0\)
Trường hợp \(x+4< 0\)
Làm tương tự
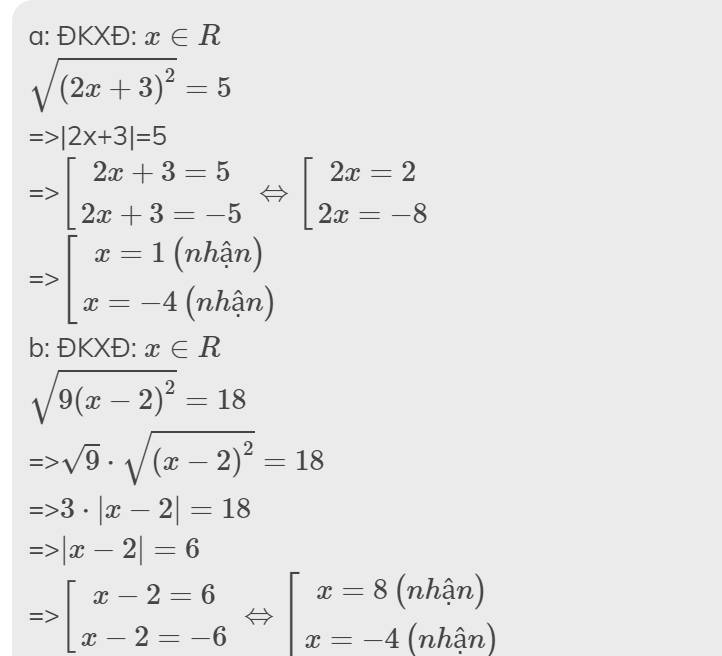
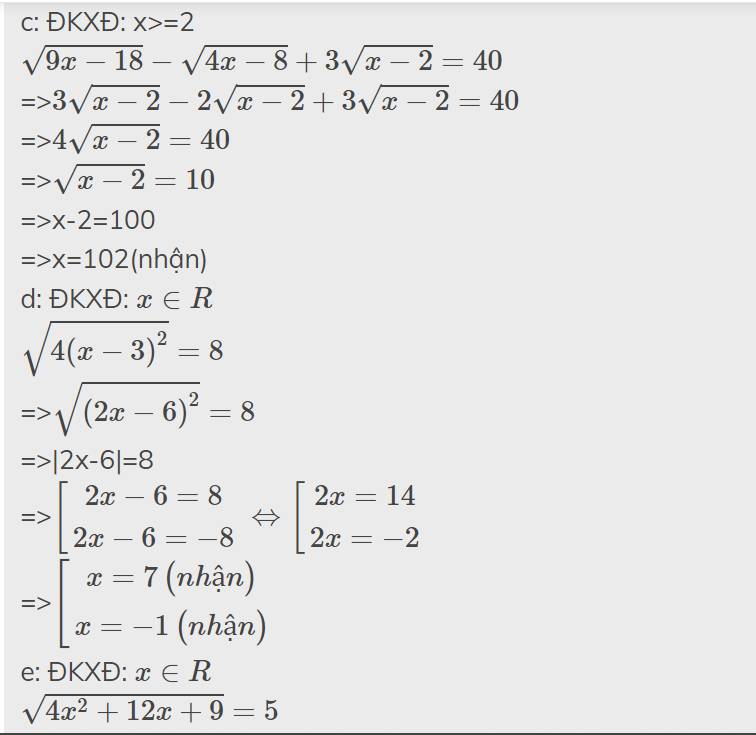
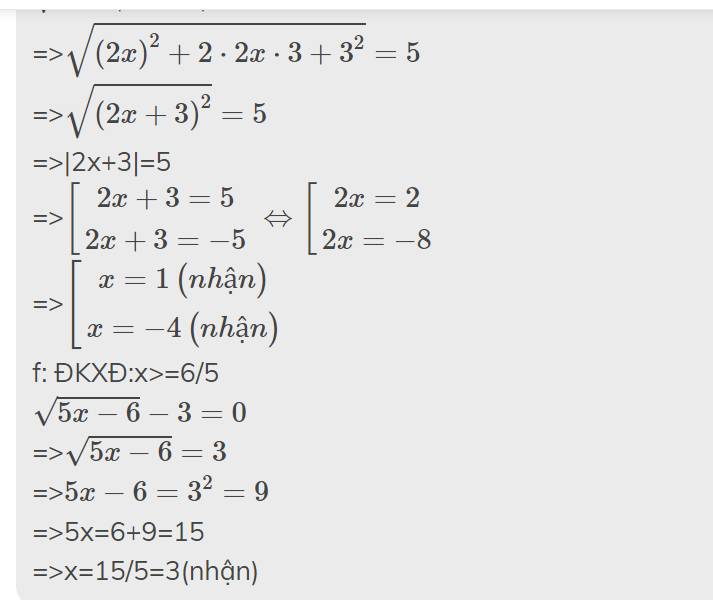
ĐKXĐ: x\(\ge0\)
Ta có:
\(\left(x+3\sqrt{x}\right)\left(x+9\sqrt{x}+18\right)=168\)
\(\Leftrightarrow\sqrt{x}\left(\sqrt{x}+3\right)\left(\sqrt{x+3}\right)\left(\sqrt{x}+6\right)=168\)
\(\Leftrightarrow\left(x+6\sqrt{x}\right)\left(x+6\sqrt{x}+9\right)=168\)
Đặt \(x+6\sqrt{x}=a\)\(\left(a\ge o\right)\). Khi đó:
\(a\left(a+9\right)=168\Leftrightarrow a^2+9a-168=0\)
Bn tu giải tiếp nhé