Tính:
a) (x-1)^3-(x+1)^3+6.((x+1).(x-1)
b) (x-1)^3-(x-1).(x^2+x+1)-3.(1-x).x
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 2:
a) \(=x^2-36y^2\)
b) \(=x^3-8\)
Bài 3:
a) \(=x^2+2x+1-x^2+2x-1-3x^2+3=-3x^2+4x+3\)
b) \(=6\left(x-1\right)\left(x+1\right)=6x^2-6\)

a)

Vậy \(({x^3} + 1):({x^2} - x + 1) = x + 1\).
b)
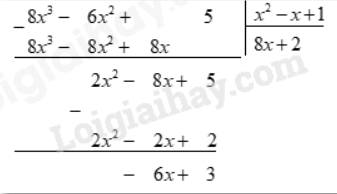
Vậy \((8{x^3} - 6{x^2} + 5) = ({x^2} - x + 1)(8x + 2) + ( - 6x + 3)\)

a) \(({x^2} + 2x + 3) + (3{x^2} - 5x + 1) = ({x^2} + 3{x^2}) + (2x - 5x) + (3 + 1) = 4{x^2} - 3x + 4\);
b) \(\begin{array}{l}(4{x^3} - 2{x^2} - 6) - ({x^3} - 7{x^2} + x - 5) = 4{x^3} - 2{x^2} - 6 - {x^3} + 7{x^2} - x + 5\\ = (4{x^3} - {x^3}) + ( - 2{x^2} + 7{x^2}) - x + ( - 6 + 5) = 3{x^3} + 5{x^2} - x - 1\end{array}\);
c) \(\begin{array}{l} - 3{x^2}(6{x^2} - 8x + 1) = - 3{x^2}.6{x^2} - - 3{x^2}.8x + - 3{x^2}.1\\ = - 18{x^{2 + 2}} + 24{x^{2 + 1}} - 3{x^2} = - 18{x^4} + 24{x^3} - 3{x^2}\end{array}\);
d) \(\begin{array}{l}(4{x^2} + 2x + 1)(2x - 1) = (4{x^2} + 2x + 1).2x - (4{x^2} + 2x + 1).1 = 4{x^2}.2x + 2x.2x + 1.2x - 4{x^2} - 2x - 1\\ = 8{x^{2 + 1}} + 4{x^{1 + 1}} + 2x - 4{x^2} - 2x - 1 = 8{x^3} + 4{x^2} + 2x - 4{x^2} - 2x - 1 = 8{x^3} - 1\end{array}\);
e) \(\begin{array}{l}({x^6} - 2{x^4} + {x^2}):( - 2{x^2}) = {x^6}:( - 2{x^2}) - 2{x^4}:( - 2{x^2}) + {x^2}:( - 2{x^2})\\ = - \dfrac{1}{2}{x^{6 - 2}} + {x^{4 - 2}} - \dfrac{1}{2}{x^{2 - 2}} = - \dfrac{1}{2}{x^4} + {x^2} - \dfrac{1}{2}.\end{array}\);
g)
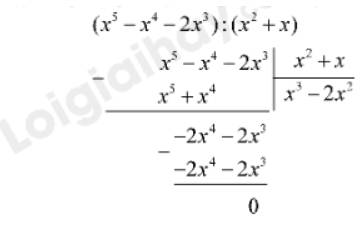
\(({x^5} - {x^4} - 2{x^3}):({x^2} + x)=x^3-2x^2\)

\(\dfrac{2x+4}{x^3-1}-\dfrac{2}{x-1}+\dfrac{x+2}{x^2+x+1}\\ =\dfrac{2x+4}{\left(x-1\right)\left(x^2+x+1\right)}-\dfrac{2}{x-1}+\dfrac{x+2}{x^2+x+1}\\ =\dfrac{2x+4}{\left(x-1\right)\left(x^2+x+1\right)}-\dfrac{2\left(x^2+x+1\right)}{\left(x-1\right)\left(x^2+x+1\right)}+\dfrac{\left(x+2\right)\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}\\ =\dfrac{2x+4-2x^2-2x-2+x^2-x+2x-2}{\left(x-1\right)\left(x^2+x+1\right)}\\ =\dfrac{-x^2+x}{\left(x-1\right)\left(x^2+x+1\right)}\\ =\dfrac{-x\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=-\dfrac{x}{x^2+x+1}\)
`a, 2/(x+1)` hay `2/(x-1)` cậu nhỉ?
`b,`
\(\dfrac{x-1}{x^2-5x+6}-\dfrac{x-3}{x-2}+\dfrac{x-2}{x-3}\\ =\dfrac{x-1}{\left(x-2\right)\left(x-3\right)}-\dfrac{x-3}{x-2}+\dfrac{x-2}{x-3}\\ =\dfrac{x-1}{\left(x-2\right)\left(x-3\right)}-\dfrac{\left(x-3\right)^2}{\left(x-2\right)\left(x-3\right)}+\dfrac{\left(x-2\right)^2}{\left(x-3\right)\left(x-2\right)}\\ =\dfrac{x-1-\left(x^2-6x+9\right)+x^2-4x+4}{\left(x-2\right)\left(x-3\right)}\\ =\dfrac{x-1-x^2+6x-9+x^2-4x+4}{\left(x-2\right)\left(x-3\right)}\\ =\dfrac{3x-6}{\left(x-2\right)\left(x-3\right)}\)
\(=\dfrac{3\left(x-2\right)}{\left(x-2\right)\left(x-3\right)}\\ =\dfrac{3}{x-3}\)

a: \(=\dfrac{x^2-2x+1}{x}:\dfrac{x-1-3x^2+3x-3}{\left(x-1\right)\left(x^2-x+1\right)}\)
\(=\dfrac{\left(x-1\right)^2}{x}\cdot\dfrac{\left(x-1\right)\left(x^2-x+1\right)}{-2x^2+4x-4}\)
\(=\dfrac{\left(x-1\right)^3\cdot\left(x^2-x+1\right)}{-2x\left(x^2-2x+2\right)}\)
b: \(=\left[\dfrac{x^2-2x+1}{x^2+x+1}+\dfrac{2x^2-4x+1}{\left(x-1\right)\left(x^2+x+1\right)}+\dfrac{1}{x-1}\right]:\dfrac{2}{x^2+1}\)
\(=\dfrac{x^3-3x^2+3x+1+2x^2-4x+1+x^2+x+1}{\left(x-1\right)\left(x^2+x+1\right)}\cdot\dfrac{x^2+1}{2}\)
\(=\dfrac{x^3+3}{\left(x-1\right)\left(x^2+x+1\right)}\cdot\dfrac{x^2+1}{2}\)

\(a,=\sqrt{5}-4\sqrt{5}-12\sqrt{5}=-15\sqrt{5}\\ b,=2\sqrt{3}-\dfrac{2+\sqrt{3}}{1}=2\sqrt{3}-2-\sqrt{3}=\sqrt{3}-2\\ c,=\dfrac{x+2\sqrt{x}+1+x-2\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\cdot\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{2\sqrt{x}}\\ =\dfrac{2\left(x+1\right)}{2\sqrt{x}}=\dfrac{x+1}{\sqrt{x}}\)

b: \(=\dfrac{7x-42-x^2+36}{x\left(x-6\right)}=\dfrac{-x^2+7x-6}{x\left(x-6\right)}=\dfrac{-x+1}{x}\)
\(\dfrac{x+3}{\left(x-3\right)\left(x+3\right)}-\dfrac{3}{x\left(x-3\right)}=\dfrac{x\left(x+3\right)-3\left(x+3\right)}{x\left(x-3\right)\left(x+3\right)}=\dfrac{x^2+3x-3x-9}{x\left(x-3\right)\left(x+3\right)}=\dfrac{\left(x-3\right)\left(x+3\right)}{x\left(x-3\right)\left(x+3\right)}=\dfrac{1}{x}\)

Bài 5:
a. 1 - 2y + y2
= (1 - y)2
b. (x + 1)2 - 25
= (x + 1)2 - 52
= (x + 1 - 5)(x + 1 + 5)
= (x - 4)(x + 6)
c. 1 - 4x2
= 12 - (2x)2
= (1 - 2x)(1 + 2x)
d. 8 - 27x3
= 23 - (3x)3
= (2 - 3x)(4 + 6x + 9x2)
e. (đề hơi khó hiểu ''x3'' !?)
g. x3 + 8y3
= (x + 2y)(x2 - 2xy + y2)
a. Câu hỏi của Nguyễn Thị Anh Thư - Toán lớp 8 - Học toán với OnlineMath
a, \(\left(x-1\right)^3-\left(x+1\right)^3+6\left(x+1\right)\left(x-1\right)\)
\(=\left(x-1-x-1\right)\left[\left(x-1\right)^2+\left(x-1\right)\left(x+1\right)+\left(x+1\right)^2\right]+6\left(x^2-1\right)\)
\(=-2\left[x^2-2x+1+x^2-1+x^2+2x+1\right]+6x^2-6\)
\(=-2\left(3x^2+1\right)+6x^2-6=-6x^2-2+6x^2-6=-8\)
b, \(\left(x-1\right)^3-\left(x-1\right)\left(x^2+x+1\right)-3x\left(1-x\right)\)
\(=\left(x-1\right)^3-\left(x-1\right)\left(x^2+x+1\right)+3x\left(x-1\right)\)
\(=\left(x-1\right)\left[\left(x-1\right)^2-\left(x^2+x+1\right)+3x\right]\)
\(=\left(x-1\right)\left(x^2-2x+1-x^2-x-1+3x\right)\)
\(=\left(x-1\right).0=0\)