Biết rằng \(\sin15độ=\frac{\sqrt{6}-\sqrt{2}}{4}\). Tính tỉ số lượng giác của góc 15 độ
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
25 tháng 8 2021
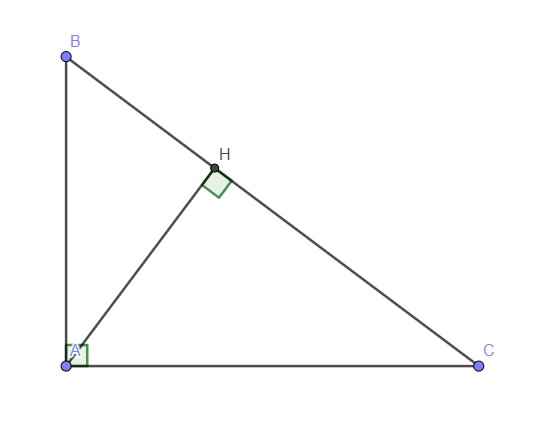
\(tanB=\sqrt{2}\Rightarrow\dfrac{AC}{AB}=\sqrt{2}\Rightarrow\dfrac{AC^2}{AB^2}=2\)
\(\Rightarrow\dfrac{AC^2}{AB^2}+1=3\Rightarrow\dfrac{AC^2+AB^2}{AB^2}=3\Rightarrow\dfrac{BC^2}{AB^2}=3\)
\(\Rightarrow\dfrac{AB}{BC}=\dfrac{1}{\sqrt{3}}\)
Mà \(sinC=\dfrac{AB}{BC}\Rightarrow sinC=\dfrac{1}{\sqrt{3}}\)
\(sin^2C+cos^2C=1\Rightarrow\dfrac{1}{3}+cos^2C=1\Rightarrow cosC=\dfrac{\sqrt{6}}{3}\)
\(tanC=\dfrac{sinC}{cosC}=\dfrac{\sqrt{2}}{2}\)
b.
Trong tam giác vuông ACH:
\(sinC=\dfrac{AH}{AC}\Rightarrow AC=\dfrac{AH}{sinC}=\dfrac{2\sqrt{3}}{\dfrac{1}{\sqrt{3}}}=6\left(cm\right)\)
Trong tam giác vuông ABC:
\(tanB=\dfrac{AC}{AB}\Rightarrow AB=\dfrac{AC}{tanB}=\dfrac{6}{\sqrt{2}}=3\sqrt{2}\)
Áp dụng Pitago:
\(BC=\sqrt{AB^2+AC^2}=3\sqrt{6}\left(cm\right)\)
NH
15 tháng 7 2023
có `cos α=1/2`
`=>cos^2 α=1/4`
Mà `cos^2 α +sin^2 α=1`
`=>1/4+sin^2 α=1`
`=>sin^2 α=1-1/4=3/4`
\(=>sin\alpha=\dfrac{\sqrt{3}}{2}\) (vì `sin α` >0)
ta có `sin α : cos α=tan α`
\(=>tan\alpha=\dfrac{\sqrt{3}}{2}:\dfrac{1}{2}=\sqrt{3}\)
ta có `tan α * cot α =1`
\(=>\sqrt{3}\cdot cot\alpha=1\\ =>cot\alpha=\dfrac{1}{\sqrt{3}}\)
tương tự ta có
\(\left\{{}\begin{matrix}sin\beta=\dfrac{\sqrt{2}}{2}\\cos\beta=1\\cot\beta=1\end{matrix}\right.\)
26 tháng 7 2023
sin a=12/13
cos^2a=1-(12/13)^2=25/169
=>cosa=5/13
tan a=12/13:5/13=12/5
cot a=1:12/5=5/12
sin b=căn 3/2
cos^2b=1-(căn 3/2)^2=1/4
=>cos b=1/2
tan b=căn 3/2:1/2=căn 3
cot b=1/căn 3
HQ
Hà Quang Minh
Giáo viên
21 tháng 9 2023
a) Ta có \({\cos ^2}\alpha + {\sin ^2}\alpha \,\,\, = \,1\)
mà \(\sin \alpha = \frac{{\sqrt {15} }}{4}\) nên \({\cos ^2}\alpha + {\left( {\frac{{\sqrt {15} }}{4}} \right)^2}\,\,\, = \,1 \Rightarrow {\cos ^2}\alpha = \frac{1}{{16}}\)
Lại có \(\frac{\pi }{2} < \alpha < \pi \) nên \(\cos \alpha < 0 \Rightarrow \cos \alpha = - \frac{1}{4}\)
Khi đó \(\tan \alpha = \frac{{\sin \alpha }}{{co{\mathop{\rm s}\nolimits} \alpha }} = - \sqrt {15} ;\cot \alpha = \frac{1}{{\tan \alpha }} = - \frac{1}{{\sqrt {15} }}\)
b)
Ta có \({\cos ^2}\alpha + {\sin ^2}\alpha \,\,\, = \,1\)
mà \(\cos \alpha = - \frac{2}{3}\) nên \({\sin ^2}\alpha + {\left( {\frac{{ - 2}}{3}} \right)^2}\,\,\, = \,1 \Rightarrow {\sin ^2}\alpha = \frac{5}{9}\)
Lại có \( - \pi < \alpha < 0\) nên \(\sin \alpha < 0 \Rightarrow \sin \alpha = - \frac{{\sqrt 5 }}{3}\)
Khi đó \(\tan \alpha = \frac{{\sin \alpha }}{{co{\mathop{\rm s}\nolimits} \alpha }} = \frac{{\sqrt 5 }}{2};\cot \alpha = \frac{1}{{\tan \alpha }} = \frac{2}{{\sqrt 5 }}\)
c)
Ta có \(\tan \alpha = 3\) nên
\(\cot \alpha = \frac{1}{{\tan \alpha }} = \frac{1}{3}\)
\(\frac{1}{{{{\cos }^2}\alpha }} = 1 + {\tan ^2}\alpha \,\,\, = \,1 + {3^2} = 10\,\, \Rightarrow {\cos ^2}\alpha = \frac{1}{{10}}\)
Mà \({\cos ^2}\alpha + {\sin ^2}\alpha \,\,\, = \,1 \Rightarrow {\sin ^2}\alpha = \frac{9}{{10}}\)
Với \( - \pi < \alpha < 0\) thì \(\sin \alpha < 0 \Rightarrow \sin \alpha = - \sqrt {\frac{9}{{10}}} \)
Với \( - \pi < \alpha < - \frac{\pi }{2}\) thì \(\cos \alpha < 0 \Rightarrow \cos \alpha = - \sqrt {\frac{1}{{10}}} \)
và \( - \frac{\pi }{2} \le \alpha < 0\) thì \(\cos \alpha > 0 \Rightarrow \cos \alpha = \sqrt {\frac{1}{{10}}} \)
d)
Ta có \(\cot \alpha = - 2\) nên
\(\tan \alpha = \frac{1}{{\cot \alpha }} = - \frac{1}{2}\)
\(\frac{1}{{{{\sin }^2}\alpha }} = 1 + co{{\mathop{\rm t}\nolimits} ^2}\alpha \,\,\, = \,1 + {( - 2)^2} = 5\,\, \Rightarrow {\sin ^2}\alpha = \frac{1}{5}\)
Mà \({\cos ^2}\alpha + {\sin ^2}\alpha \,\,\, = \,1 \Rightarrow {\cos ^2}\alpha = \frac{4}{5}\)
Với \(0 < \alpha < \pi \) thì \(\sin \alpha > 0 \Rightarrow \sin \alpha = \sqrt {\frac{1}{5}} \)
Với \(0 < \alpha < \frac{\pi }{2}\) thì \(\cos \alpha > 0 \Rightarrow \cos \alpha = \sqrt {\frac{4}{5}} \)
và \(\frac{\pi }{2} \le \alpha < \pi \) thì \(\cos \alpha < 0 \Rightarrow \cos \alpha = - \sqrt {\frac{4}{5}} \)
26 tháng 8 2021
Áp dụng định lí Pytago vào ΔMNP vuông tại M, ta được:
\(MN^2+MP^2=NP^2\)
\(\Leftrightarrow MP^2=3^2-\left(\sqrt{5}\right)^2=4\)
hay MP=2cm
Áp dụng hệ thức lượng trong tam giác vuông vào ΔMNP vuông tại M có MK là đường cao ứng với cạnh huyền NP, ta được:
\(\left\{{}\begin{matrix}MN^2=NK\cdot NP\\MK\cdot NP=MN\cdot MP\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}KN=\dfrac{5}{9}\left(cm\right)\\MK=\dfrac{2\sqrt{5}}{3}\left(cm\right)\end{matrix}\right.\)
Xét ΔNMK vuông tại K có
\(\sin\widehat{NMK}=\dfrac{KN}{MN}=\dfrac{\sqrt{5}}{9}\)
\(\cos\widehat{NMK}=\dfrac{MK}{MN}=\dfrac{2}{3}\)
\(\tan\widehat{NMK}=\dfrac{KN}{KM}=\dfrac{\sqrt{5}}{6}\)
\(\cot\widehat{NMK}=\dfrac{KM}{KN}=\dfrac{6\sqrt{5}}{5}\)
11 tháng 4 2018
Bài 7 :
( bạn đạt A = (...) cái biểu thức đấy nhé, tự đặt )
Ta có :
\(\frac{1}{\sqrt{1}}=\frac{1}{1}>\frac{1}{10}=\frac{1}{\sqrt{100}}\)
\(\frac{1}{\sqrt{2}}>\frac{1}{\sqrt{100}}\)
\(\frac{1}{\sqrt{3}}>\frac{1}{\sqrt{100}}\)
\(............\)
\(\frac{1}{\sqrt{100}}=\frac{1}{\sqrt{100}}\)
\(\Rightarrow\)\(A=\frac{1}{\sqrt{1}}+\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{3}}+...+\frac{1}{\sqrt{100}}>\frac{1}{\sqrt{100}}+\frac{1}{\sqrt{100}}+\frac{1}{\sqrt{100}}+...+\frac{1}{\sqrt{100}}\)
\(A>\frac{100}{\sqrt{100}}=\frac{100}{10}=10\)
\(\Rightarrow\)\(A>10\)
Vậy \(A>10\)
Chúc bạn học tốt ~
BH
11 tháng 4 2018
Bạn làm được mình bài 7 thôi à, mình thấy bạn giỏi lắm mà. Mình có tới mấy chục bài cần giải cơ. Dạo này mình hỏi nhiều vì sắp đi thi.