Mình đg cần gấp
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
giúp mình vs mình đg cần gấp
28 tháng 7 2021
a) Ta có: \(Q=\dfrac{3x+\sqrt{9x}-3}{x+\sqrt{x}-2}-\dfrac{\sqrt{x}+1}{\sqrt{x}+2}+\dfrac{\sqrt{x}-2}{1-\sqrt{x}}\)
\(=\dfrac{3x+3\sqrt{x}-3-\left(x-1\right)-\left(x-4\right)}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{3x+3\sqrt{x}-3-x+1-x+4}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{x+3\sqrt{x}+2}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{\sqrt{x}+1}{\sqrt{x}-1}\)
b) Thay \(x=4+2\sqrt{3}\) vào Q, ta được:
\(Q=\dfrac{\sqrt{3}+1+1}{\sqrt{3}+1-1}=\dfrac{2+\sqrt{3}}{\sqrt{3}}=\dfrac{2\sqrt{3}+3}{3}\)
c) Để Q=3 thì \(\sqrt{x}+1=3\sqrt{x}-3\)
\(\Leftrightarrow\sqrt{x}-3\sqrt{x}=-3-1\)
\(\Leftrightarrow2\sqrt{x}=4\)
hay x=4
28 tháng 7 2021
d) Để \(Q>\dfrac{1}{2}\) thì \(\dfrac{\sqrt{x}+1}{\sqrt{x}-1}-\dfrac{1}{2}>0\)
\(\Leftrightarrow\dfrac{2\sqrt{x}+2-\sqrt{x}+1}{2\left(\sqrt{x}-1\right)}>0\)
\(\Leftrightarrow\sqrt{x}-1>0\)
\(\Leftrightarrow x>1\)
Kết hợp ĐKXĐ, ta được: x>1
e) Để Q nguyên thì \(\sqrt{x}+1⋮\sqrt{x}-1\)
\(\Leftrightarrow2⋮\sqrt{x}-1\)
\(\Leftrightarrow\sqrt{x}-1\in\left\{-1;1;2\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{0;2;3\right\}\)
hay \(x\in\left\{0;4;9\right\}\)
30 tháng 7 2021
Cơ chế hình thành cây có kiểu gen Aaa là do rồi loạn giảm phân, diễn ra ở kì sau của giảm phân 1.
Sơ đồ lai:
P: Aa x Aa
GP: Aa ; 0 ; A ; a
F1: Aaa ; a
TP
26 tháng 1 2022
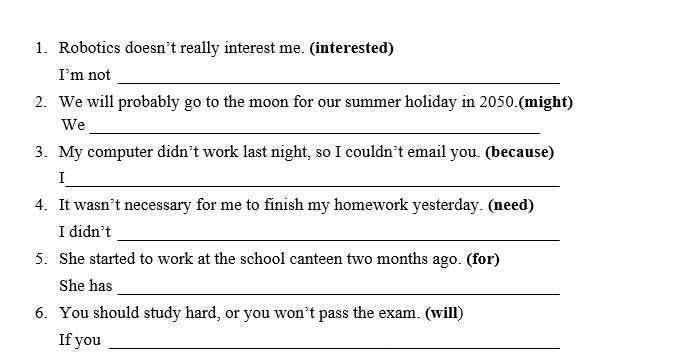
1 I'm not really interested in robotics.
2 We might go to the moon for our summer holiday in 2050.
3 I couldn't email you last night because my computer didn't work.
4 I didn't need to finish my homework yesterday.
5 She has worked at the school canteen for two months.
6 If you don't study hard, you won't pass the exam.
8 tháng 10 2023
\(\left(x^2-1\right)'=2x;\left[\left(x-2\right)^4\right]'=4\cdot\left(x-2\right)^3\cdot\left(x-2\right)'=4\left(x-2\right)^3\)
\(f''\left(x\right)=\left(x^2-1\right)'\left(x-2\right)^4+\left(x^2-1\right)\left[\left(x-2\right)^4\right]'\)
\(=2x\left(x-2\right)^4+\left(x^2-1\right)\cdot4\left(x-2\right)^3\)
\(=2\left(x-2\right)^3\left[x\left(x-2\right)+2x^2-2\right]\)
\(=2\left(x-2\right)^3\left(3x^2-2x-2\right)\)
Đặt \(f'\left(x\right)=0\)
=>\(\left[{}\begin{matrix}x=1\\x=-1\\x=2\end{matrix}\right.\)
\(f''\left(2\right)=0;f''\left(1\right)=2>0;f''\left(-1\right)=-162< 0\)
=>Chọn B
