tìm giá trị nhỏ nhất của hàm số y=sin^2x-4sinx-5
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
DH
0
28 tháng 10 2023
\(f'\left(x\right)=\left(sin^2x\right)'+4\cdot\left(sinx'\right)-5'\)
\(=2\cdot sinx\cdot cosx+4\cdot cosx=2cosx\left(sinx+2\right)\)
\(f'\left(x\right)=0\)
=>\(cosx\left(sinx+2\right)=0\)
=>\(cosx=0\)
=>\(x=\dfrac{\Omega}{2}+k\Omega\)
mà \(x\in\left[0;\dfrac{\Omega}{2}\right]\)
nên \(x=\dfrac{\Omega}{2}\)
\(f\left(\dfrac{\Omega}{2}\right)=sin^2\left(\dfrac{\Omega}{2}\right)+4\cdot sin\left(\dfrac{\Omega}{2}\right)-5\)
=1+4-5=0
\(f\left(0\right)=sin^20+4\cdot sin0-5=-5\)
=>Chọn D
PT
1
BT
0
PP
1
HP
2 tháng 8 2021
Đặt \(sin^24x=t\left(t\in\left[0;1\right]\right)\)
\(y=1-8sin^22x.cos^22x+2sin^42x\)
\(=1-2sin^24x+2sin^42x\)
\(\Rightarrow y=f\left(t\right)=1-2t+2t^2\)
\(y_{min}=min\left\{f\left(0\right);f\left(1\right);f\left(\dfrac{1}{2}\right)\right\}=\dfrac{1}{2}\)
\(y_{max}=max\left\{f\left(0\right);f\left(1\right);f\left(\dfrac{1}{2}\right)\right\}=1\)
PT
1
CM
7 tháng 6 2018
Ta có : y = sin2x – 4sinx – 5= (sinx- 2)2 - 9
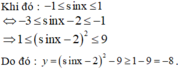
Vậy giá trị nhỏ nhất của hàm số là - 8
Đáp án B
HT
1
NV
Nguyễn Việt Lâm
Giáo viên
19 tháng 9 2021
Đặt \(sinx=t\in\left[-1;1\right]\)
\(y=f\left(t\right)=t^2+2t\)
Xét hàm \(y=f\left(t\right)=t^2+2t\) trên \(\left[-1;1\right]\)
\(-\dfrac{b}{2a}=-1\in\left[-1;1\right]\)
\(f\left(-1\right)=-1\) ; \(f\left(1\right)=3\)
\(\Rightarrow y_{min}=-1\) khi \(sinx=-1\Rightarrow x=-\dfrac{\pi}{2}+k2\pi\)
\(y_{max}=3\) khi \(sinx=1\Rightarrow x=\dfrac{\pi}{2}+k2\pi\)
NC
123
NV
Nguyễn Việt Lâm
Giáo viên
23 tháng 8 2020
\(y=\left(sinx+1\right)\left(sinx-5\right)\)
Do \(-1\le sinx\le1\Rightarrow\left\{{}\begin{matrix}sinx+1\ge0\\sinx-5< 0\end{matrix}\right.\)
\(\Rightarrow y\le0\Rightarrow y_{max}=0\) khi \(sinx=-1\)
\(y=sin^2x-4sinx+3-8=\left(1-sinx\right)\left(3-sinx\right)-8\)
Do \(-1\le sinx\le1\Rightarrow\left\{{}\begin{matrix}1-sinx\ge0\\3-sinx>0\end{matrix}\right.\) \(\Rightarrow\left(1-sinx\right)\left(3-sinx\right)\ge0\)
\(\Rightarrow y_{min}=-8\) khi \(sinx=1\)
HT
16 tháng 9 2020
Cưa cưa em hỏi ké phát, phương pháp chung của những dạng tìm gtnn,ln của hàm số lượng giác là biến đổi nó về dạng gì ạ? Và help me with question, pls:
Max: \(\sin^2x+\cos2x+\sin2x\)