Tìm chữ số x,y biết 10x + y =x3 +y2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
15 tháng 12 2020
a.
\(1-4x^2=\left(1-2x\right)\left(1+2x\right)\)
b.
\(8-27x^3=\left(2\right)^3-\left(3x\right)^3=\left(2-3x\right)\left(4+6x+9x^2\right)\)
c.
\(27+27x+9x^2+x^3=x^3+3.x^2.3+3.3^2.x+3^3\)
\(=\left(x+3\right)^3\)
d.
\(2x^3+4x^2+2x=2x\left(x^2+2x+1\right)=2x\left(x+1\right)^2\)
e.
\(x^2-y^2-5x+5y=\left(x-y\right)\left(x+y\right)-5\left(x-y\right)\)
\(=\left(x-y\right)\left(x+y-5\right)\)
f.
\(x^2-6x+9-y^2=\left(x-3\right)^2-y^2=\left(x-3-y\right)\left(x-3+y\right)\)
8 tháng 12 2023
10: \(x\left(x-y\right)+x^2-y^2\)
\(=x\left(x-y\right)+\left(x-y\right)\left(x+y\right)\)
\(=\left(x-y\right)\left(x+x+y\right)\)
\(=\left(x-y\right)\left(2x+y\right)\)
11: \(x^2-y^2+10x-10y\)
\(=\left(x^2-y^2\right)+\left(10x-10y\right)\)
\(=\left(x-y\right)\left(x+y\right)+10\left(x-y\right)\)
\(=\left(x-y\right)\left(x+y+10\right)\)
12: \(x^2-y^2+20x+20y\)
\(=\left(x^2-y^2\right)+\left(20x+20y\right)\)
\(=\left(x-y\right)\left(x+y\right)+20\left(x+y\right)\)
\(=\left(x+y\right)\left(x-y+20\right)\)
13: \(4x^2-9y^2-4x-6y\)
\(=\left(4x^2-9y^2\right)-\left(4x+6y\right)\)
\(=\left(2x-3y\right)\left(2x+3y\right)-2\left(2x+3y\right)\)
\(=\left(2x+3y\right)\left(2x-3y-2\right)\)
14: \(x^3-y^3+7x^2-7y^2\)
\(=\left(x^3-y^3\right)+\left(7x^2-7y^2\right)\)
\(=\left(x-y\right)\left(x^2+xy+y^2\right)+7\cdot\left(x^2-y^2\right)\)
\(=\left(x-y\right)\left(x^2+xy+y^2\right)+7\left(x-y\right)\left(x+y\right)\)
\(=\left(x-y\right)\left(x^2+xy+y^2+7x+7y\right)\)
15: \(x^3+4x-\left(y^3+4y\right)\)
\(=x^3-y^3+4x-4y\)
\(=\left(x^3-y^3\right)+\left(4x-4y\right)\)
\(=\left(x-y\right)\left(x^2+xy+y^2\right)+4\left(x-y\right)\)
\(=\left(x-y\right)\left(x^2+xy+y^2+4\right)\)
16: \(x^3+y^3+2x+2y\)
\(=\left(x^3+y^3\right)+\left(2x+2y\right)\)
\(=\left(x+y\right)\left(x^2-xy+y^2\right)+2\left(x+y\right)\)
\(=\left(x+y\right)\left(x^2-xy+y^2+2\right)\)
17: \(x^3-y^3-2x^2y+2xy^2\)
\(=\left(x^3-y^3\right)-\left(2x^2y-2xy^2\right)\)
\(=\left(x-y\right)\left(x^2+xy+y^2\right)-2xy\left(x-y\right)\)
\(=\left(x-y\right)\left(x^2+xy+y^2-2xy\right)\)
\(=\left(x-y\right)\left(x^2-xy+y^2\right)\)
18: \(x^3-4x^2+4x-xy^2\)
\(=x\left(x^2-4x+4-y^2\right)\)
\(=x\left[\left(x^2-4x+4\right)-y^2\right]\)
\(=x\left[\left(x-2\right)^2-y^2\right]\)
\(=x\left(x-2-y\right)\left(x-2+y\right)\)
CM
27 tháng 9 2018
Xét x = 0 thì: 10 0 + 48 = y 2 ⇔ y 2 = 49 = 7 2 => y = 7
Xét với x ≠ 0 thì 10 x có chữ số tận cùng là 0, Do đó 10 x + 48 có tận cùng là 8
Mà y 2 là số chính phương nên không thể có tận cùng là 8
Vậy x = 0, y = 7
NH
2
11 tháng 8 2021
a) Ta có: \(M=x^2-2xy+y^2-10x+10y\)
\(=\left(x-y\right)^2-10\left(x-y\right)\)
\(=9^2-10\cdot9=-9\)
3 tháng 10 2023
`#3107.101107`
`D = x^3 - y^3 - 3xy` biết `x - y - 1 = 0`
Ta có:
`x - y - 1 = 0`
`=> x - y = 1`
`D = x^3 - y^3 - 3xy`
`= (x - y)(x^2 + xy + y^2) - 3xy`
`= 1 * (x^2 + xy + y^2) - 3xy`
`= x^2+ xy + y^2 - 3xy`
`= x^2 - 2xy + y^2`
`= x^2 - 2*x*y + y^2`
`= (x - y)^2`
`= 1^2 = 1`
Vậy, với `x - y = 1` thì `D = 1`
________
`E = x^3 + y^3` với `x + y = 5; x^2 + y^2 = 17`
`x + y = 5`
`=> (x + y)^2 = 25`
`=> x^2 + 2xy + y^2 = 25`
`=> 2xy = 25 - (x^2 + y^2)`
`=> 2xy = 25 - 17`
`=> 2xy = 8`
`=> xy = 4`
Ta có:
`E = x^3 + y^3`
`= (x + y)(x^2 - xy + y^2)`
`= 5 * [ (x^2 + y^2) - xy]`
`= 5 * (17 - 4)`
`= 5 * 13`
`= 65`
Vậy, với `x + y = 5; x^2 + y^2 = 17` thì `E = 65`
________
`F = x^3 - y^3` với `x - y = 4; x^2 + y^2 = 26`
Ta có:
`x - y = 4`
`=> (x - y)^2 = 16`
`=> x^2 - 2xy + y^2 = 16`
`=> (x^2 + y^2) - 2xy = 16`
`=> 2xy = (x^2 + y^2) - 16`
`=> 2xy = 26 - 16`
`=> 2xy = 10`
`=> xy = 5`
Ta có:
`F = x^3 - y^3`
`= (x - y)(x^2 + xy + y^2)`
`= 4 * [ (x^2 + y^2) + xy]`
`= 4 * (26 + 5)`
`= 4*31`
`= 124`
Vậy, với `x - y = 4; x^2 + y^2 = 26` thì `F = 124.`
AH
Akai Haruma
Giáo viên
5 tháng 9 2020
Lời giải:
Ta thấy:
$10x\equiv 0\pmod 5$
$288\equiv 3\pmod 5$
$\Rightarrow y^2\equiv 3\pmod 5$ (vô lý)
Do ta biết rằng một số chính phương khi chia cho $5$ chỉ có thể có dư là $0,1,4$.
Như vậy, không tồn tại số tự nhiên $x,y$ thỏa mãn điều kiện đề bài.
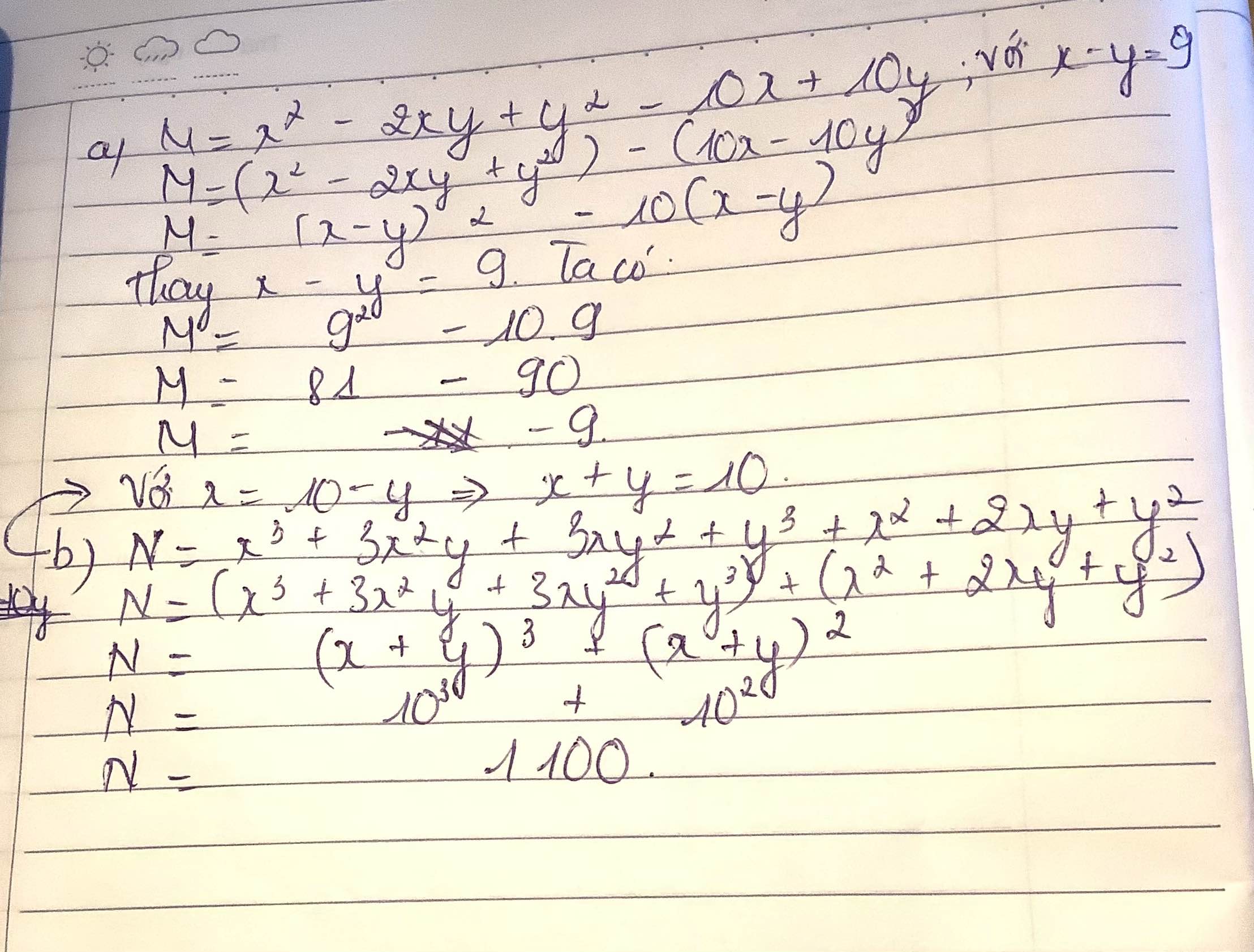
Lời giải:
$x^3+y^2=10x+y<100$
$\Rightarrow x^3<100$
$\Rightarrow x< 4,64\Rightarrow x=1,2,3,4$
Nếu $x=1$ thì:
$10+y=1+y^2\Rightarrow y^2-y=9$
$\Rightarrow y(y-1)=9$ (loại vì không có 2 số nguyên liên tiếp nào có tích bằng 9)
Nếu $x=2$ thì:
$20+y=8+y^2$
$\Rightarrow y^2-y=12$
$\Rightarrow y(y-1)=3.4\Rightarrow y=4$
Nếu $x=3$ thì:
$30+y=27+y^2$
$\Rightarrow y^2-y=3$ (loại vì không có 2 số nguyên liên tiếp nào có tích bằng 3)
Nếu $x=4$ thì:
$40+y=64+y^2$
$\Rightarrow y^2-y=-24<0$ (loại vì với $y\geq 1$ thì $y^2-y\geq 0$)
Vậy.................